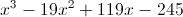Method 1:

The x-intercepts are .  These values would be obtained if the original quadratic were factored, or reverse-FOILed and the factors were set equal to zero.

For , .  For , .  These equations determine the resulting factors and the resulting function; .

Multiplying the factors and simplifying, 

.

Answer: .

Method 2:

Use the form , where is the vertex. 

 is , so , .

Answer:

The zeros for this polynomial are .

This means that the factors are equal to zero when these values are plugged in for x.

multiply both sides by 2

so one factor is

multiply both sides by 3

so one factor is

so one factor is

Multiply these three factors:

The zeros of this polynomial are . This means that the factors equal zero when these values are plugged in.

One factor is

One factor is

The third factor is equivalent to . Set equal to 0 and multiply by 2:

Multiply these three factors:

The graph is negative since it goes down then up then down, so we have to switch all of the signs:

The zeros of the polynomial are . That means that the factors equal zero when these values are plugged in.

The first factor is or equivalently multiply both sides by 5: 

The second and third factors are and

Multiply:

Because the graph goes down-up-down instead of the standard up-down-up, the graph is negative, so change all of the signs:

The zeros for this polynomial are . That means that the factors are equal to zero when these values are plugged in. 

or equivalently multiply both sides by 4

the first factor is 

multiply both sides by 3

the second factor is

the third factor is

Multiply the three factors:

We begin by attempting to find any rational roots using the Rational Root Theorem, which states that the possible rational roots are the positive or negative versions of the possible fractional combinations formed by placing a factor of the constant term in the numerator and a factor of the leading coefficient in the denominator.

That was a lot of wordage in one sentence, so let's break that down.  We begin with our polynomial.

The constant term is the term without a variable (just a plain number).  In our case the constant is 60.  What are the possible factors of 60?

The leading coefficient is the number in front of the largest power of the variable.  When the terms are listed in descending order (highest to lowest power), the leading coefficient is always the first number.  In our case the leading coefficient is hard to spot.  Since there is no number in front of , the coefficient is 1 by default.

This is nice because the only factor of 1 is well ... 1.

We then create all the possible fractions with a factor of the constant in the numerator and a factor of the leading coefficient in the denominator.  This actually isn't as bad as it could be since our only possible denominator is 1.  Any fraction with a denominator of 1 is just the numerator.  Therfore, our possible "fractions" are simply

However, we must consider the positive or negative versions of these, so our final list of possible rational roots is

Unfortunately, this is where the process (at least without the assitance of a graphing calculator) becomes less fun.  Using synthetic division, we must simply try each possible root until we have success. There's really no consistent rule to tell us where to start. Generally starting with the smaller whole numbers is best because the synthetic division is easier.  Therefore, we could begin with  then proceed to , etc.  

For the sake of keeping this explanation as short as possible, I am going to skip straight to 2, where we will first find success.

Therefore, 2 is a root.  However, it is always important to check to see if a root is in fact a double root (it works twice).  Therefore, let's try it one more time.

2 does in fact work twice and is thus a double root.  Since we only have three terms remainng, we can convert from synthetic back to an algebraic expression.

We can then factor.

Writing our root of 2 as an algebraic expression gives .  Since we have double root, we need two of these.  Therfore, our final factored expression is.

Expansion and simplification give us  which is properly expressed in standard form with the constant on the right. Below is a walkthrough of the expansion and simplification (including binomial and trinomial expansion techniques).

Remember to multiply each term in one polynomial with each term in the other polynomial. 

To express this polynomial as a product of linear factors you have to find the zeros of the polynomial by the method of your choosing and then combine the linear expressions that yield those zeros.

Factoring will get you , but then you are left to sort through the thrid degree polynomial. We can quickly synthetically divide the polynomial  by its potential roots  factors of . So that's . 

We know that  is a zero and dividing the original polynomial by  and  gives us the polynomial . We can factor this or use the quadratic formula to give .

This leaves us with four linear expressions that compose the polynomial:

Since 5 is a single root and 7 is a double root, and the degree of the polynomial is 3, the polynomial is . To put this in expanded form:

Use grouping to factorize the common terms:

Therefore, the two real roots come from and .