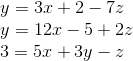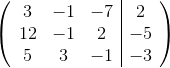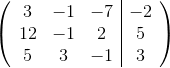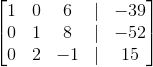Note the first and the last columns are equal.

Therefore, when we try to find the determinant using the following formula we get the determinant equaling 0:

This means simply, that the matrix does not have an inverse.

For a 2x2 matrix

the inverse can be found by 

Because the determinant is equal to zero in this problem, or

,

the inverse does not exist.

We use the inverse of a 2x2 matrix formula to determine the answer. Given a matrix 

 it's inverse is given by the formula:

First we define the determinant of our matrix:

Then, 

This matrix has no inverse because the columns are not linearly independent. This means if you row reduce to try to compute the inverse, one of the rows will have only zeros, which means there is no inverse.

By writing the augmented matrix , and reducing the left side to the identity matrix, we can implement the same operations onto the right side, and we arrive at , with the right side representing the inverse of the original matrix.

There are a couple of ways to do this. I will use the determinant method.

First we need to find the determinant of this matrix, which is

for a matrix in the form:

 .

Substituting in our values we find the determinant to be:

Now one formula for finding the inverse of the matrix is

.

By definition, an inverse matrix is the matrix B that you would need to multiply matrix A by to get the identity. Since the identity matrix yields whatever matrix it is being multiplied by, the answer is the identity itself.

Arrange the equations into the form:

, where a,b,c,d are constants.

Then we have the system of equations: .

The augmented matrix is found by copying the constants into the respective rows and columns of a matrix.

The vertical line in the matrix is analogous to the = sign thus resulting in the following:

Create an augmented matrix by entering the coefficients into one matrix and appending a vector to that matrix with the constants that the equations are equal to. Then you can row reduce to solve the system.

First, lets make this augmented matrix:

Now we can row reduce the matrix using the three row reduction operations: mutliply a row, add one row to another, swap row positions.

First, we can subtract .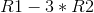 

Swap 

Subtract 

Add  

Subtract 

Mulitply 

You can stop here given that this augmented matrix can be rewritten as a system again with

 , or you can continue using the matrix, subtracting multiples of  from the other two rows to get an identity matrix yielding the solution to the system. 

For two matrices to be equal, two conditions must hold:

1) The matrices must have the same dimension. This can be seen to be the case, since both matrices have two rows and one column.

2) All corresponding entries must be equal. For this to happen, it must hold that

This is a system of two equations in two variables, which can be solved as follows:

Add both sides of the equations:

It follows that

Substitute back:

Thus, there exists a solution to the system, and, consequently, to the original matrix equation. The statement is therefore false.