All Precalculus Resources
Example Questions
Example Question #101 : Exponential And Logarithmic Functions
Express the following in expanded form.
To solve, simply remember that when adding logs, you multiply their insides and when subtract logs, you divide your insides. You must use this in reverse to solve. Thus,
Example Question #67 : Properties Of Logarithms
Completely expand this logarithm:
The answer is not present.
We expand logarithms using the same rules that we use to condense them.
Here we will use the quotient property

and the power property
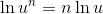
Use the quotient property:
Rewrite the radical:
Now use the power property:
Example Question #102 : Exponential And Logarithmic Functions
Expand the logarithm
In order to expand the logarithmic expression, we use the following properties
As such
Example Question #103 : Exponential And Logarithmic Functions
Given the equation 

The natural logarithm and natural exponent are inverses of each other. Taking the 

That is
Now, 
Example Question #1 : Graph Logarithms
What is the domain of the function
The function 



Example Question #2 : Graph Logarithms
What is the range of the function
To find the range of this particular function we need to first identify the domain. Since 

From here we want to find the function value as 

To find this approximate value we will plug in 
This is our lowest value we will obtain. As we plug in large values we get large function values.
Therefore our range is:
Example Question #1 : Graph Logarithms
Which of the following logarithmic functions match the provided diagram?

Looking at the diagram, we can see that when 



Example Question #1 : Graph Logarithms
Which of the following diagrams represents the graph of the following logarithmic function?






For 









Example Question #2 : Graph Logarithms
Which of the following diagrams matches the given logarithmic function:






For this function, 




Example Question #3 : Graph Logarithms
Which of the following logarithmic functions match the given diagram?

Looking at the graph, the y-value diminishes exponentially as 

Also, 





This indicates that the diagram is consistent with the function 
Certified Tutor
Certified Tutor
All Precalculus Resources














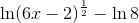

















![[0,\infty ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/350105/gif.latex)

![[-2,\infty ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/350102/gif.latex)
![(0,\infty ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/350104/gif.latex)



![(-\infty,\infty]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/350112/gif.latex)
![[-\infty,\infty]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/350113/gif.latex)





















