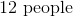All Pre-Algebra Resources
Example Questions
Example Question #1 : Word Problems With One Unknown
John makes $12.50/hour gardening. He earned $275 this week. Write the equation for figuring out his wages, and then solve it to find out how many hours he worked.
Step 1: In order to find out how many hours John works, you first need a formula for calculating his wages:
(hourly wage)(hours)= total wages
Step 2: substitute the known values (hourly wage, total wages)
Step 3: isolate the unknown variable (hours) by dividing both sides by his hourly wage, then solve
Example Question #2 : Algebraic Equations
3 people can pave a driveway in 4 hours. How long will it take for 8 people to pave a driveway?
With inverse proportionality, when one quantity increases the other decreases, and vice versa. The key to solving this problem is to keep in mind that each person works at the same rate regardless of how many people share the workload.
Let:



Using these variables, we can set up an equation that will give us the total time:
Solve for 
Using this constant, we can return to the first equation and solve for the time when 8 people are working:
Example Question #2 : Word Problems With One Unknown
While reading a book Sarah notices that she has read 3 pages after 10 minutes. If she keeps reading at this rate, how many pages will she have read in 1 hour?
The number of pages read is directly proportional to the time spent reading. To solve this problem we need to find the constant of proportionality, which is represented by 
Here 

Using the information given:
One hour is 60 minutes, so using the direct proportionality equation again gives:
Example Question #3 : Word Problems
If it takes 9 hours for 4 people to build a shed, how many people are needed to build the same type of shed in one-third of the time?
The constant of proportionality is equal to the product of time (

Let 


Now, this problem asks for the number of people needed to build the second shed. This means we need to find a way to solve for 

Use the given values to solve. Note that 
Example Question #3 : Word Problems With One Unknown
It takes 45 minutes to drive to the nearest bowling alley taking city streets going 30 miles per hour (mph). How long will it take to get there using the freeway going 65mph if the distance is the same?
(Round to the nearest minute).
We can set up a rate equation, in which distance is equal to rate (speed) times time:
Our speed and time may change with the different routes, but the distance stays the same.
City route:
Freeway route:
We can set these equations equal to each other, since both are equal to 
Solve for the freeway time, 
Rounding to the nearest minute, we get 21 for our final answer.
Example Question #4 : Word Problems With One Unknown
A father is buying cheeseburgers for his children. Each cheeseburger costs $3.50. He spends $17.50 on cheeseburgers. How many cheeseburgers did he buy?
Set up and equation where 
Solve for c:
Example Question #2 : Algebraic Equations
Grace is 4 years older than Elena. If Grace is 9 years old, how old is Elena?
Let Elena's be equal to 
Example Question #8 : Word Problems
Suzie needs to ride her bike to the grocery store, which is is 15 miles away. It takes Suzie 45 minutes to bike to the grocery store. What is Suzies average speed? Express answer in miles per hour.
Distance is the product of rate and time:


Plug in the information given in the problem, remembering that the questions says to state the answer in terms of miles per hour, and we are give her time in minutes. Change minutes to hours:
It took Suzie .75 hours to ride to the grocery store.
Suzie's rate is 
Example Question #4 : Word Problems With One Unknown
An ice cream cone costs 


Calculate the cost of an ice cream cone with tax:
Determine how many cones, 

Divide both sides by 3.18:
Therefore, 

Example Question #5 : Algebraic Equations
Thirty-six friends are renting a party bus to the school prom. Rental of the party bus will cost $300 up front plus $18 per hour. The friends have the bus from 5:00 PM to 3:00 AM.
Each friend has agreed to chip in $12. One of the teachers at the high school, Mr. Jones, has offered to chip in the rest. If he does, how much will he pay?
The friends have the bus from 5:00 PM to 3:00 AM - this is

The cost of renting the bus will be

The thirty-six students will contribute $12 each for a total of

The remaining amount, which Mr. Jones will pay, is

Certified Tutor
Certified Tutor
All Pre-Algebra Resources