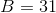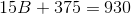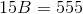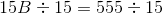All ISEE Upper Level Math Resources
Example Questions
Example Question #41 : Quadrilaterals
A trapezoid has the base lengths of 



The area of a trapezoid is given by

where 


Example Question #1 : How To Find The Area Of A Trapezoid
In the following trapezoid 


The area of a trapezoid is given by

where 


Substitute these values into the area formula:
Example Question #273 : Plane Geometry

What is the area of the shaded portion of the above square?
Quadrilateral 




where 


The length of 


Therefore,

Substituting:
Example Question #42 : Quadrilaterals

Note: Figure NOT drawn to scale.
The area of the above trapezoid is 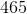

Substitute 
Example Question #43 : Quadrilaterals
Cassie is making a kite for her little brother. She has two plastic tubes to use as the skeleton, measuring 

To find the area of a kite, use the formula 


Since Cassie has one tube measuring 




Example Question #2 : How To Find The Area Of A Kite
Two diagonals of a kite have the lengths of 

The area of a kite is half the product of the diagonals, i.e.

where 

Example Question #3 : How To Find The Area Of A Kite
In the following kite, 



When you know the length of two unequal sides of a kite and their included angle, the following formula can be used to find the area of a kite:

where 


Example Question #4 : How To Find The Area Of A Kite
Find the area of a kite with one diagonal having length 18in and the other diagonal having a length that is half the first diagonal.
To find the area of a kite, we will use the following formula:
where p and q are the lengths of the diagonals of the kite.
Now, we know the length of one diagonal is 18in. We also know the other diagonal is half of the first diagonal. Therefore, the second diagonal has a length of 9in.
Knowing this, we can substitute into the formula. We get
Example Question #1 : Kites
A kite has the area of 

The area of a kite is half the product of the diagonals, i.e.

where 

Example Question #52 : Quadrilaterals

Give the area of the above parallelogram if 
Multiply height 

By the 30-60-90 Theorem:
and
The area is therefore
Certified Tutor
Certified Tutor
All ISEE Upper Level Math Resources