Isosceles triangles always have two equivalent interior angles, and all three interior angles of any triangle always have a sum of  degrees. Since this is an obtuse isosceles triangle, the two missing angles must be acute angles. 

The solution is:

Isosceles triangles always have two equivalent interior angles, and all three interior angles of any triangle always have a sum of  degrees. Since this is an acute isosceles triangle, all of the interior angles must be acute angles.

The solution is:

Isosceles triangles always have two equivalent interior angles, and all three interior angles of any triangle always have a sum of  degrees. Since this is an obtuse isosceles triangle, the two missing angles must be acute angles. 

The solution is:

First, we must draw a picture to include all important parts given in the problem. 

Once this is determined we can use trigonometry to find the angle of elevation. 

Use the inverse sin on a calculator to solve. 

If a triangle is isosceles, two of the angles must be congruent. So the angle must be either 50 degrees or 80 degrees. 

We know that the three angles in all triangles must sum to equal 180 degrees. The only answer choice that is both the same as one of the given angles and results in a sum equal to 180 degrees is the 50 degree angle. 

50+50+80=180. 

An isosceles triangle has 2 congruent angles and then a third angle. These angles, as in any triangle, must add to 180.

One possibility is that the 25-degree angle is the "different" one, and the other two are congruent. This could be expressed using the algebraic expression . To find the other two angles, solve for x. First combine like terms:

subtract 25 from both sides

divide both sides by 2

The other possibility is that there are 2 25-degree angles and then some different angle measure. This could be expressed using the algebraic expression . Again, solve for x. First add the 2 25's to get 50:

subtract 50 from both sides

We are given that, in , two sides are congruent; specifically, . It is a consequence of the Isosceles Triangle Theorem that the angles opposite the sides are also congruent - that is, .

In addition to the fact that  and , we also have that , since, by the Reflexive Property of Congruence, any segment is congruent to itself. We can restate this in a more usable form as ; since we have three side congruences between triangles, it follows from the Side-Side-Side Postulate that .

Neither similarity nor congruence of the two triangles follows from the statements given, as can be seen from the figure below:

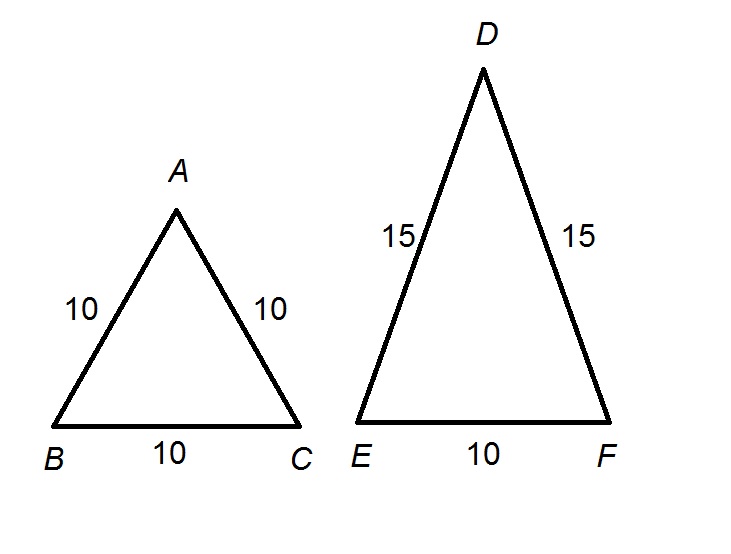
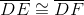
, , and . However, the triangles are not similar, as 

and

, so at least one pair of corresponding sides is not in proportion. Therefore, 

The triangles are not similar, and thus cannot be congruent either, so neither statement holds.

Use the Pythagorean Theorem to find the base of the right triangle.

Now, because two of the angles in this triangle are the same, this is an isosceles triangle. In an isosceles triangle, the sides that are directly across from the congruent angles are also congruent.

In addition, the height in an isosceles triangle will always cut the 3rd side in half. With this information, fill out the triangle as shown below:

To find the perimeter, add up all the sides.