Call Now to Set Up Tutoring:

(888) 888-0446

Intermediate Geometry : Plane Geometry

Study concepts, example questions & explanations for Intermediate Geometry

Create An Account

All Intermediate Geometry Resources

8 Diagnostic Tests 250 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #261 : Triangles

An equilateral triangle is placed on top of a regular hexagon as shown by the figure below.

Find the area of the entire figure.

Possible Answers:

3098.59

2790.18

3199.26

3103.84

Correct answer:

3103.84

Explanation:

Recall that a regular hexagon can be divided into congruent equilateral triangles.

Since the extra equilateral triangle on top has the same side lengths as of the equilateral triangles made by dividing the hexagon, the entire figure has a total of congruent equilateral triangles.

Thus, to find the area of the entire figure, we must first find the area of the equilateral triangle.

Recall how to find the area of an equilateral triangle:

$\text{Area of Equilateral Triangle}=\frac{\sqrt3(\text{side})^2}{4}$

Plug in the length of a side of the equilateral triangle.

$\text{Area of Equilateral Triangle}=\frac{\sqrt3(32)^2}{4}=\frac{1024\sqrt3}{4}=256\sqrt3$

Now, multiply this area by to find the area of the entire figure.

$\text{Area of Figure}=7(\text{Area of Equilateral Triangle})$

$\text{Area of Figure}=7(256\sqrt3)=3103.84$

Make sure to round to places after the decimal.

Report an Error

Example Question #62 : Equilateral Triangles

An equilateral triangle is placed on top of a regular hexagon as shown by the figure below.

Find the area of the entire figure.

Possible Answers:

3612.06

3938.29

4510.20

3782.09

Correct answer:

3938.29

Explanation:

Recall that a regular hexagon can be divided into congruent equilateral triangles.

Since the extra equilateral triangle on top has the same side lengths as of the equilateral triangles made by dividing the hexagon, the entire figure has a total of congruent equilateral triangles.

Thus, to find the area of the entire figure, we must first find the area of the equilateral triangle.

Recall how to find the area of an equilateral triangle:

$\text{Area of Equilateral Triangle}=\frac{\sqrt3(\text{side})^2}{4}$

Plug in the length of a side of the equilateral triangle.

$\text{Area of Equilateral Triangle}=\frac{\sqrt3(36)^2}{4}=\frac{1296\sqrt3}{4}=324\sqrt3$

Now, multiply this area by to find the area of the entire figure.

$\text{Area of Figure}=7(\text{Area of Equilateral Triangle})$

$\text{Area of Figure}=7(324\sqrt3)=3928.29$

Make sure to round to places after the decimal.

Report an Error

Example Question #48 : How To Find The Area Of An Equilateral Triangle

Pyramid

The cube in the above diagram has edges of length 1. Give the area of $\bigtriangleup ABC$ .

Possible Answers:

$\frac{1}{2}$

$\frac{ 3\sqrt{3}}{4}$

$\frac{ \sqrt{3}}{3}$

$\frac{ \sqrt{3}}{2}$

$\frac{2}{3}$

Correct answer:

$\frac{ \sqrt{3}}{2}$

Explanation:

Each of the three triangles $\bigtriangleup ADB$ , $\bigtriangleup ADC$ , and $\bigtriangleup BDC$ has two legs of length 1; therefore, each is an isosceles right triangle, and each is a 45-45-90 triangle. By the 45-45-90 Triangle Theorem, each hypotenuse - $\overline{AB} , \overline{AC} , \overline{BC}$ - has length $\sqrt{2}$ times that of the legs, or simply $\sqrt{2}$ . This makes $\bigtriangleup ABC$ an equilateral triangle with common sidelength $s = \sqrt{2}$ . Using the area formula

$A = \frac{s^{2}\sqrt{3}}{4}$

and substituting, the area can be calculated as

$A = \frac{ (\sqrt{2})^{2}\sqrt{3}}{4} = \frac{2 \sqrt{3}}{4}= \frac{ \sqrt{3}}{2}$ .

Report an Error

Example Question #1 : How To Find The Height Of An Equilateral Triangle

ΔABC is an equilateral triangle with side 12.

Find the height of ΔABC (to the nearest tenth).

Possible Answers:

10.0

10.2

10.6

10.4

9.8

Correct answer:

10.4

Explanation:

Equilateral triangles have sides of all equal length and angles of 60°. To find the height, we can draw an altitude to one of the sides in order to split the triangle into two equal 30-60-90 triangles.

Now, the side of the original equilateral triangle (lets call it "a") is the hypotenuse of the 30-60-90 triangle. Because the 30-60-90 triange is a special triangle, we know that the sides are x, x $\sqrt{3}$ , and 2x, respectively.

Thus, a = 2x and x = a/2.
Height of the equilateral triangle = $\frac{a\sqrt{3}}{2}$

Report an Error

Example Question #1 : How To Find The Height Of An Equilateral Triangle

ΔABC is an equilateral triangle with side of length 8.

Find the height (to the nearest tenth).

Possible Answers:

6.9

7.3

6.7

7.5

7.1

Correct answer:

6.9

Explanation:

Equilateral triangles have sides of equal length, with angles of 60°. To find the height, we can draw an altitude to one of the sides in order to split the triangle into two equal 30-60-90 triangles.

Thus, a = 2x and x = a/2.
Height of the equilateral triangle = $\frac{a\sqrt{3}}{2}$

Report an Error

Example Question #1 : How To Find The Height Of An Equilateral Triangle

What is the height of an equilateral triangle with a side length of 8 in?

Possible Answers:

$4\sqrt{2}$

$6\sqrt{2}$

$6\sqrt{3}$

$4\sqrt{3}$

Correct answer:

$4\sqrt{3}$

Explanation:

An equilateral triangle has three congruent sides, and is also an equiangular triangle with three congruent angles that each meansure 60 degrees.

To find the height we divide the triangle into two special 30 - 60 - 90 right triangles by drawing a line from one corner to the center of the opposite side. This segment will be the height, and will be opposite from one of the 60 degree angles and adjacent to a 30 degree angle. The special right triangle gives side ratios of , $x\sqrt{3}$ , and . The hypoteneuse, the side opposite the 90 degree angle, is the full length of one side of the triangle and is equal to . Using this information, we can find the lengths of each side fo the special triangle.

$8=2x\rightarrow 4=x\rightarrow 4\sqrt{3}=x\sqrt{3}$

The side with length $x\sqrt{3}$ will be the height (opposite the 60 degree angle). The height is $4\sqrt{3}$ inches.

Report an Error

Example Question #1 : How To Find The Height Of An Equilateral Triangle

What is the height of a triangle with side lengths 4, 4, 4?

Possible Answers:

$\sqrt{12}$

$\sqrt{36}$

$\sqrt{20}$

Correct answer:

$\sqrt{12}$

Explanation:

To solve, it's easiest to first visualize the height's relationship with the rest of the triangle's sides:

Equilateral height

The height is one of the legs of a right triangle. The hypotenuse is 4, and the other leg is 2, or half of the base side, 4. To determine the height, use Pythagorean Theorem:

x^2 + 2^2 = 4^2

x^2 + 4 = 16 subtract 4 from both sides

x^2 = 12 take the square root of both sides

$x = \sqrt{12}$

Report an Error

Example Question #1 : How To Find The Height Of An Equilateral Triangle

If an equilateral triangle has a length of $6\;cm$ for all sides, what would be the heigh of the triangle? Round to the nearest tenth.

Possible Answers:

$4.2\;cm$

$5.2\;cm$

$4.5\;cm$

$3.9\;cm$

$6\;cm$

Correct answer:

$5.2\;cm$

Explanation:

The following formula can be used to determine the height of an equilateral triangle when we are given the length of the sides:

$\\h^2=s^2-\frac{s^2}{2} \\h^2=(6\;cm)^2-(\frac{1}{2}\cdot6\;cm)^2$

$\\h^2=36\;cm^2-9\;cm^2\\h^2=27\;cm^2\\h\approx5.2\;cm$

Report an Error

Example Question #2 : How To Find The Height Of An Equilateral Triangle

The equilateral triangle shown below has a side length of $15\;cm$ . Given this information, solve for the height of the triangle.

Varsity record

Possible Answers:

$15\;cm$

$12\;cm$

$14\;cm$

$13\;cm$

$11\;cm$

Correct answer:

$13\;cm$

Explanation:

To solve for the height of an equilateral triangle, we can divide the triangle into two right triangles. In the below image, the bisecting line represents the height, and we can solve for height by applying the Pythagorean Theorem:

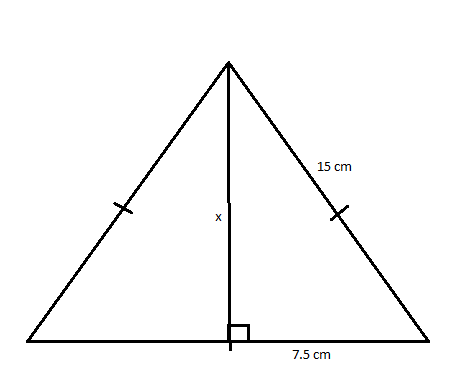
Varsity record

$\\z^2=x^2+y^2\\(15\;cm)^2=x^2+(7.5\;cm)^2\\225\;cm^2=x^2+56.25\;cm^2\\x^2=168.75\;cm^2\\x=\sqrt{168.75\;cm^2}\approx13\;cm$

Report an Error

Example Question #1 : How To Find The Height Of An Equilateral Triangle

Find the height of an equilateral triangle with a side length of $12\:cm$ . Round your answer to the nearest tenth.

Possible Answers:

$10.1\:cm$

$12.0\:cm$

$10.4\:cm$

$10.2\:cm$

$12.0\sqrt2\:cm$

Correct answer:

$10.4\:cm$

Explanation:

An equilateral triangle has three equal sides, as can be deduced from its name. This also means that as a result, the triangle is also equiangular. That is, all its interior angles are the same. Because the sum of internal angles of a triangle is $180^{\circ}$ , that means that each interior angle is $60^{\circ}$ .

Find_the_height_of_equilateral

The height (the dotted line in the diagram) is the length that bisects the apical angle while bisecting the base of the triangle. Drawing the dotted line splits the equilateral triangle into two congruent right triangles. Because they're the same, we only need to consider one of the two smaller triangles to obtain our answer. Keeping in mind that the base of the equilateral triangle has been bisected, this means that the base of the right triangle has the length of $6\:cm$ . With a base of $6\:cm$ and a hypotenuse of $12\:cm$ , we can easily solve for the height (the third side) with the Pythagorean Theorem.

This problem can also be solved using trigonometric functions or even revisiting 30-60-90 triangle rules.

Using the Pythagorean Theorem,

a^2+b^2=c^2

a^2+6^2=12^2

a^2+36=144

a^2+36-36= 144-36

a^2=108

$\sqrt{a^2}=\sqrt{108}$

$a=\sqrt{108}$

$a= 10.39 \approx10.4\:cm$

Therefore, the height of the equilateral triangle is $10.4\:cm$ .

Report an Error

View Tutors

Jayla
Certified Tutor

Rasmussen university, Bachelor of Science, Health Services Administration. Rasmussen university, Master of Science, Business ...

View Tutors

Lilian Natalia
Certified Tutor

University of Texas Rio Grande Valley, Bachelor of Science, Mechanical Engineering. University of Texas Rio Grande Valley, Ma...

View Tutors

Naomi
Certified Tutor

Oakwood University, Bachelor in Arts, Communication, General. Western Governors University, Bachelor in Arts, Elementary Scho...

All Intermediate Geometry Resources

8 Diagnostic Tests 250 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

ISEE Tutors in San Diego, LSAT Tutors in New York City, SSAT Tutors in Chicago, French Tutors in Phoenix, Statistics Tutors in Miami, SSAT Tutors in New York City, ACT Tutors in Los Angeles, Chemistry Tutors in San Francisco-Bay Area, GMAT Tutors in Washington DC, Spanish Tutors in Houston

Popular Courses & Classes

MCAT Courses & Classes in Washington DC, ACT Courses & Classes in Chicago, ACT Courses & Classes in Miami, GMAT Courses & Classes in New York City, GMAT Courses & Classes in Miami, GRE Courses & Classes in Washington DC, GMAT Courses & Classes in Philadelphia, SAT Courses & Classes in Phoenix, SSAT Courses & Classes in Houston, LSAT Courses & Classes in Seattle

Popular Test Prep

SSAT Test Prep in Washington DC, ACT Test Prep in Chicago, ACT Test Prep in Miami, ISEE Test Prep in Houston, SSAT Test Prep in Seattle, MCAT Test Prep in Los Angeles, SAT Test Prep in Washington DC, LSAT Test Prep in Houston, SSAT Test Prep in San Francisco-Bay Area, SSAT Test Prep in Phoenix

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Intermediate Geometry : Plane Geometry

Study concepts, example questions & explanations for Intermediate Geometry

All Intermediate Geometry Resources

Example Questions

Example Question #261 : Triangles

Example Question #62 : Equilateral Triangles

Example Question #48 : How To Find The Area Of An Equilateral Triangle

Example Question #1 : How To Find The Height Of An Equilateral Triangle

Example Question #1 : How To Find The Height Of An Equilateral Triangle

Example Question #1 : How To Find The Height Of An Equilateral Triangle

Example Question #1 : How To Find The Height Of An Equilateral Triangle

Example Question #1 : How To Find The Height Of An Equilateral Triangle

Example Question #2 : How To Find The Height Of An Equilateral Triangle

Example Question #1 : How To Find The Height Of An Equilateral Triangle

All Intermediate Geometry Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: