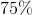Conduction is a form of heat transfer requiring direct contact between two objects. In the question, the burned side of the bread was likely in direct contact with the heat source, while the other was only in contact with hot air. The burned side is heated by conduction, while the uncooked side is heated by convection.

Convection is the transfer of heat through a fluid medium, namely a liquid or gas. Radiation is the transfer of heat through electromagnetic waves.

Conduction is the form of heat transfer that requires direct contact between two objects.

Radiation is heat transfer via electromagnetic radiation. Convection uses a fluid medium, such as air or water, for heat transfer. Induction and thermodynamic discharge are not recognized types of heat transfer.

Conduction occurs when heat is transferred by direct contact between two objects. Convection occurs when heat is transferred via contact between a fluid and an object. Radiation is heat transfer via electromagnetic radiation.

In this question, the fire is a source of heat and electromagnetic radiation. When the radiation from the fire impacts the marshmallow, it is being heated by radiation. The fire is also heating the surrounding air, creating fluid currents. The heated air is also transferring energy to the marshmallow, heating by convection.

There is no conduction in this example.

The efficiency of a Carnot Cycle is defined by:

In this calculation, temperatures are given in Kelvin. Since Celsius and Kelvin exist in a one-to-one ratio, a conversion is unnecessary unless desired. We will perform the conversion, since most classes will advise it.

Using these values, we can calculate the efficiency:

Convert this value to a percentage:

For this problem, use Gay-Lussac's law to set up a direct proportion between pressure and temperature. Note that this law only applies when volume is constant.

Plug in our given values and solve for the initial pressure.

The formula for heat energy is:

We are given the initial and final temperatures, mass, and specific heat. Using these values, we can find the heat released. Note that the time is irrelevant to this calculation.

That means that the soup "lost"  of energy. This is the amount that it released into the room. The value is negative for the soup, the source of the heat, but positive for the room, which receives it.

The formula for heat energy is:

We are given the final temperature, mass, specific heat, and heat released. Using these values, we can find the initial temperature. Note that the time is irrelevant to this calculation. Since heat is released from the soup, the net change in the soup's energy is negative. Since the soup is cooling, we expect our answer to be greater than .

The formula for heat energy is:

We are given the initial temperature, mass, specific heat, and heat released. Using these values, we can find the final temperature. Note that the time is irrelevant to this calculation. Since heat is released from the soup, the net change in the soup's energy is negative. Since the soup is cooling, we expect our answer to be less than .

When an object changes phase, it requires energy called "latent heat." In this case, even though the temperature is remaining constant, the energy inside of the ice cube is decreasing as it expends energy to melt.

The equation for two items reaching a thermal equilibrium is given by describing a heat transfer. The heat removed from one object is equal to the heat added to the other.

We are given the specific heat values of each substance, as well as their masses. We also know the initial temperature of each substance. Use these terms in the equation to solve for the final temperature. Remember that the final temperature will be the same for each substance, since they will be in thermodynamic equilibrium.