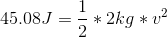Call Now to Set Up Tutoring:

(888) 888-0446

High School Physics : Calculating Kinetic Energy

Study concepts, example questions & explanations for High School Physics

Create An Account

All High School Physics Resources

6 Diagnostic Tests 233 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #31 : Energy And Work

A 2kg book falls off the top of a 2.3m bookshelf. What is its velocity right before it hits the ground?

$\small g=-9.8\frac{m}{s^2}$

Possible Answers:

$-26.86\frac{m}{s}$

$-6.71\frac{m}{s}$

$13.43\frac{m}{s}$

$45.08\frac{m}{s}$

$3.36\frac{m}{s}$

Correct answer:

$-6.71\frac{m}{s}$

Explanation:

The relationship between velocity and energy is:

$KE=\frac{1}{2}mv^2$

We know the mass, but we need to find the total kinetic energy.

Remember the law of conservation of energy: the total energy at the beginning equals the total energy at the end. In this case, we have only potential energy at the beginning and only kinetic energy at the end. (The initial velocity is zero, and the final height is zero).

PE_i=KE_f

If we can find the potential energy, we can find the kinetic energy. The formula for potential energy is 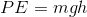PE=mgh .

Using our given values for the mass, height, and gravity, we can solve using multiplication. Note that the height becomes negative because the book is traveling in the downward direction.

$PE=2kg*-9.8\frac{m}{s^2}*-2.3m$

PE=45.08J

The kinetic energy will also equal 45.08J , due to conservation of energy.

Using this value and our given mass, we can calculate the velocity from our original kinetic energy equation.

$KE=\frac{1}{2}mv^2$

$45.08J=\frac{1}{2}*2kg*v^2$

45.08J=1kg*v^2

$45.08\frac{m^2}{s^2}=v^2$

$\sqrt{45.08\frac{m^2}{s^2}}=\sqrt{v^2}$

Since we are taking the square root, our answer can be either negative or positive. The final velocity of the book will be in the downward direction; thus, our final velocity should be negative.

$-6.71\frac{m}{s}=v$

Report an Error

Example Question #31 : Energy

Starting from rest a ball with a mass of 3kg experiences a constant force of 9N for 4s. Find the final kinetic energy (in joules) of the ball after 4s.

Possible Answers:

286J

216J

200J

150J

320J

Correct answer:

216J

Explanation:

Use Newton's laws to find the acceleration of the ball. Find the final speed of the ball, and then solve for kinetic energy.

Acceleration:

F=ma

$9N=(3kg)a\rightarrow a=\frac{9N}{3kg}=3\frac{m}{s^2}$

Final speed:

$V=V_{0}+at$

$V=0+\left(3\frac{m}{s^2}\right)4s=12\frac{m}{s}$

Kinetic energy:

$KE=\frac{1}{2}mv^2$

$KE=\frac{1}{2}(3kg)\left(12\frac{m}{s}^2\right)=216J$

Report an Error

Example Question #32 : Energy And Work

Sam throws a 0.5kg rock off the edge of a 10m tall building at an angle of $35^{\circ}$ from the horizontal. The rock has an initial speed of $30\frac{m}{s}$ .

$\small g=-9.8\frac{m}{s^2}$

What is the final vertical kinetic energy right before the rock hits the ground?

Possible Answers:

49J

123.1J

166J

88.4J

98J

Correct answer:

123.1J

Explanation:

The formula for kinetic energy is $KE=\frac{1}2mv^2{}$ .

We first need to find the initial velocity in the vertical direction. To find the vertical velocity we use the equation $v_{i}* \sin(\Theta)=v_{iy}$ .

We can plug in the given values for the angle and initial velocity to solve.

$30\frac{m}{s}* \sin(35^{\circ})=v_{iy}$

$30\frac{m}{s}* (0.57)=v_{iy}$

$17.21\frac{m}{s}=v_{iy}$

We know that the rock is going to travel a net distance of -10m , as that is the distance between where the rock's initial and final positions. We now know the displacement, initial velocity, and acceleration, which will allow us to solve for the final velocity.

$v_f^2=v_1^2+2a\Delta y$

$v_f^2=(17.21\frac{m}{s})^2+2(-9.8\frac{m}{s^2})(-10m)$

$v_f^2=(296.18\frac{m^2}{s^2})+196\frac{m^2}{s^2}$

$v_f^2=492.18\frac{m^2}{s^2}$

$\sqrt{v_f^2}=\sqrt{492.18\frac{m^2}{s^2}}$

$v_{fy}=22.19\frac{m}{s}$

Now we have the mass and final velocity, allowing us to solve for the final kinetic energy.

$KE_f=\frac{1}{2}mv_f^2$

$KE_f=\frac{1}{2}(0.5kg)(22.19\frac{m}{s})^2$

KE_f=123.1J

Report an Error

View Tutors

Donald
Certified Tutor

Cleveland State University, Bachelor of Science, Chemistry. University of Southern California, Doctor of Philosophy, Organic ...

View Tutors

Victoria
Certified Tutor

Norte Dame de Namur University, Bachelor in Arts, Sociology. University of San Francisco, Master of Arts, Organizational Lead...

View Tutors

Shweta
Certified Tutor

All India Management Association , Master's/Graduate, Human Resource Management . Indian Law Institute , Master's/Graduate, C...

All High School Physics Resources

6 Diagnostic Tests 233 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Algebra Tutors in Washington DC, Calculus Tutors in Seattle, SAT Tutors in Los Angeles, GMAT Tutors in Houston, LSAT Tutors in Washington DC, Spanish Tutors in New York City, Calculus Tutors in Chicago, SAT Tutors in Chicago, SAT Tutors in Washington DC, MCAT Tutors in Miami

Popular Courses & Classes

SAT Courses & Classes in San Francisco-Bay Area, Spanish Courses & Classes in New York City, ACT Courses & Classes in Los Angeles, LSAT Courses & Classes in Los Angeles, Spanish Courses & Classes in Boston, GMAT Courses & Classes in New York City, GRE Courses & Classes in Chicago, Spanish Courses & Classes in Miami, LSAT Courses & Classes in Washington DC, ISEE Courses & Classes in Denver

Popular Test Prep

ISEE Test Prep in Boston, ACT Test Prep in Denver, LSAT Test Prep in Washington DC, GMAT Test Prep in Philadelphia, LSAT Test Prep in Denver, ACT Test Prep in Atlanta, SSAT Test Prep in Atlanta, GRE Test Prep in Chicago, GMAT Test Prep in Los Angeles, SSAT Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

High School Physics : Calculating Kinetic Energy

Study concepts, example questions & explanations for High School Physics

All High School Physics Resources

Example Questions

Example Question #31 : Energy And Work

Example Question #31 : Energy

Example Question #32 : Energy And Work

All High School Physics Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: