All High School Math Resources
Example Questions
Example Question #1 : Applying The Law Of Cosines
A triangle has sides of length 12, 17, and 22. Of the measures of the three interior angles, which is the greatest of the three?
We can apply the Law of Cosines to find the measure of this angle, which we will call :
The widest angle will be opposite the side of length 22, so we will set:


Example Question #1 : Applying The Law Of Cosines
In 




A triangle with these characteristics cannot exist.
By the Law of Cosines:
or, equivalently,
Substitute:
Example Question #91 : Trigonometry

In this figure, angle 



For this problem, use the law of sines:

In this case, we have values that we can plug in:
Cross multiply:
Multiply both sides by 
Example Question #92 : Trigonometry

In this figure 



For this problem, use the law of sines:

In this case, we have values that we can plug in:
Example Question #1 : Applying The Law Of Sines
In 




Since we are given 


Substitute in the above equation:
Cross-multiply and solve for 
Example Question #2 : Applying The Law Of Sines
In 




No triangle can exist with these characteristics.
Since we are given 




Substitute and solve for 
Take the inverse sine of 0.6355:
There are two angles between 


This, however, is impossible, since this would result in the sum of the triangle measures being greater than 

Example Question #92 : Trigonometry

In this figure, angle 



Undefined
For this problem, use the law of sines:
%7D)
In this case, we have values that we can plug in:
Example Question #11 : Triangles
 In this figure, if angle
In this figure, if angle 



(NOTE: Figure not necessarily drawn to scale.)
Undefined
First, observe that this figure is clearly not drawn to scale. Now, we can solve using the law of sines:

In this case, we have values that we can plug in:
Example Question #1 : Solving Triangles
There is not enough information
The side-angle-side (SAS) postulate can be used to determine that the triangles are similar. Both triangles share the angle farthest to the right. In the smaller triangle, the upper edge has a length of 



The statement is true, so the triangles must be similar.
We can use this ratio to solve for the missing side length.
To simplify, we will only use the lower edge and left edge comparison.
Cross multiply.
Example Question #11 : Triangles
We can solve using the trigonometric definition of tangent.
We are given the angle and the adjacent side.
We can find 
Certified Tutor
All High School Math Resources














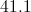







































































 .
.


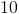






 .
.













