All High School Math Resources
Example Questions
Example Question #5 : Solving And Graphing Radical Equations
Solve the following radical expression:
Begin by taking the square of both sides:
Combine like terms:
Factor the equation and solve:
However, when plugging in the values, 
Example Question #6 : Solving And Graphing Radical Equations
Solve the following radical expression:
To solve the radical expression, begin by subtracting 

Now, square both sides of the equation:

Combine like terms:

Factor the expression and solve:

However, when plugged into the original equation, 
Example Question #41 : Radicals
Solve the equation for 
Add 
Square both sides.
Isolate 
Example Question #92 : Algebra Ii
Solve for 
Begin by cubing both sides:

Now we can easily solve:
Example Question #1 : Exponents
Which of the following is equivalent to 
By definition,

In our problem, 

Then, we have 
Example Question #1 : Negative Exponents
Solve for 
Raise both sides of the equation to the inverse power of 
Subtract 
Example Question #1 : Fractional Exponents
Convert the exponent to radical notation.
Remember that exponents in the denominator refer to the root of the term, while exponents in the numerator can be treated normally.
Example Question #91 : Algebra Ii
Which of the following is equivalent to 
By definition, a number raised to the 
Since the square root of 64 is 8, 8 is our solution.
Example Question #1 : Fractional Exponents
Simplify the expression:
Remember that fraction exponents are the same as radicals.
A shortcut would be to express the terms as exponents and look for opportunities to cancel.
Either method, we then need to multiply to two terms.
Example Question #1 : Multiplying And Dividing Exponents
Simplify the following expression.
When dividing with exponents, the exponent in the denominator is subtracted from the exponent in the numerator. For example: 
In our problem, each term can be treated in this manner. Remember that a negative exponent can be moved to the denominator.
Now, simplifly the numerals.
Certified Tutor
All High School Math Resources



































![\sqrt[3]{y+1}=3](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/151936/gif.latex)





![(\sqrt[3]{y+1}=3)^3](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/151992/gif.latex)

















![\small \small \sqrt[3]{x^7}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/39747/gif.latex)


![\small \small \sqrt[7]{x^3}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/39746/gif.latex)
![x^{\frac{a}{b}}=\sqrt[b]{x^a}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/88792/gif.latex)
![x^{\frac{3}{7}}=\sqrt[7]{x^3}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/110623/gif.latex)









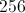

![256^{\frac{3}{4}}=\sqrt[4]{256^3}=64](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/113588/gif.latex)













