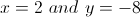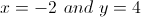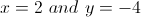We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

High School Math : Basic Single-Variable Algebra

Study concepts, example questions & explanations for High School Math

Create An Account

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

← Previous 1 2 3 4 5 6 Next →

Example Question #31 : Equations

is what percent of ?

Possible Answers:

Correct answer:

Explanation:

Verbal cues include "IS" means equals and "OF" means multiplication.

So the equation to solve becomes

$15=P\cdot 75$ and dividing both sides by gives $P=0.20 \; or \; 20\%$

Report an Error

Example Question #1 : Systems Of Equations

Solve the system of equations.

4x+y=16

2x+3y=18

Possible Answers:

(-3,4)

(4,3)

(3,4)

(-4,3)

None of the other answers are correct.

Correct answer:

(3,4)

Explanation:

Isolate in the first equation.

y=16-4x

Plug into the second equation to solve for .

2x+3(16-4x)=18

2x+48-12x=18

-10x+48=30

-10x=-30

x=3

Plug into the first equation to solve for .

4(3)+y=16

12+y=16

y=4

Now we have both the and values and can express them as a point: (3,4) .

Report an Error

Example Question #1 : Solving Equations

Solve for and .

3x+2y=2

2x+2y=4

Possible Answers:

$x=-2\ and\ y=8$

$x=-2\ and\ y=4$

$x=2\ and\ y=-8$

Cannot be determined.

$x=2\ and\ y=-4$

Correct answer:

$x=-2\ and\ y=4$

Explanation:

1st equation: 3x+2y=2

2nd equation: 2x+2y=4

Subtract the 2nd equation from the 1st equation to eliminate the "2y" from both equations and get an answer for x:

Plug the value of into either equation and solve for :

2(-2)+2y=4

-4+2y=4

2y=4+4

2y=8

Report an Error

Example Question #31 : Equations

Solve for :

$\left | 5x + 39 \right | = 44$

Possible Answers:

$x = -16\frac{3}{5} \textrm{ or } x = 1$

$x = 16\frac{3}{5}$

$x = -1 \textrm{ or } x = 1$

$x = 1 \textrm{ or }x = 16\frac{3}{5}$

$x = -16\frac{3}{5} \textrm{ or }x = 16\frac{3}{5}$

Correct answer:

$x = -16\frac{3}{5} \textrm{ or } x = 1$

Explanation:

Rewrite $\left | 5x + 39 \right | = 44$ as a compound statement and solve each part separately:

$\left | 5x + 39 \right | = 44$

$5x + 39 = - 44 \textrm{ or }5x + 39 = 44$

5x + 39 = - 44

5x + 39 - 39 = - 44- 39

5x = - 83

$5x \div 5 = - 83 \div 5$

$x = -16\frac{3}{5}$

5x + 39 = 44

5x + 39 - 39 = 44- 39

5x = 5

$5x \div 5 =5 \div 5$

x = 1

Therefore the solution set is $\left \{-16\frac{3}{5}, 1 \right \}$ .

Report an Error

Example Question #33 : Equations

Solve the following equation for :

3(x+2) = 4(x-3)

Possible Answers:

x = 2

x = 18

x = -18

x = -9

x = -6

Correct answer:

x = 18

Explanation:

3(x+2) = 4(x-3)

The first step in solving this equation is to distribute the 3 and the 4 through the parentheses:

$3\cdot x+3\cdot2 = 4\cdot x-4\cdot 3$

Simplify:

3x+6 = 4x-12

Now, we want to get like terms on the same sides of the equation. That is, all of the terms with an should be on one side, and those without an should be on the other. To do this, we first subtract from both sides:

3x+6 -4x= 4x-12-4x

Simplify:

-x+6 = -12

Now, we subtract 6 from both sides:

-x+6-6 = -12-6

-x = -18

x = 18

Report an Error

Example Question #34 : Equations

Goldenrod paint is made by mixing one part red with three parts yellow. How many gallons of yellow paint should be mixed with two quarts of red paint?

Possible Answers:

$0.75\; gallons$

$1.50\; gallons$

$1.00\; gallon$

$1.25\; gallons$

$1.75\; gallons$

Correct answer:

$1.50\; gallons$

Explanation:

This problem is solved using proportions and the following conversion factor:

$4\; quarts=1\; gallon$

Let yellow quarts of paint.

$\frac{red}{yellow}=\frac{1}{3}=\frac{2\; quarts}{x\; quarts}$

Then cross multiply to get $x=6\; quarts = 1.50 \; gallons$ .

Report an Error

Example Question #35 : Equations

Solve for :

$\frac{1}{2}(x+3)=\frac{1}{3}(x+5)$

Possible Answers:

Correct answer:

Explanation:

Multiply both sides by to eliminate the fractions to get 3(x+3)=2(x+5) .

Then use the distributive property to get 3x+9=2x+10 .

Now subtract from both sides to get x+9=10 .

Now subtract from both sides to get x=1 .

Report an Error

Example Question #36 : Equations

To mail a package, there is an initial charge of $55\cent$ to cover the first ounce, with another $25\cent$ for each additional ounce. How much does it cost to mail a half pound package?

Possible Answers:

$\$ 3.50$

$\$ 2.30$

$\$ 3.95$

$\$ 4.40$

$\$ 2.00$

Correct answer:

$\$ 2.30$

Explanation:

$1\; pound = 16 \; ounces$ , so we are trying to mail ounces. The total postage becomes $0.55+7(0.25)=0.55+1.75=\$ 2.30$ .

Report an Error

Example Question #1 : Setting Up Expressions

In April, the price of a t-shirt is $\$X$ . In May, the store increases the price by 50%, so that the new price is $\$Y$ . Then in June, the store decreases the price by 50%, so that the t-shirt price is now $\$Z$ . What is the ratio of to ?

Possible Answers:

$\frac{4}{3}$

$\frac{1}{2}$

$\frac{3}{4}$

Correct answer:

$\frac{3}{4}$

Explanation:

If the original price of the T-shirt is , increasing the price by 50% means that the new price is 150% of , or 1.5X .

If the price is then decreased by 50%, the new price is 50% of

$.5\cdot (1.5X)=.75X$

The ratio of to is then:

$\frac{.5*1.5*X}{X}$

The 's in the numerator and denominator cancel, leaving $.5\cdot 1.5$ , or

$\frac{3}{4}$ .

Report an Error

Example Question #1 : Simplifying Expressions

Simplify $\frac{x^4-y^4}{(x-y)^2}\cdot\frac{x-y}{4x^2-4y^2}$ .

Possible Answers:

$\frac{(x^2+y^2)}{4(x-y)}$

$\frac{x-y}{4(x+y)}$

$\frac{x^2-y^2}{4(x+y)}$

$\frac{x-y}{x+y}$

$\frac{(x^2+y^2)}{4(x+y)}$

Correct answer:

$\frac{(x^2+y^2)}{4(x-y)}$

Explanation:

When multiplying rational expressions, we simply have to multiply the numerators together and the denominators together. (Warning: you only need to find a lowest common denominator when adding or subtracting, but not when multiplying or dividing rational expression.)

$\frac{x^4-y^4}{(x-y)^2}\cdot\frac{x-y}{4x^2-4y^2}=\frac{(x-y)(x^4-y^4)}{(x-y)^2\cdot(4x^2-4y^2)}$

In order to simplify this, we will need to factor x^4-y^4 and 4x^2-4y^2 . Because looks a little simpler, let's start with it first.

We can easily factor out a four from both terms.

4x^2-4y^2=4(x^2-y^2) .

Next, notice that x^2-y^2 fits the form of our difference of squares factoring formula. In general, we can factor a^2-b^2 as (a-b)(a+b) . In the polynomial x^2-y^2 we will let a=x and b=y . Thus, x^2-y^2=(x-y)(x+y) .

Now, we can see that 4x^2-4y^2=4(x^2-y^2)=4(x-y)(x+y) .

We then factor x^4-y^4 . This also fits our difference of squares formula; however, this time a=x^2 and b=y^2 . In other words, x^4-y^4=(x^2)^2-(y^2)^2 . Applying the formula, we see that

x^4-y^4=(x^2)^2-(y^2)^2=(x^2-y^2)(x^2+y^2) . Now, let's take our factorization one step further and factor x^2-y^2 , which we already did above.

(x^2-y^2)(x^2+y^2)=(x-y)(x+y)(x^2+y^2) .

Be careful here. A common mistake that students make is to try to factor x^2+y^2 . There is no sum of squares factoring formula. In other words, in general, if we have a^2+b^2 , we can't factor it any further. (It is considered prime.)

We will then put all of these pieces of information in order to simplify our rational expression.

$\frac{(x-y)(x^4-y^4)}{(x-y)^2\cdot(4x^2-4y^2)}=\frac{(x-y)(x-y)(x+y)(x^2+y^2)}{(x-y)^2\cdot(4(x-y)(x+y))}\\ \\ \\ =\frac{(x-y)^2(x+y) (x^2+y^2)}{4(x-y)^3(x+y)}$

Lastly, we cancel the factors that appear in both the numerator and the denominator. We can cancel an x+y and a (x-y)^2 term.

$\frac{(x-y)^2(x+y) (x^2+y^2)}{4(x-y)^3(x+y)}=\frac{(x^2+y^2)}{4(x-y)}$ .

The answer is $\frac{(x^2+y^2)}{4(x-y)}$ .

Report an Error

← Previous 1 2 3 4 5 6 Next →

View Tutors

Jessica
Certified Tutor

Emporia State University, Bachelor, Spanish. Wichita State University, Master's/Graduate, Spanish.

View Tutors

Sekou
Certified Tutor

University of New Haven, Bachelor in Arts, Mathematics.

View Tutors

Nicole
Certified Tutor

University of Wisconsin-Madison, Bachelor in Arts, Spanish.

All High School Math Resources

8 Diagnostic Tests 613 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Chemistry Tutors in Atlanta, Statistics Tutors in New York City, GRE Tutors in Los Angeles, Spanish Tutors in Atlanta, LSAT Tutors in Houston, Reading Tutors in San Francisco-Bay Area, Computer Science Tutors in Washington DC, Math Tutors in Houston, LSAT Tutors in San Diego, Chemistry Tutors in Boston

Popular Courses & Classes

GRE Courses & Classes in San Diego, LSAT Courses & Classes in Seattle, MCAT Courses & Classes in Denver, LSAT Courses & Classes in Miami, SAT Courses & Classes in New York City, LSAT Courses & Classes in Atlanta, SSAT Courses & Classes in Houston, Spanish Courses & Classes in Seattle, SAT Courses & Classes in Miami, GMAT Courses & Classes in Philadelphia

Popular Test Prep

SAT Test Prep in New York City, GMAT Test Prep in Denver, ISEE Test Prep in New York City, MCAT Test Prep in Miami, ISEE Test Prep in San Diego, GMAT Test Prep in Seattle, SSAT Test Prep in Los Angeles, SAT Test Prep in Phoenix, SAT Test Prep in Los Angeles, GRE Test Prep in Houston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

High School Math : Basic Single-Variable Algebra

Study concepts, example questions & explanations for High School Math

All High School Math Resources

Example Questions

Example Question #31 : Equations

Example Question #1 : Systems Of Equations

Example Question #1 : Solving Equations

Example Question #31 : Equations

Example Question #33 : Equations

Example Question #34 : Equations

Example Question #35 : Equations

Example Question #36 : Equations

Example Question #1 : Setting Up Expressions

Example Question #1 : Simplifying Expressions

All High School Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: