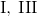All GRE Subject Test: Math Resources
Example Questions
Example Question #3 : Trigonometric Integrals
Evaluate:
1) The 1/2 is a constant, and so is pulled out front.
2) The integral of cos(x) is sin(x), by definition.
3) Writing the limits for evaluation:
4) Using the unit circle, 


5)Simplifying:
Example Question #1 : Sequences & Series
There are 2 series, 


Divergent
Inconclusive
Convergent
Convergent
Infinite series can be added and subtracted with each other.
Since the 2 series are convergent, the sum of the convergent infinite series is also convergent.
Note: The starting value, in this case n=1, must be the same before adding infinite series together.
Example Question #3 : Concepts Of Convergence And Divergence
You have a divergent series 

Inconclusive
Convergent
Divergent
Divergent
This is a fundamental property of series.
For any constant c, if 





Example Question #161 : Calculus
There are 2 series 

Is the sum of these 2 infinite series convergent, divergent, or inconclusive?
Inconclusive
Convergent
Divergent
Convergent
A way to find out if the sum of the 2 infinite series is convergent or not is to find out whether the individual infinite series are convergent or not.
Test the first series

This is a geometric series with 
By the geometric test, this series is convergent.
Test the second series

This is a geometric series with 
By the geometric test, this series is convergent.
Since both of the series are convergent, 
Example Question #162 : Calculus
Find the radius of convergence for the power series
We can use the limit
to find the radius of convergence. We have
This means the radius of convergence is 
Example Question #163 : Calculus
Determine if the following series is divergent, convergent or neither.
Both
Neither
Divergent
Convergent
Inconclusive
Divergent
In order to figure if
is convergent, divergent or neither, we need to use the ratio test.
Remember that the ratio test is as follows.
Suppose we have a series 
Then if



Now lets apply the ratio test to our problem.
Let
and
Now

Now lets simplify this expression to

Since 
we have sufficient evidence to conclude that the series is divergent.
Example Question #1 : Limits Of Sequences
Calculate the sum of the following infinite geometric series:
This is an infinite geometric series.
The sum of an infinite geometric series can be calculated with the following formula,


Solution:
Value of 
r is the value contained in the exponent
Example Question #164 : Gre Subject Test: Math
Determine how many terms need to be added to approximate the following series within 
This is an alternating series test.
In order to find the terms necessary to approximate the series within 
Step 1:
An alternating series can be identified because terms in the series will “alternate” between + and –, because of
Note: Alternating Series Test can only show convergence. It cannot show divergence.
If the following 2 tests are true, the alternating series converges.
-
- {
} is a decreasing sequence, or in other words
Solution:
1.
2. {
Since the 2 tests pass, this series is convergent.
Step 2:
Plug in n values until
4 needs to be added to approximate the sum within 
Example Question #1 : Sequences & Series
Evaluate: 
Step 1: Plug in values into the function and add up the fraction:
Step 2: Find the sum of the fractions....
We can convert the fractions to decimals:
Step 3: Round to 
Example Question #165 : Gre Subject Test: Math
Which of the following are not infinite sequences?
Step 1: Define what an infinite sequence is...
An infinite sequence is a sequence that is non-terminating.
Step 2: Determine if each sequence above is infinite...
For 
For 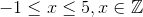
For ![\sqrt[n]{x}; x \in \mathbb{R},n \in \mathbb{N}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/881616/gif.latex)
Certified Tutor
Certified Tutor
All GRE Subject Test: Math Resources







![\frac{1}{2}sin(x)|^{\pi/3}_{0} = \frac{1}{2}\left [ sin(\pi/3)-sin(0)\right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/768326/gif.latex)
![\frac{1}{2}\left [ \frac{\sqrt3}{2}-0\right ]=\frac{\sqrt3}{4}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/768330/gif.latex)








![\small \small \lim_{n\to\infty} \left| \frac{a_{n+1}}{a_n} \right|=\lim \left| \frac{x^{n+1}[(n+1)!]^2}{(2(n+1))!} \frac{(2n!)}{(n!)^2x^n}\right|](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/383920/gif.latex)
![\small \small \small \small =\lim |x|\left| \frac{(2n)!}{(2n+2)!} \frac{[(n+1)!]^2}{(n!)^2}\right|=\lim |x|\left| \frac{(n+1)(n+1)}{(2n+1)(2n+2)}\right|=\frac{|x|}{4}<1](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/383921/gif.latex)













































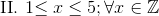
![$III. \sqrt[n]{x}; x \in \mathbb{R},n \in \mathbb{N}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/881613/gif.latex)