All GRE Math Resources
Example Questions
Example Question #4 : How To Find Excluded Values
Find the excluded values of the following algebraic fraction
The numerator cancels all the binomials in the denomniator so ther are no excluded values.
To find the excluded values of a algebraic fraction you need to find when the denominator is zero. To find when the denominator is zero you need to factor it. This denominator factors into
so this is zero when x=4,7 so our answer is
Example Question #241 : Gre Quantitative Reasoning
Find the inverse equation of:
To solve for an inverse, we switch x and y and solve for y. Doing so yields:
Example Question #242 : Gre Quantitative Reasoning
Find the inverse equation of 
1. Switch the 

2. Solve for 
Example Question #243 : Gre Quantitative Reasoning



When 

If 



If 


1. Using any of the two 

Using 
2. Use your new equation 

Example Question #244 : Gre Quantitative Reasoning
|
x |
y |
|
|
|
|
|
|
|
|
|
|
|
|
If 


An inverse variation is a function in the form: 


Substitute each 

Therefore, the constant of variation, 




Example Question #4 : Algebraic Fractions








Because we know 



When 


When 


Therefore, when 

Example Question #1 : How To Evaluate A Fraction
Evaluate the following equation when 
1. Plug in 

2. Perform the above operations.
Example Question #245 : Gre Quantitative Reasoning
Mary walked to school at an average speed of 2 miles per hour and jogged back along the same route at an average speed of 6 miles per hour. If her total traveling time was 1 hour, what was the total number of miles in the round trip?
Since Mary traveled 3 times as quickly coming from school as she did going to school (6 miles per hour compared to 2 miles per hour), we know that Mary spent only a third of the time coming from school as she did going. If x represents the number of hours it took to get to school, then x/3 represents the number of hours it took her to return.
Knowing that the total trip took 1 hour, we have:
x + x/3 = 1
3x/3 + 1x/3 = 1
4x/3 = 1
x = 3/4
So we know it took Mary 3/4 of an hour to travel to school (and the remaining 1/4 of an hour to get back).
Remembering that distance = rate * time, the distance Mary traveled on her way to school was (2 miles per hour) * (3/4 of an hour) = 3/2 miles. Furthermore, since she took the same route coming back, she must have traveled 3/2 of a mile to return as well.
Therefore, the the total number of miles in Mary's round trip is 3/2 miles + 3/2 miles = 6/2 miles = 3 miles.
Example Question #246 : Gre Quantitative Reasoning
If 

To raise 


Example Question #247 : Gre Quantitative Reasoning
Solve ![]()
–1
0
infinitely many solutions
no solution
infinitely many solutions
The common denominator of the left side is x(x–1). Multiplying the top and bottom of 1/x by (x–1) yields
![]()
![]()
![]()
![]()
Since this statement is true, there are infinitely many solutions.
All GRE Math Resources










































































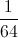




![w^\frac{2}{3}=\sqrt[3]{w^2}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/177545/gif.latex)
![(\frac{1}{8})^\frac{2}{3}=\sqrt[3]{(\frac{1}{8})^2}=\sqrt[3]{\frac{1}{64}}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/177546/gif.latex)
![\sqrt[3]{\frac{1}{64}}=\frac{1}{4}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/177547/gif.latex)




