All GED Math Resources
Example Questions
Example Question #2051 : Ged Math
Approximately what percent of respondents prefers McDonald's?

The percentage of respondents who answered McDonald's is between 

Example Question #2 : Representing Data
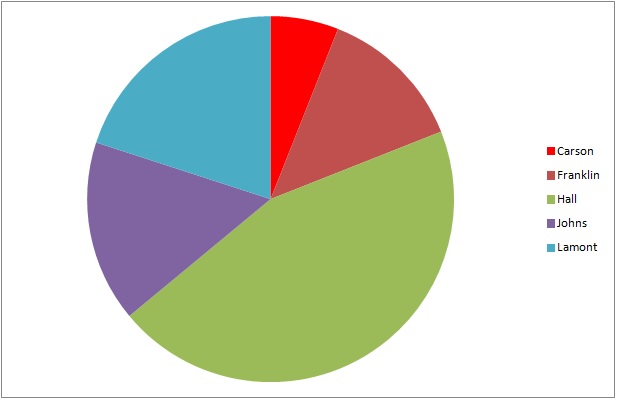
The above graph represents the results of the senior class election at Washington High. According to the rules, the candidate who receives the most votes will become the class president; the candidate who finishes second will become the class vice-president; the candidate who finishes third will become the class secretary-treasurer.
Who will be the secretary-treasurer?
Carson
Lamont
Franklin
Johns
Johns
The wedges, in descending order by size, are those representing Hall, Lamont, Johns, Franklin, Carson. Johns, therefore, finished third, and will be the secretary-treasurer.
Example Question #3 : Representing Data

The above graph represents the results of the student body election at Big State College. According to the rules, if a candidate receives over 50% of the vote, (s)he will win outright. If no candidate receives over 50% of the vote, there will be a runoff election between the top two finishers.
Which of the following is the result of the election?
Hall and Lamont will face each other in a runoff.
Lamont won outright.
Hall and Johns will face each other in a runoff.
Hall won outright.
Hall and Lamont will face each other in a runoff.
The largest wedge of the circle graph is green, which, according to the legend, represents Hall. However, it is less than half of the graph, so he did not win outright. He will face his most successful opponent - Lamont, whose bright blue wedge is the second-largest (though not by much) - in a runoff.
Example Question #1 : Pie Charts

The above represents the results of a student council election at Jefferson High. According to the results, the top three finishers will be awarded seats on the student council.
Which two did not win a seat on the student council?
Franklin and Carson
Lamont and Carson
Franklin and Johns
Johns and Carson
Franklin and Carson
The wedges, in descending order by size, are those representing Hall, Lamont, Johns, Franklin, Carson. Hall, Lamont, and Johns were elected to the student council, so the correct choice is Franklin and Carson.
Example Question #3 : Pie Charts

The above graph represents the results of a general election for two seats on the city council of Wilson City. Frankllin and Hall are Democrats; Johns and Lamont are Republicans; Carson is an independent.
4,987 people voted for Republicans. Which of the following is the best estimate of the number of people who voted in the election overall?
The two wedges that represent the Republican candidates - purple (Johns) and blue (Lamont) - make up about 40% of the circle. The problem becomes, essentially, to figure out what 4,987 is 40% of. This can be done by dividing 4,987 by 0.40:
12,500 is the best estimate.
Example Question #6 : Representing Data

The above graph represents the results of a general election for two seats on the city council of Lincoln City. Frankllin and Hall are Democrats; Johns and Lamont are Republicans; Carson is an independent.
4,429 people voted for Democrats. Which of the following is the best estimate for the number of people who voted for Republicans?
The two wedges that represent the Democratic candidates - green (Hall) and dark red (Franklin) - together make up about 55% of the circle. The two wedges that represent the Republican candidates - purple (Johns) and blue (Lamont) - make up about 40% of the circle. We can estimate the number of people who voted for a Republican using a proportion statement:
Solve for 
The best estimate is 3,200 votes.
Example Question #1 : Representing Data

Refer to the above graph. How many people voted against Vickers?
Do not use a calculator.
Add the number of people who voted for each of the other four candidates:
Example Question #212 : Statistics

Refer to the above graph. Which of the following comes closest to the ratio of people who voted for Zimmerman to people who voted for Ullman?
Divide the number of people who voted for Zimmerman, 512, by the number of people who voted for Ullman, 133.
About four times as many people voted for Zimmerman as Ullman, so of the given choices, 
Example Question #5 : Representing Data

The above circle graph shows the results of a student body election. By the rules, if no candidate wins more than 50% of the vote, there will be a runoff between the top two finishers.
Which of the following questions cannot be answered about the election by examining the above graph?
Who will face each other in the runoff?
How many students did not vote?
How many more votes did Williams get than Vickers?
What percent of the vote did Ullman win?
How many students did not vote?
Ullman's percent of the vote can be calculated by dividing 133 by the sum of the voters of the five candidates. Vickers' number can be subtracted from Willams' number to determine the difference. The two participants in the runoff can be easily seen to be Zimmerman and Williams as they won the most and second-most votes.
However, the question "How many students did not vote?" cannot be answered as the graph only shows the students who did.
Example Question #5 : Representing Data

Do NOT use a calculator for this question.
Refer to the above circle graph.
The top two finishers engage in a runoff election.
The third-place finisher tells his voters to choose the second-place finisher. The fourth- and fifth-place finishers tell their voters to choose the first-place finisher.
If each voter who selected one of the top two finishers stays with his choice, and every other voter does what is requested of him by his original chosen candidate, who will win the election?
Vickers
Ullman
Williams
Zimmerman
Williams
In one group will be those who voted for first-place finisher Zimmerman, fourth-place finisher Ullman, and fifth-place finisher Young. These people will vote for Zimmerman.
In the second group will be those who voted for second-place finisher Williams and third-place finisher Vickers. These people will vote for Williams.
The pieces of the circle representing Vickers and Williams together make up more than half the circle. Therefore, Williams will win the runoff.
Certified Tutor
Certified Tutor
All GED Math Resources































