All GED Math Resources
Example Questions
Example Question #541 : Geometry And Graphs
Find the measure of angle B if it is the supplement to angle A:
If two angles are supplementary, that means the sum of their degrees of measure will add up to 180. In order to find the measure of angle B, subtract angle A from 180 like shown:
This gives us a final answer of 18 degrees for angle B.
Example Question #542 : Geometry And Graphs
Find the measure of angle B if it is the supplement to angle A:
If two angles are supplementary, that means the sum of their degrees of measure will add up to 180. In order to find the measure of angle B, subtract angle A from 180 like shown:
This gives us a final answer of 22 degrees for angle B.
Example Question #1 : Opposite And Corresponding Angles
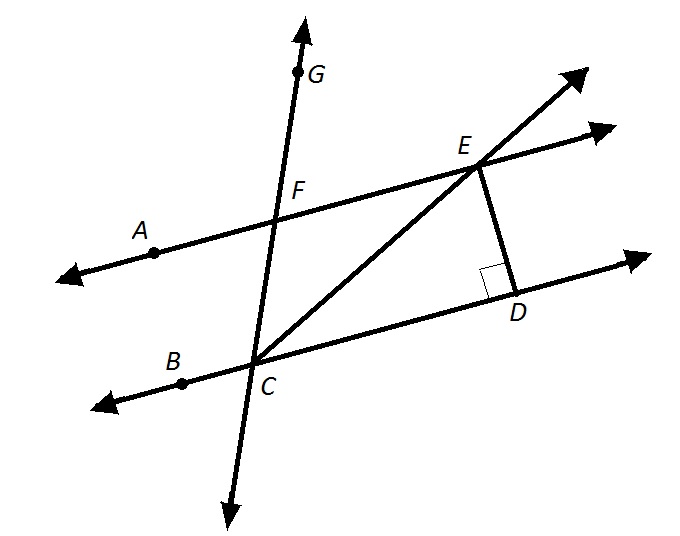
Refer to the above diagram.
Which of the following is a valid alternative name for 
The name of a ray includes two letters, so 
The first letter must be the endpoint. Since 



Example Question #1 : Opposite And Corresponding Angles

Refer to the above diagram.



What is 







We now look at 

Example Question #2 : Opposite And Corresponding Angles

Refer to the above diagram.
Which of the following facts does not, by itself, prove that 





From the Parallel Postulate and its converse, as well as its various resulting theorems, two lines in a plane crossed by a transversal are parallel if any of the following happen:
Both lines are perpendicular to the same third line - this happens if 


Same-side interior angles are supplementary - this happens if 


Alternate interior angles are congruent - this happens if 

However, the fact that 


"

Example Question #4 : Opposite And Corresponding Angles
In two intersecting lines, the opposite angles are 


In an intersecting line, vertical angles are equal to each other.
Set up an equation such that both angles are equal.
Solve for 

Add 14 on both sides.
Divide by 7 on both sides.
The answer is:
Example Question #4 : Opposite And Corresponding Angles
Suppose a pair of opposite angles are measured 


Vertical angles are equal.
Set both angles equal and solve for x.
Subtract 
Add 8 on both sides.
Divide by 4 on both sides.
The answer is:
Example Question #4 : Opposite And Corresponding Angles
Suppose two vertical angles in a pair of intersecting lines. What is the value of 


Vertical angles of intersecting lines must equal to each other.
Set up an equation such that both angle measures are equal.
Add three on both sides.
Divide by three on both sides.
The answer is:
Example Question #4 : Opposite And Corresponding Angles
Suppose two opposite angles are measured 


Opposite angles equal. Set up an equation such that both angle values are equal.
Add 5 on both sides.
Divide by 5 on both sides.
The answer is:
Example Question #4 : Opposite And Corresponding Angles
With a pair of intersecting lines, a set of opposite angles are measured 


Opposite angles of two intersecting lines must equal to each other. Set up an equation such that both angle are equal.
Add 9 on both sides.
Subtract 
This means that 

Certified Tutor
All GED Math Resources


























































































