We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

GED Math : GED Math

Study concepts, example questions & explanations for GED Math

Create An Account

All GED Math Resources

4 Diagnostic Tests 263 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #2 : Squares, Rectangles, And Parallelograms

Pentagons

Refer to the above diagram. Parallel sides are so indicated.

Identify the above polygon.

Possible Answers:

Parallelogram

Trapezoid

Hexagon

Pentagon

Correct answer:

Trapezoid

Explanation:

A four-sided figure, or quadrilateral, with one pair of parallel sides and its other sides nonparallel is called a trapezoid.

Report an Error

Example Question #3 : Perimeter And Sides Of Quadrilaterals

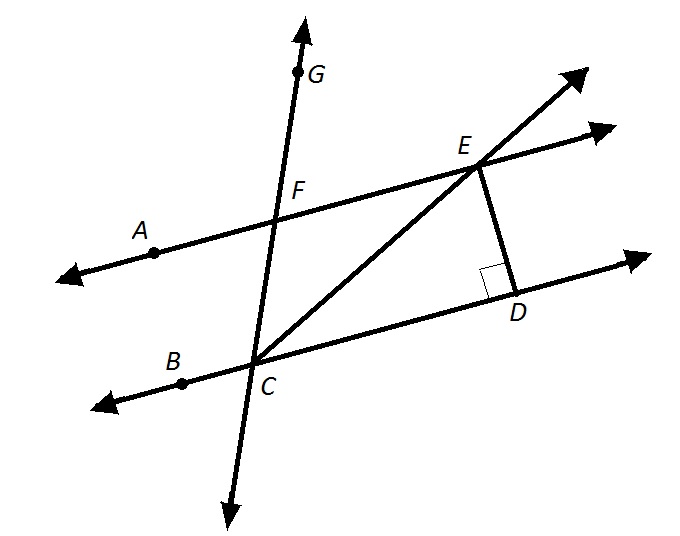
Thingy

Refer to the above figure. You are given that $\overleftrightarrow{AE} ||\overleftrightarrow{BD}$ and that $\angle FCD$ is acute.

Which of the following words accurately describes Polygon EFCD ?

Possible Answers:

Pentagon

Hexagon

Parallelogram

Trapezoid

Correct answer:

Trapezoid

Explanation:

Polygon EFCD has four sides and is therefore a quadrilateral. $\overleftrightarrow{AE} ||\overleftrightarrow{BD}$ , so $\overline{FE }|| \overline{CD}$ . Also, since $\angle FCD$ is acute and $\angle CDE$ is right, $\angle FCD \ncong \angle CDE$ , so $\overline{FC } \nparallel \overline{DE}$ .

The quadrilateral has one pair of parallel sides, and the other two sides are not parallel. Therefore, it is a trapezoid.

Report an Error

Example Question #5 : Squares, Rectangles, And Parallelograms

Pentagons

Refer to the above three figures. All parallel sides are so indicated.

Which of the figures can be called a parallelogram?

Possible Answers:

Figures A, B, and C

Figures A and B only

Figure B only

Figure C only

Correct answer:

Figures A and B only

Explanation:

A parallelogram, by definition, has two pairs of parallel sides. Figures A and B fit that criterion, but Figure C does not.

Report an Error

Example Question #2 : Squares, Rectangles, And Parallelograms

Garden

Note: Figure NOT drawn to scale.

Refer to the above figure, which shows a rectangular garden (in green) surrounded by a dirt path (in brown). The dirt path is feet wide throughout. Which of the following polynomials gives the perimeter of the garden?

Possible Answers:

320- 4x

320-8x

160-4x

160- 8x

Correct answer:

320-8x

Explanation:

The length of the garden is than that of the entire lot, or

L = 100 - 2x .

The width of the garden is than that of the entire lot, or

W = 60 - 2x .

The perimeter is twice the sum of the two:

P = 2 (L + W)

$= 2 (100-2x+60-2x) = 2\left ( 160-4x\right )= 320-8x$

Report an Error

Example Question #3 : Squares, Rectangles, And Parallelograms

Rhombus

Note: Figure NOT drawn to scale.

Quadrilateral ABCD is a rhombus. Calculate its perimeter if:

AC = 24

BD = 10

Possible Answers:

Correct answer:

Explanation:

The four sides of a rhombus are congruent. Also, the diagonals of a rhombus are perpendicular bisectors to each other, so the four triangles they form are right triangles. Therefore, the Pythagorean theorem can be used to determine the common sidelength of Quadrilateral ABCD .

We focus on $\Delta AXB$ . The diagonals of a rhombus, as is the case with any parallelogram, are each the other's bisector, so

$AX = \frac{1}{2} (AC) = \frac{1}{2} \cdot 24 = 12$

$XB = \frac{1}{2} (BD) = \frac{1}{2} \cdot 10 = 5$

By the Pythagorean Theorem,

$AB = \sqrt{(AX)^{2}+ (XB)^{2}} = \sqrt{12^{2}+5^{2}} = \sqrt{144+25} = \sqrt{169} = 13$

13 is the common length of the four sides of Quadrilateral ABCD , so its perimeter is $4 \times 13 = 52$ .

Report an Error

Example Question #5 : Perimeter And Sides Of Quadrilaterals

Rectangle

Note: Figure NOT drawn to scale

AB = 14, BC = 6, AD = 7, DF = 3

Give the ratio of the perimeter of Rectangle ACGF to that of Rectangle ABED .

Possible Answers:

$4\textrm{ to }3$

$100\textrm{ to }49$

$10 \textrm{ to }7$

$1\textrm{ to }1$

Correct answer:

$10 \textrm{ to }7$

Explanation:

The perimeter of Rectangle ABED is

P = AB +BE + ED + AD .

Opposite sides of a rectangle are congruent, so

AB= ED = 14, AD = BE = 7

and

P = 14 + 7 + 14 + 7 = 42 .

The perimeter of Rectangle ACGF is

P = AC + CG + GF + AF .

Opposite sides of a rectangle are congruent, so

GF = AC = AB + BC = 14 + 6 = 20 ,

CG = AF = AD + DF = 7 + 3 = 10 ,

and

P = 20 + 10 + 20 + 10 = 60 .

The ratio of the perimeters is

$\frac{60}{42} = \frac{60\div 6}{42\div 6} =\frac{10}{7}$ - that is, 10 to 7.

Report an Error

Example Question #11 : Perimeter And Sides Of Quadrilaterals

Garden

Note: Figure NOT drawn to scale

Refer to the above figure, which shows a rectangular garden (in green) surrounded by a dirt path (in brown). The dirt path is six feet wide throughout. Which of the following polynomials gives the perimeter of the garden, in feet?

Possible Answers:

6x-48

3x-24

6x-24

3x-48

Correct answer:

6x-48

Explanation:

The length of the garden, in feet, is $2 \times 6 = 12$ feet less than that of the entire lot, or

L = 2x-12 .

The width of the garden, in feet, is $2 \times 6 = 12$ less than that of the entire lot, or

W = x-12 .

The perimeter, in feet, is twice the sum of the two:

P = 2 (L + W)

= 2 (2x-12 + x - 12 )= 2 (3x-24) = 6x - 48

Report an Error

Example Question #1264 : Ged Math

Given Square ABCD , the distance from to is one mile. Which of the following gives the perimeter of the square, to the nearest foot?

Possible Answers:

$29,868\textrm{ ft}$

$10,560\textup{ ft}$

$7,467\textrm{ ft}$

$14,934\textrm{ ft}$

Correct answer:

$14,934\textrm{ ft}$

Explanation:

The segment $\overline{AC}$ is a diagonal of the square. It is also the hypotenuse of a right triangle whose legs both measure the same length, which we will call ; this is also the length of one side of the square.

The right triangle formed is an isosceles triangle - and, subsequently, a $45^{\circ }-45^{\circ }-90^{\circ }$ triangle - and therefore,

$s \sqrt{2} = AC$

$\overline{AC}$ is one mile, or 5,280 feet, long, so we substitute AC = 5,280 and solve for :

$s \sqrt{2} = 5,280$

$s = 5,280 \div \sqrt{2} \approx 5,280 \div 1.414 \approx 3,733.5$

The perimeter, in feet, is four times this, or

$P = 4s \approx 4 \times 3,733.5 \approx 14,934$ feet.

Report an Error

Example Question #11 : Perimeter And Sides Of Quadrilaterals

The length of each side of a square is increased by 12%. By what percent has its perimeter increased?

Possible Answers:

$48 \%$

$44 \%$

$24 \%$

$12 \%$

Correct answer:

$12 \%$

Explanation:

Let be the original sidelength of the square. Increasing this by 12% is the same as adding 0.12 times that sidelength to the original sidelength. The new sidelength is therefore

s + 0.12s = 1.12s .

The perimeter of a square is four times its sidelength. Its original perimeter was ; after the increase, it is $4 \cdot 1.12s = 4.48 s$ . The percent of increase is therefore

$\frac{4.48s - 4s }{4s} \times 100 \% = \frac{0.48 s}{4s} \times 100 \% = 0.12 \times 100 \% = 12 \%$ .

Report an Error

Example Question #1261 : Ged Math

The perimeter of a square is 24cm. Find the length of one side of the square.

Possible Answers:

$\text{There is not enough information to answer the question.}$

$3\text{cm}$

$7\text{cm}$

$4\text{cm}$

$6\text{cm}$

Correct answer:

$6\text{cm}$

Explanation:

The formula to find the perimeter of a square is

P = 4a

where a is the length of any side of the square. Because a square has 4 equal sides, we can use any side in the formula. To find the length of one side of the square, we will solve for a.

Now, we know the perimeter of the square is 24cm. So, we will substitute and solve for a. We get

$24\text{cm} = 4a$

$\frac{24\text{cm}}{4} = \frac{4a}{4}$

$6\text{cm} = a$

$a = 6\text{cm}$

Therefore, the length of one side of the square is 6cm.

Report an Error

View Tutors

Alexandra
Certified Tutor

Rice University, Bachelor in Arts, Biochemistry and Molecular Biology. University of North Texas Health Science Center, Maste...

View Tutors

Xenia
Certified Tutor

Boston University, Bachelor of Science, Business Administration and Management. Columbia University in the City of New York, ...

View Tutors

Jonathan
Certified Tutor

University of Illinois at Chicago, Bachelor in Arts, Criminal Justice. Syracuse University, Masters in Business Administratio...

All GED Math Resources

4 Diagnostic Tests 263 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

ISEE Tutors in Houston, SAT Tutors in Dallas Fort Worth, GRE Tutors in Los Angeles, LSAT Tutors in Los Angeles, LSAT Tutors in Washington DC, SSAT Tutors in Denver, Math Tutors in Denver, MCAT Tutors in New York City, Algebra Tutors in Boston, French Tutors in Atlanta

Popular Courses & Classes

ISEE Courses & Classes in Houston, MCAT Courses & Classes in Atlanta, GMAT Courses & Classes in Phoenix, SAT Courses & Classes in Denver, GMAT Courses & Classes in Chicago, SAT Courses & Classes in Phoenix, SSAT Courses & Classes in New York City, GRE Courses & Classes in Chicago, MCAT Courses & Classes in Denver, LSAT Courses & Classes in Seattle

Popular Test Prep

GMAT Test Prep in Phoenix, SSAT Test Prep in Seattle, GRE Test Prep in Seattle, SAT Test Prep in Boston, GMAT Test Prep in San Diego, SAT Test Prep in San Francisco-Bay Area, LSAT Test Prep in Boston, SAT Test Prep in Seattle, GMAT Test Prep in Chicago, SSAT Test Prep in Chicago

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

GED Math : GED Math

Study concepts, example questions & explanations for GED Math

All GED Math Resources

Example Questions

Example Question #2 : Squares, Rectangles, And Parallelograms

Example Question #3 : Perimeter And Sides Of Quadrilaterals

Example Question #5 : Squares, Rectangles, And Parallelograms

Example Question #2 : Squares, Rectangles, And Parallelograms

Example Question #3 : Squares, Rectangles, And Parallelograms

Example Question #5 : Perimeter And Sides Of Quadrilaterals

Example Question #11 : Perimeter And Sides Of Quadrilaterals

Example Question #1264 : Ged Math

Example Question #11 : Perimeter And Sides Of Quadrilaterals

Example Question #1261 : Ged Math

All GED Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: