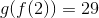This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the inside function is in the form  and the question asks to calculate  we know that the input value is two.

After the function value of  is calculated, it will be the input value for .

Step 2: Given the function, input the desired  value. 

In step one the input value of two was found. Now substitute 2 in for every in the function .

Step 3: Substitute the function value of the inside portion into .

This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the function is in the form  and the question asks to calculate  we know that the input value is two.

Step 2: Given the function, input the desired  value. 

In step one the input value of two was found. Now substitute 2 in for every in the function.

Step 3: Answer the question.

This particular function when solved algebraically results in one value.

The answer is

This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the inside function is in the form  and the question asks to calculate  we know that the input value is negative two.

After the function value of  is calculated, it will be the input value for .

Step 2: Given the function, input the desired  value. 

In step one the input value of negative two was found. Now substitute -2 in for every in the function .

Step 3: Substitute the function value of the inside portion into .

This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the function is in the form  and the question asks to calculate  we know that the input value is negative three.

Step 2: Given the function, input the desired  value. 

In step one the input value of negative three was found. Now substitute negative three in for every  in the function.

Step 3: Answer the question.

This particular function when solved algebraically results in one value.

The answer is

.

This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the function is in the form  and the question asks to calculate  we know that the input value is six.

Step 2: Given the function, input the desired  value. 

In step one the input value of six was found. Now substitute 6 in for every in the function.

Step 3: Verify solution by graphing the function.

To find the function value on a graph go to  and find the y value that lies on the graph at . Looking at the graph above when , .

Recall that , thus verifying the solution found in step 2.

This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the function is in the form  and the question asks to calculate  we know that the input value is five.

Step 2: Given the function, input the desired  value. 

In step one the input value of five was found. Now substitute 5 in for every  in the function.

Step 3: Verify solution by graphing function.

To find the function value on a graph go to  and find the y value that lies on the graph at . Looking at the graph above when , .

Recall that , thus verifying the solution found in step 2.

This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the function is in the form  and the question asks to calculate  we know that the input value is one.

Step 2: Given the function, input the desired  value. 

In step one the input value of one was found. Now substitute 1 in for every  in the function.

Since a fraction cannot have a zero in the denominator, the function value at  does not exist.

Step 3: Verify solution by graphing function.

Recall that graphically, a vertical asymptote represents where the function does not exist.

Looking at the above graph the function does not exist at  thus verifying the solution found in step 2.

This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the function is in the form  and the question asks to calculate  we know that the input value is two.

Step 2: Given the function, input the desired  value. 

In step one the input value of two was found. Now substitute 2 in for every  in the function.

Step 3: Verify solution by graphing the function.

To find the function value on a graph go to  and find the y value that lies on the graph at . Looking at the graph above when , .

Recall that , thus verifying the solution found in step 2.

This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the function is in the form  and the question asks to calculate  we know that the input value is four.

Step 2: Given the function, input the desired  value. 

In step one the input value of four was found. Now substitute 4 in for every  in the function.

Step 3: Verify solution by graphing the function.

To find the function value on a graph go to  and find the y value that lies on the graph at . Looking at the graph above when , .

Recall that , thus verifying the solution found in step 2.

This question is testing the knowledge and skills of calculating a function value. Similar to domain and range, calculating the function value requires the application of input values into the function to find the output value. In other words, evaluating a function at a particular value results in the function value; which is another way of saying function value is the output. 

For the purpose of Common Core Standards, function notation and evaluation fall within the Cluster A of the function and use of function notation concept (CCSS.MATH.CONTENT.HSF-IF.A). 

Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.

Step 1: Identify the input value.

Since the inside function is in the form  and the question asks to calculate  we know that the input value is two.

After the function value of  is calculated, it will be the input value for .

Step 2: Given the function, input the desired  value. 

In step one the input value of two was found. Now substitute 2 in for every in the function .

Step 3: Substitute the function value of the inside portion into .