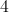Common Core: 8th Grade Math : Geometry
Study concepts, example questions & explanations for Common Core: 8th Grade Math
All Common Core: 8th Grade Math Resources
Example Questions
Example Question #5 : Explain A Proof Of The Pythagorean Theorem And Its Converse: Ccss.Math.Content.8.G.B.6
If the equation 
The Pythagorean Theorem only works if the hypotenuse is an odd number
The Pythagorean Theorem only works if the hypotenuse is an even number
A right triangle has a hypotenuse of 
A right triangle has a hypotenuse of 
A right triangle has a hypotenuse of
The equation shown in the question, 
In this equation:
This means that 
Example Question #6 : Explain A Proof Of The Pythagorean Theorem And Its Converse: Ccss.Math.Content.8.G.B.6
If the equation 
A right triangle has a hypotenuse of 
The Pythagorean Theorem only works if the hypotenuse is an odd number
The Pythagorean Theorem only works if the hypotenuse is an even number
A right triangle has a hypotenuse of 
A right triangle has a hypotenuse of
The equation shown in the question, 
In this equation:
This means that 
Example Question #7 : Explain A Proof Of The Pythagorean Theorem And Its Converse: Ccss.Math.Content.8.G.B.6
If the equation 
A right triangle has a hypotenuse of 
A right triangle has a hypotenuse of 
The Pythagorean Theorem only works if the hypotenuse is an even number
The Pythagorean Theorem only works if the hypotenuse is an odd number
A right triangle has a hypotenuse of
The equation shown in the question, 
In this equation:
This means that 
Example Question #3 : Explain A Proof Of The Pythagorean Theorem And Its Converse: Ccss.Math.Content.8.G.B.6
If the equation 
The Pythagorean Theorem only works if the hypotenuse is an even number
A right triangle has a hypotenuse of 
The Pythagorean Theorem only works if the hypotenuse is an odd number
A right triangle has a hypotenuse of 
A right triangle has a hypotenuse of
The equation shown in the question, 
In this equation:
This means that 
Example Question #91 : Geometry
Can the Pythagorean Theorem be used to solve for the missing side length in the triangle provided?
No because the triangle doesn't provide enough information
Yes because the triangle is a right triangle
No because the triangle is not a right triangle
Yes because the triangle is only missing one side length
No because the triangle is not a right triangle
The Pythagorean Theorem states that for right triangles, the square of the hypotenuse is equal to the sum of the square of the other two sides. In other terms:
However, the triangle provided is not a right triangle because there is not an angle that is equal to 
Example Question #12 : Explain A Proof Of The Pythagorean Theorem And Its Converse: Ccss.Math.Content.8.G.B.6
Which answer choice provides side lengths of a right triangle?
In order to solve this problem, we use the converse of the Pythagorean Theorem. We will substitute the given side lengths to determine which three side lengths make the formula for Pythagorean Theorem true. It is important to remember that the hypotenuse will always be the longest side length, so the value for 
Let's plug in the side lengths into our formula and solve:
This means that a triangle that has side lengths of 
Example Question #92 : Geometry
The base and height of a right triangle are each 1 inch. What is the hypotenuse?
You need to use the Pythagorean Theorem, which is 
Add the first two values and you get 
Example Question #2 : How To Find The Length Of The Hypotenuse Of A Right Triangle : Pythagorean Theorem
A right triangle has legs with lengths of 
Using the numbers given to us by the question,
Example Question #2 : How To Find The Length Of The Hypotenuse Of A Right Triangle : Pythagorean Theorem
A right triangle has legs with the lengths 
Use the Pythagorean Theorem to find the length of the hypotenuse.
Example Question #2 : Apply The Pythagorean Theorem To Determine Unknown Side Lengths In Right Triangles: Ccss.Math.Content.8.G.B.7
Find the length of the hypotenuse in the right triangle below.
Use the Pythagorean Theorem to find the hypotenuse.
All Common Core: 8th Grade Math Resources
