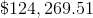The periodic compound interest formula is

where  is the amount of money in the account at the end of the period,  is the principal at the beginning,  is the annual interest rate in decimal form,  is the number of periods per year at which the interest is compounded, and  is the number of years over which the interest accumulates. 

Since Sheila deposits $1,000 per month, we apply this formula twelve times, with  equal to the principal at the beginning of each successive month, ,  (monthly interest), and .

We can go ahead and calculate , since , , and  remain constant:

.

The formula can be rewritten as 

At the beginning of January, Sheila deposits $10,000. At the end of January, there is

in the account.

At the beginning of February, she again deposits $10,000, so there is now

in the account.

At the end of February, there is 

in the account.

Repeat addition of $10,000, then multiplication by  1.006333, ten more times to get the amount of money in the account at the end of December. This will be $125.056.56

Use the formula for compound interest to solve: 

"A" is the amount of interest, "P" is the initial amount invested, "r" is the interest rate, "m" is the number of times per year the interest is compounded, and "t" is the number of years the money is invested.

Plug in the appropriate values for the equation: 

Because "t" is measure in years, 57 months needs to be converted to years (i.e. 57 months=4.75 years)  to solve this equation

Simplify the equation: 

Solution: 

Which of the following will form a circle when graphed?

The equation of a circle follows the general formula of

Where r is the radius of the circle, and (h,k) are the coordinates of the center of the circle.

If we examine our answer choices, only 

follows this form.

The standard form of a circle is the following:

where r is the radius and (a, b) is the center. In order to rework what we have into this form, we have to complete the square.

Since 

we need to find an a so that 2a=4.

 .

For the y coordinate, we need to find the same thing except instead we have the equation 2b=6.

.

And then we have the coordinates of the circle's center: 

The -intercept of the graph of  occurs at the point at which  - that is, at the point with coordinates . By definition,  if , so we want to find this value. To do this, substitute accordingly:

Solve for  by isolating it on the left; first, subtract 15:

Divide by 7:

The -intercept of the graph of   is at .

The -intercept of the graph of a function is the point at which it intersects the -axis. The -coordinate is 0, so the -coordinate can be found by evaluating .

The function is piecewise-defined, so it is necessary to use the definition applicable for . However, the first definition applies for values of  less than 0, and the second, for values greater.  is undefined, and the graph of  has no -intercept.

The -intercept of the graph of a function is the point at which it intersects the -axis. The -coordinate is 0, so the -coordinate can be found by solving the equation 

This necessitates setting both definitions of  equal to 0 and solving for . For the first definition:

 for 

Add 16:

By the Square Root Property:

 or 

Since this definition holds only for , we only select .

For the second definition:

 for 

Add 25:

By the Square Root Property:

 or 

Since this definition holds only for , we only select .

Therefore, the graph has two -intercepts, which are at  and .

The -intercept of the graph of a function is the point at which it intersects the -axis. The -coordinate is 0, so the -coordinate can be found by solving the equation 

This necessitates setting both definitions of  equal to 0 and solving for . For the first definition:

 for 

However, this definition only holds for values less than 0. No solution is yielded.

For the second definition:

 for 

However, this definition only holds for values greater than or equal to 0. No solution is yielded.

Therefore,  has no solution, and the graph of  has no -intercepts.

The -intercept of the graph of a function is the point at which it intersects the -axis. The -coordinate is 0, so the -coordinate can be found by evaluating .

The function is piecewise-defined, so it is necessary to use the definition applicable for . Since , and  for . this is the definition to use.

The -intercept of the graph is the point .

If two distinct lines intersect at the point  - that is, if both pass through this point - it follows that  is a solution of the equations of both. Therefore, set  in both equations and determine whether they are true or not.

True -  is a solution of this equation.

True -  is a solution of this equation.

Therefore,  is a solution of both equations, and the lines intersect at this point.