Call Now to Set Up Tutoring:

(888) 888-0446

Calculus 2 : Ratio Test

Study concepts, example questions & explanations for Calculus 2

Create An Account

All Calculus 2 Resources

9 Diagnostic Tests 308 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

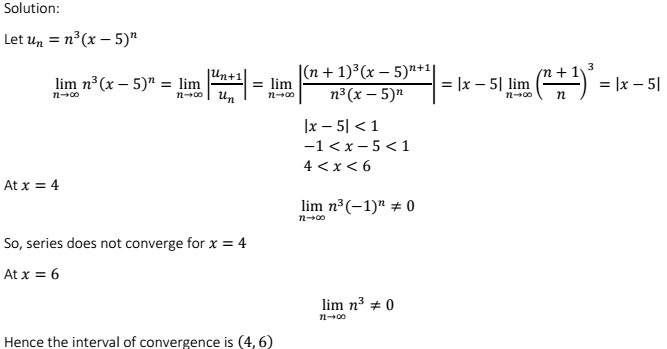
Example Question #111 : Ratio Test

Find the interval of convergence of the following series

Possible Answers:

(4,6)

[4,6)

[4,6]

Correct answer:

(4,6)

Explanation:

Untitled

Report an Error

Example Question #111 : Ratio Test

Determine if the series converges or diverges:

$\sum_{n=0}^{\infty} \frac{4^{n+2}(n!)}{3^n}$

Possible Answers:

The series may absolutely converge, conditionally converge, or diverge

The series converges

The series diverges

The series conditionally converges

Correct answer:

The series diverges

Explanation:

To determine the convergence of the series, we must use the ratio test, which states that for the series $\sum_{n=0}^{\infty} a_n$ , and $L=\lim_{n\rightarrow \infty} \left | \frac{a_{n+1}}{a_n} \right |$ , if L is greater than 1, the series diverges, if L is equal to 1, the series may absolutely converge, conditionally converge, or diverge, and if L is less than 1 the series is (absolutely) convergent.

For our series, we get

$L=\lim_{n\rightarrow \infty} \left | \frac{4^{n+3}(n+1)!}{3^{n+1}}\cdot \frac{ 3^n}{n!(4^{n+2})}\right |$

Using the properties of radicals and exponents to simplify, we get

$\lim_{n\rightarrow \infty}\frac{4(n+1)}{3}=\infty>1$

L is greater than 1 so the series is divergent.

Report an Error

Example Question #111 : Ratio Test

Determine whether the series converges or diverges:

$\sum_{n=0}^{\infty}\frac{n^{2}3^n}{n!}$

Possible Answers:

The series conditionally converges

The series converges

The series may absolutely converge, conditionally converge, or diverge

The series diverges

Correct answer:

The series converges

Explanation:

For our series, we get

$L=\lim_{n\rightarrow \infty} \left | \frac{(n+1)^2(3^{n+1})}{(n+1)!}\cdot \frac{n!}{n^2\cdot 3^n} \right |$

Using the properties of radicals and exponents to simplify, we get

$\lim_{n\rightarrow \infty}\frac{3(n+1)}{n^2}=0<1$

L is less than 1 so the series is convergent.

Report an Error

Example Question #114 : Ratio Test

Use the ratio test on the given series and interpret the result.

$\small \sum_{n=0}^{\infty}\frac{(-1)^{2n+1}(3n-2)}{4^{2n+1}}$

Possible Answers:

The series is conditionally convergent.

The series is absolutely convergent, and therefore convergent

The series is divergent

The series is either divergent, conditionally convergent, or absolutely convergent.

The series is convergent, but not absolutely convergent.

Correct answer:

The series is absolutely convergent, and therefore convergent

Explanation:

$\small \sum_{n=0}^{\infty}\frac{(-1)^{2n+1}(3n-2)}{4^{2n+1}}$

_____________________________________________________________

The ratio test can be used to prove that an infinite series $\small \sum_{n=0}^{\infty}a_n$ is convergent or divergent. In some cases, however, the ratio test may be inconclusive.

Define:

$\small L= \lim_{n->\infty}\left | \frac{a_{n+1}}{a_n}\right |$

If...

$\small L <1$ then the series is absolutely convergent, and therefore convergent.

$\small L>1$ then the series is divergent,

$\small L=1$ then the series is either conditionally convergent, absolutely convergent, or divergent.

______________________________________________________________

To compute the limit, we first need to write the expression for $\small a_{n+1}$ .

$\small \small a_{n+1}=\frac{(-1)^{2(n+1)+1}[3(n+1)-2]}{4^{2(n+1)+1}}$

$\small \small \small a_{n+1}=\frac{(-1)^{2n+3}(3n+1)}{4^{2n+3}}$

Now we can find the limit,

$\small \small L= \lim_{n->\infty}\left | \frac{a_{n+1}}{a_n}\right |$

$\small L=\small \small \lim_{n->0}\left |\frac{ (-1)^{2n+3}(3n+1)}{4^{2n+3}}\times \frac{4^{2n+1}}{(-1)^{2n+1}(3n-2)}\right |$

We can cancel all factors with the exponent.

$\small L=\small \small \lim_{n->0}\left |\frac{ (-1)^{3}(3n+1)}{4^{3}}\times \frac{4^1}{(-1)^1(3n-2)}\right |$

Now the negative factor in the numerator (-1)^3 = -1 will cancel with in the denominator, although this is trivial since we are taking the absolute value regardless.

Continue simplifying,

$L = \lim_{n->0}\left | \frac{3n+1}{16}\times \frac{1}{3n-2}\right |$

$L = \lim_{n->0}\left | \frac{3n+1}{48n-32}\right |=\frac{3}{48}=\frac{1}{16}$

Therefore,

L<1

This proves that the series $\small \sum_{n=0}^{\infty}\frac{(-1)^{2n+1}(3n-2)}{4^{2n+1}}$ is absolutely convergent, and therefore convergent.

Report an Error

Example Question #2931 : Calculus Ii

For the following series, perform the ratio test and interpret the results.

$\small \sum_{n=0}^{\infty}(-1)^n(6n^2+1)$

Possible Answers:

The series is either conditionally convergent, absolutely convergent, or divergent.

Divergent

Absolutely Convergent

Conditionally Convergent

Correct answer:

The series is either conditionally convergent, absolutely convergent, or divergent.

Explanation:

$\small \sum_{n=0}^{\infty}(-1)^n(6n^2+1)$

______________________________________________________________

The ratio test can be used to prove that an infinite series $\small \sum_{n=0}^{\infty}a_n$ is convergent or divergent. In some cases, however, the ratio test may be inconclusive.

Define:

$\small L= \lim_{n->\infty}\left | \frac{a_{n+1}}{a_n}\right |$

If...

$\small L <1$ then the series is absolutely convergent, and therefore convergent.

$\small L>1$ then the series is divergent,

$\small L=1$ then the series is either conditionally convergent, absolutely convergent, or divergent.

______________________________________________________________

$L=\lim_{n->\infty}\left |\frac{a_{n+1}}{a_n} \right |= \lim_{n->\infty}\left |\frac{ (-1)^{n+1}[6(n+1)^2+1]}{(-1)^n(6n^2+1)} \right |$

$=\lim_{n->\infty}\left | \frac{(-1) (6n^2+12n+7)}{6n^2+1} \right|$

$=\lim_{n->\infty} \frac{6n^2+12n+7}{6n^2+1}$

Divide above and below by n^2 , in the limit the all terms disappear except for the coefficients on the leading terms. The limit is therefore equal to $\frac{6}{6}=1$

L = 1

Therefore, the series is either conditionally convergent, absolutely convergent, or divergent.

Report an Error

View Calculus 2 Tutors

Chelsea
Certified Tutor

The University of Texas at Dallas, Bachelor of Science, Physics. The University of Texas at Dallas, Master of Science, Bioinf...

View Calculus 2 Tutors

Sumit
Certified Tutor

Calcutta University, Bachelor of Science, Mathematics. Indian Statistical Institute, Master of Science, Mathematics.

View Calculus 2 Tutors

Jason
Certified Tutor

Brigham Young University, Bachelor, Neuroscience.

All Calculus 2 Resources

9 Diagnostic Tests 308 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

GRE Tutors in Philadelphia, ACT Tutors in Denver, Algebra Tutors in Houston, ACT Tutors in Houston, French Tutors in Boston, Reading Tutors in San Francisco-Bay Area, MCAT Tutors in San Francisco-Bay Area, Statistics Tutors in Miami, Math Tutors in Chicago, English Tutors in New York City

Popular Courses & Classes

SSAT Courses & Classes in Seattle, GMAT Courses & Classes in Boston, Spanish Courses & Classes in New York City, LSAT Courses & Classes in Miami, GMAT Courses & Classes in Phoenix, ISEE Courses & Classes in Phoenix, ACT Courses & Classes in Miami, LSAT Courses & Classes in Boston, MCAT Courses & Classes in Philadelphia, SSAT Courses & Classes in Houston

Popular Test Prep

ISEE Test Prep in Washington DC, SAT Test Prep in Chicago, GRE Test Prep in Chicago, SSAT Test Prep in Chicago, MCAT Test Prep in Chicago, ACT Test Prep in Miami, SAT Test Prep in Los Angeles, GMAT Test Prep in Phoenix, GMAT Test Prep in Denver, GRE Test Prep in Miami

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Calculus 2 : Ratio Test

Study concepts, example questions & explanations for Calculus 2

All Calculus 2 Resources

Example Questions

Example Question #111 : Ratio Test

Example Question #111 : Ratio Test

Example Question #111 : Ratio Test

Example Question #114 : Ratio Test

Example Question #2931 : Calculus Ii

All Calculus 2 Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: