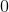We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Calculus 2 : Derivative Review

Study concepts, example questions & explanations for Calculus 2

Create An Account

All Calculus 2 Resources

9 Diagnostic Tests 308 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Derivative Review

Evaluate the limit using one of the definitions of a derivative.

$\lim_{x \rightarrow -\frac{\pi}{2}} \frac{\sin^2x -1}{x+\frac{\pi}{2}}$

Possible Answers:

Does not exist

$\frac{1}{2}$

$\pi$

Correct answer:

Explanation:

Evaluating the limit directly will produce an indeterminant solution of $\small \tiny{\frac{0}{0}}$ .

The limit definition of a derivative is $\lim_{h \rightarrow 0} \frac{f(x+h)-f(x)}{h}=f'(x)$ . However, the alternative form, $\lim_{x \rightarrow a} \frac{f(x)-f(a)}{x-a}=f'(x)$ , better suits the given limit.

Let $f(x)=\sin^2x$ and notice $f\left(-\frac{\pi}{2}\right)=1$ . It follows that ${\lim_{x \rightarrow -\frac{\pi}{2}} \frac{\sin^2-1}{x-\left(-\frac{\pi}{2} \right )} = \frac{d}{dx}\sin^2x}$ .

Thus, the limit is ${2\sin\left(-\frac{\pi}{2} \right ) \cos\left(-\frac{\pi}{2}\right)} = 0.$

Report an Error

Example Question #2 : Derivative Review

Evaluate the limit using one of the definitions of a derivative.

$\lim_{x \rightarrow 1} \frac{x^{49}-1}{x-1}$

Possible Answers:

Does not exist

Correct answer:

Explanation:

Evaluating the derivative directly will produce an indeterminant solution of $\small \tiny{\frac{0}{0}}$ .

Let $f(x)=x^{49}$ and notice f(1)=1 . It follows that ${\lim_{x \rightarrow 1} \frac{x^{49}-1}{x-1} = \frac{d}{dx}x^{49}}$ . Thus, the limit is $49(1)^{48}=49$ .

Report an Error

Example Question #3 : Derivative Review

Suppose and are differentiable functions, and f(3)=1, f'(3)=4, g(-1)=3, g'(-1)=-1 . Calculate the derivative of h(x) = f(x+2)g(-x) , at x = 1

Possible Answers:

None of the other answers

Correct answer:

None of the other answers

Explanation:

The correct answer is 11.

Taking the derivative of h(x) involves the product rule, and the chain rule.

h'(x)=[f(x+2)][-g'(-x)]+[g(-x)][f'(x+2)]

= -g'(-x)f(x+2)+g(-x)f'(x+2)

Substituting x=1 into both sides of the derivative we get

$h'(1)= -g'(-1)f(3)+g(-1)f'(3) = -1\cdot1 + 3 \cdot 4 = 11$ .

Report an Error

Example Question #4 : Definition Of Derivative

Evaluate the limit

$\small \small \lim_{\mu\to 0} \frac{\tan \mu}{\mu}$

without using L'Hopital's rule.

Possible Answers:

$\small \frac{\sqrt{2}}{2}$

$\small -\frac{\sqrt{2}}{2}$

$\small 2$

$\small 1$

Correct answer:

$\small 1$

Explanation:

If we recall the definition of a derivative of a function $\small f(x)$ at a point $\small x$ , one of the definitions is

$\small f'(x)=\lim_{h\to 0}\frac{f(x+h)-f(x)}{h}$ .

If we compare this definition to the limit

$\small \small \lim_{\mu\to 0} \frac{\tan \mu}{\mu}$

we see that that this is the limit definition of a derivative, so we need to find the function $\small f(x)$ and the point $\small x$ at which we are evaluating the derivative at. It is easy to see that the function is $\small f(x)=\tan x$ and the point is $\small x=0$ . So finding the limit above is equivalent to finding $\small f'(0)$ .

We know that the derivative is $\small f'(x)=\sec^2(x)$ , so we have

$\small \small \small \lim_{\mu\to 0} \frac{\tan \mu}{\mu}=f'(0)=\sec^2(0)=1^2=1$ .

Report an Error

Example Question #4 : Definition Of Derivative

Approximate the derivative if $y=\sqrt{x}$ where x=2 .

Possible Answers:

0.35359

0.35355

0.35351

0.35346

0.35353

Correct answer:

0.35355

Explanation:

Write the definition of the limit.

$\lim_{h\to 0}\frac{f(x+h)-f(x)}{h}$

Substitute x=2 .

$\lim_{h\to 0}\frac{f(2+h)-f(2)}{h}=\lim_{h\to 0}\frac{\sqrt{2+h}-\sqrt2}{h}$

Since is approaching to zero, it would be best to evaluate when we assume that is progressively decreasing. Let's assume h=0.1, 0.0001, 0.000001 and check the pattern.

$\frac{\sqrt{2+0.1}-\sqrt2}{0.1}=0.349241$

$\frac{\sqrt{2+0.0001}-\sqrt2}{0.0001}=0.353549$

$\frac{\sqrt{2+0.000001}-\sqrt2}{0.000001}=0.353553$

The best answer is: 0.35355

Report an Error

Example Question #5 : Definition Of Derivative

Given:

f(x)=3x^2(x^2-5x+2)^5

Find f'(x):

Possible Answers:

(15x^2)(x^2-5x+2)^4

(x^2-5x+2)^4(36x^3-45x^2+12x)

(x^2-5x+2)^4(60x^2-150)

(x^2-5x+2)^5(36x^3-105x^2+12x)

(x^2-5x+2)^4(36x^3-105x^2+12x)

Correct answer:

(x^2-5x+2)^4(36x^3-105x^2+12x)

Explanation:

Computation of the derivative requires the use of the Product Rule and Chain Rule.

The Product Rule is used in a scenario when one has two differentiable functions multiplied by each other:

f(x)*g(x)

$\frac{d}{dx}[f(x)*g(x)]=f(x)*g'(x)+g(x)*f(x)$

This can be easily stated in words as: "First times the derivative of the second, plus the second times the derivative of the first."

In the problem statement, we are given:

f(x)=3x^2(x^2-5x+2)^5

3x^2 is the "First" function, and (x^2-5x+2)^5 is the "Second" function.

The "Second" function requires use of the Chain Rule.

When:

$y=(f(x))^a \rightarrow \frac{dy}{dx}=a*(f(x))^{a-1}*f'(x)$

Applying these formulas results in:

f'(x)=(3x^2)[5(x^2-5x+2)^4(2x-5)]+(x^2-5x+2)^5(6x)

Simplifying the terms inside the brackets results in:

f'(x)=(3x^2)[(10x-25)(x^2-5x+2)^4]+(x^2-5x+2)^5(6x)

We notice that there is a common term that can be factored out in the sets of equations on either side of the "+" sign. Let's factor these out, and make the equation look "cleaner".

f'(x)=(x^2-5x+2)^4[(3x^2)(10x-25)+(6x)(x^2-5x+2)]

Inside the brackets, it is possible to clean up the terms into one expanded function. Let us do this:

f'(x)=(x^2-5x+2)^4[30x^3-75x^2+6x^3-30x^2+12x]

Simplifying this results in one of the answer choices:

f'(x)=(x^2-5x+2)^4(36x^3-105x^2+12x)

Report an Error

Example Question #4 : Definition Of Derivative

What is the value of the limit below?

$\lim_{x\to \frac{\pi}{6}}\frac{sin(x)-sin(\frac{\pi}{6})}{x-\frac{\pi}{6}}$

Possible Answers:

$\frac{\sqrt3}{2}$

$\frac{1}{2}$

Correct answer:

$\frac{\sqrt3}{2}$

Explanation:

Recall that one definition for the derivative of a function $f(x)\ at\ x=a$ is $f'(a)=\lim_{x\to a}\frac{f(x)-f(a)}{x-a}$ .

This means that this question is asking us to find the value of the derivative of sin(x) at $x=\frac{\pi}{6}$ .

Since

$\frac{d}{dx}sin(x)=cos(x)$ and $cos(\frac{\pi}{6})=\frac{\sqrt3}{2}$ , the value of the limit is $\frac{\sqrt3}{2}$ .

Report an Error

Example Question #3 : Derivatives

$f(x)=x^2\tan^{-1}(3x^5)$

$\frac{dy}{dx}=?$

Possible Answers:

$2x\tan^{-1}(3x^5)$

$\frac{15x^4\tan^{-1}(3x^5)}{1+9x^{10}}+2x^3$

$\frac{15x^6}{1+9x^{10}}+2x\tan^{-1}(3x^5)$

$\frac{15x^8}{1+9x^7}+2x\tan^{-1}(3x^5)$

$\frac{15x^4\tan^{-1}(3x^5)}{1+9x^{7}}+2x^3$

Correct answer:

$\frac{15x^6}{1+9x^{10}}+2x\tan^{-1}(3x^5)$

Explanation:

Evaluation of this integral requires use of the Product Rule. One must also need to recall the form of the derivative of $\tan^{-1}(x)$ .

Product Rule:

$\frac{d}{dx}[f(x)g(x)]=f(x)g'(x)+g(x)f'(x)$

$\frac{d}{dx}[\tan^{-1}(u)]=\frac{1}{1+u^2}\frac{du}{dx}$

Applying these two rules results in:

$\frac{dy}{dx}=\frac{d}{dx}[x^2\tan^{-1}(3x^5)]$

$\frac{dy}{dx}=(x^2)(\frac{1}{1+(3x^5)^2})(15x^4)+\tan^{-1}(3x^5)(2x)$

$\frac{dy}{dx}=\frac{15x^6}{1+9x^{10}}+2x\tan^{-1}(3x^5)$

This matches one of the answer choices.

Report an Error

Example Question #3 : Derivative Review

Use the definition of the derivative to solve for f'(x) .

f(x)=x^3

Possible Answers:

$\frac{1}{3}x^2$

3x^2

x^2

Correct answer:

3x^2

Explanation:

In order to find f'(x) , we need to remember how to find f'(x) by using the definition of derivative.

Definition of Derivative:

$f'(x)=\lim_{h\rightarrow0} \frac{f(x+h)-f(x)}{h}$

Now lets apply this to our problem.

$f'(x)=\lim_{h\rightarrow0}\frac{(x+h)^3-x^3}{h}$

Now lets expand the numerator.

$f'(x)=\lim_{h\rightarrow0}\frac{x^3+3x^2h+3xh^2+h^3-x^3}{h}$

We can simplify this to

$f'(x)=\lim_{h\rightarrow0}\frac{3x^2h+3xh^2+h^3}{h}$

Now factor out an h to get

$f'(x)=\lim_{h\rightarrow0}\frac{h(3x^2+3xh+h^2)}{h}$

We can simplify and then evaluate the limit.

$f'(x)=\lim_{h\rightarrow0} 3x^2+3xh+h^2$

$\\ \\ =3x^2+3x(0)+0^2 \\ =3x^2$

Report an Error

Example Question #5 : Definition Of Derivative

Use the definition of the derivative to solve for f'(x) .

f(x)=10x^2

Possible Answers:

20x

20x^2

Correct answer:

20x

Explanation:

In order to find f'(x) , we need to remember how to find f'(x) by using the definition of derivative.

Definition of Derivative:

$f'(x)=\lim_{h\rightarrow0} \frac{f(x+h)-f(x)}{h}$

Now lets apply this to our problem.

$f'(x)=\lim_{h\rightarrow0}\frac{10(x+h)^2-10x^2}{h}$

Now lets expand the numerator.

$f'(x)=\lim_{h\rightarrow0}\frac{10x^2+20xh+10h^2-10x^2}{h}$

We can simplify this to

$f'(x)=\lim_{h\rightarrow0}\frac{20xh+10h^2}{h}$

Now factor out an h to get

$f'(x)=\lim_{h\rightarrow0}\frac{h(20x+10h)}{h}$

We can simplify and then evaluate the limit.

$f'(x)=\lim_{h\rightarrow0} 20x+10h$

$\\ \\ =20x+10(0) \\ =20x$

Report an Error

View Calculus 2 Tutors

MEER
Certified Tutor

IIT , Master's/Graduate, Microelectronics .

View Calculus 2 Tutors

Muhammad
Certified Tutor

Quaid-E-Azam University, Master of Science, Applied Mathematics. Quaid-E-Azam University, Master of Philosophy, Applied Lingu...

View Calculus 2 Tutors

Vikash
Certified Tutor

national institute of technology Jamshedpur , Bachelor, Civil engineering .

All Calculus 2 Resources

9 Diagnostic Tests 308 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

French Tutors in Philadelphia, French Tutors in San Francisco-Bay Area, Math Tutors in Philadelphia, Reading Tutors in Miami, Reading Tutors in Los Angeles, French Tutors in San Diego, Physics Tutors in New York City, Statistics Tutors in Seattle, SSAT Tutors in Seattle, Physics Tutors in Denver

Popular Courses & Classes

GMAT Courses & Classes in Phoenix, ISEE Courses & Classes in Denver, SAT Courses & Classes in San Diego, Spanish Courses & Classes in Atlanta, LSAT Courses & Classes in Phoenix, LSAT Courses & Classes in Houston, ACT Courses & Classes in Chicago, ACT Courses & Classes in Atlanta, Spanish Courses & Classes in Miami, LSAT Courses & Classes in Atlanta

Popular Test Prep

ACT Test Prep in San Francisco-Bay Area, GRE Test Prep in Miami, SAT Test Prep in Houston, LSAT Test Prep in Chicago, MCAT Test Prep in Seattle, SAT Test Prep in Seattle, GRE Test Prep in Boston, MCAT Test Prep in Phoenix, ISEE Test Prep in Denver, GMAT Test Prep in Dallas Fort Worth

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Calculus 2 : Derivative Review

Study concepts, example questions & explanations for Calculus 2

All Calculus 2 Resources

Example Questions

Example Question #1 : Derivative Review

Example Question #2 : Derivative Review

Example Question #3 : Derivative Review

Example Question #4 : Definition Of Derivative

Example Question #4 : Definition Of Derivative

Example Question #5 : Definition Of Derivative

Given:

Find f'(x):

Example Question #4 : Definition Of Derivative

Example Question #3 : Derivatives

$f(x)=x^2\tan^{-1}(3x^5)$

Example Question #3 : Derivative Review

Example Question #5 : Definition Of Derivative

All Calculus 2 Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: