All Basic Geometry Resources
Example Questions
Example Question #1141 : Basic Geometry
A right isosceles triangle is stacked on top of a square as shown in the figure. Find the perimeter of the compound shape.


Notice that the hypotenuse of the right isosceles triangle is also the length of a side of the square.
First, we will need to find the length of the legs of the triangle by using the Pythagorean theorem.
Substitute in the given length of the hypotenuse to find the length of the leg of the triangle.
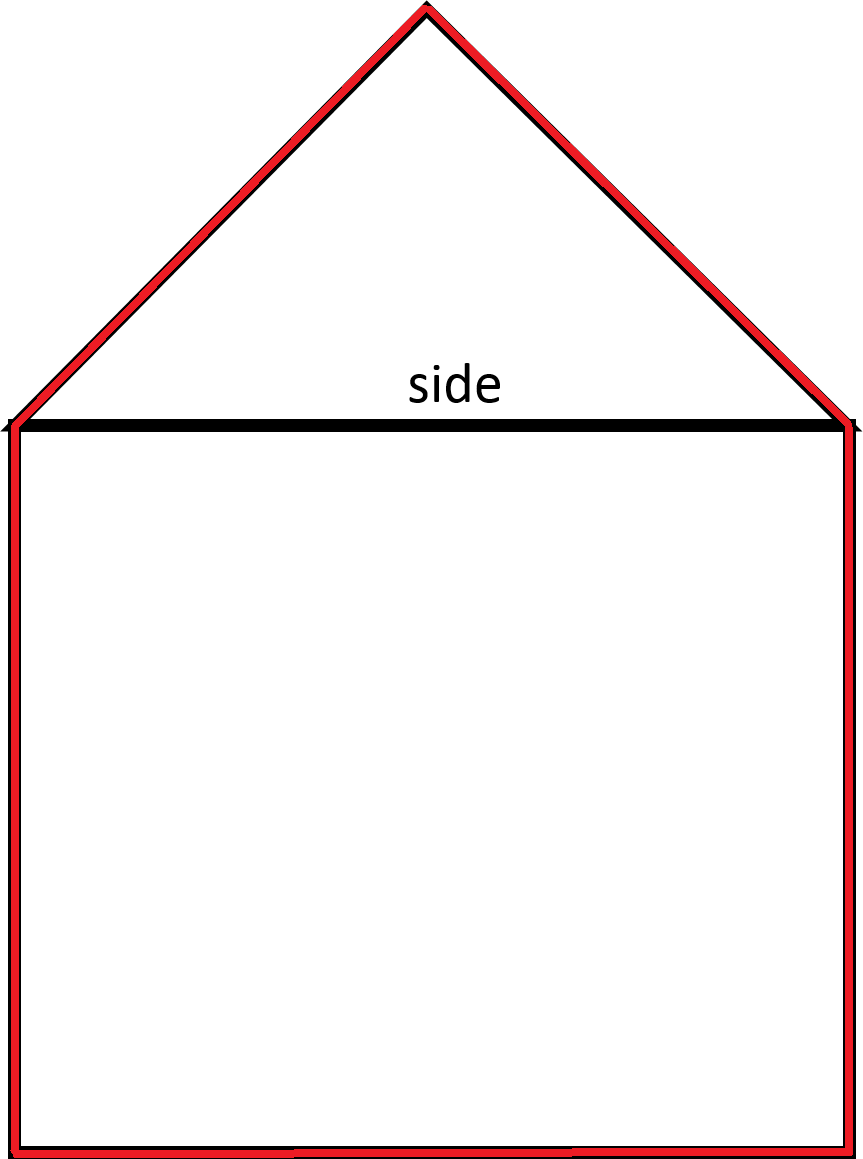
In order to find the perimeter, add up the lengths outlined in red. The perimeter includes the two legs of the triangle and three sides of the square.
Therefore:
Example Question #41 : How To Find The Perimeter Of A 45/45/90 Right Isosceles Triangle
A right isosceles triangle is stacked on top of a square as shown in the figure. Find the perimeter of the compound shape.


Notice that the hypotenuse of the right isosceles triangle is also the length of a side of the square.
First, we will need to find the length of the legs of the triangle by using the Pythagorean theorem.
Substitute in the given length of the hypotenuse to find the length of the leg of the triangle.

In order to find the perimeter, add up the lengths outlined in red. The perimeter includes the two legs of the triangle and three sides of the square.
Therefore:
Example Question #42 : How To Find The Perimeter Of A 45/45/90 Right Isosceles Triangle
A right isosceles triangle is stacked on top of a square as shown in the figure. Find the perimeter of the compound shape.


Notice that the hypotenuse of the right isosceles triangle is also the length of a side of the square.
First, we will need to find the length of the legs of the triangle by using the Pythagorean theorem.
Substitute in the given length of the hypotenuse to find the length of the leg of the triangle.

In order to find the perimeter, add up the lengths outlined in red. The perimeter includes the two legs of the triangle and three sides of the square.
Therefore:
Example Question #1 : How To Find The Length Of The Side Of A 45/45/90 Right Isosceles Triangle
The perimeter of a 45-45-90 triangle is 100 inches. To the nearest tenth of an inch, what is the length of each leg?
Let 

Therefore,
Example Question #1 : How To Find The Length Of The Side Of A 45/45/90 Right Isosceles Triangle

Example Question #3 : How To Find The Length Of The Side Of A 45/45/90 Right Isosceles Triangle

Example Question #161 : Triangles
Angle 


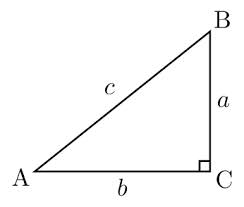
Since we know two of the three angles in this triangle, we can calculate the third, 
Therefore this is a 45/45/90 right triangle. Remember that 45/45/90 right triangles are have a leg:leg:hypotenuse ratio of 1:1:
We know the hypotenuse, 


To make this look like one of the answer choies, rationalize the denominator by muliplying the fraction by 
Example Question #1 : How To Find The Length Of The Side Of A 45/45/90 Right Isosceles Triangle



What is the length of 
There is not enough information given to answer this question.
We know that the sides of 



Divide the length of the hypotenuse by 
Multiply the length of the shorter sides by the ratio of magnification.
So the length of 


Example Question #162 : 45/45/90 Right Isosceles Triangles
The following image is not to scale.
Find the length of one of the legs of the right triangle.
Because of the tick marks on both legs, we can determine that this right triangle is a 45/45/90 triangle. Because the length of both legs are the same, this means that the angle opposite of each leg is also the same.
45/45/90 triangles are special, just like 30/60/90 triangles. Solving for one of the leg lengths can be determined easily through remembering the following:
Using this and the 7ft, we can solve for "s" which will provide us with the leg length.

Example Question #2 : How To Find The Length Of The Side Of A 45/45/90 Right Isosceles Triangle
If the hypotenuse of a right isosceles triangle is 
A right isosceles triangle is also a 
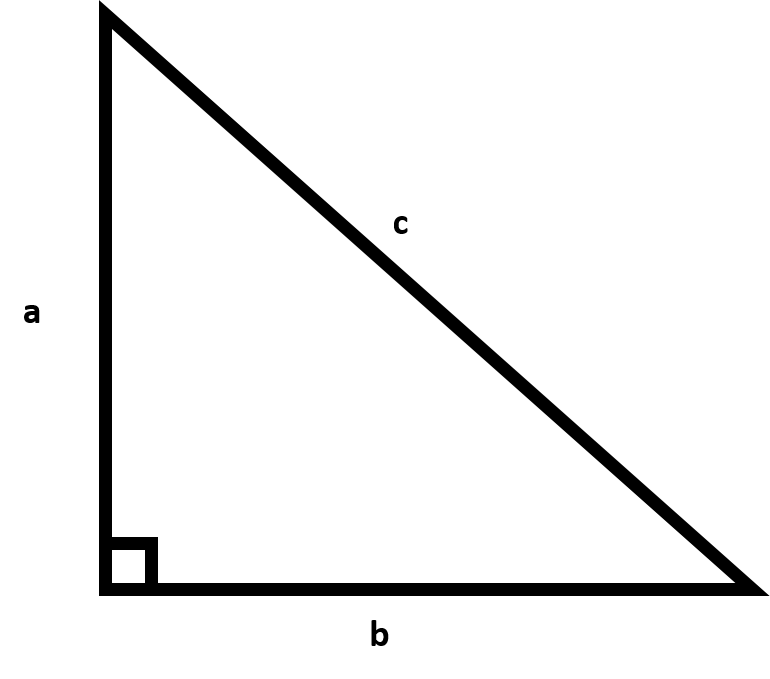
To find the length of a side, we will need to use the Pythagorean Theorem:
Since this is an isosceles triangle,
The Pythagorean Theorem can then be rewritten as the following:
Since we are trying to find the length of a side of this triangle, solve for 
Simplify.
Multiply the fraction by one in the form of 
Solve.
Now, substitute in the length of the hypotenuse in for 
Simplify.
Reduce.
All Basic Geometry Resources



































































































