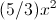Call Now to Set Up Tutoring:

(888) 888-0446

AP Calculus AB : Understanding the limiting process.

Study concepts, example questions & explanations for AP Calculus AB

Create An Account

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

← Previous 1 2 3 Next →

Example Question #1 : Understanding The Limiting Process.

Find the derivative.

y = sec (5x³)

Possible Answers:

y' = sec(5x³)tan(5x³)

y' = –csc(5x³)cot(5x³)(15x²)

y' = –sec(5x³)tan(5x³)(15x²)

y' = sec(5x³)tan(5x³)(15x²)

y' = –csc(5x³)cot(5x³)

Correct answer:

y' = sec(5x³)tan(5x³)(15x²)

Explanation:

The derivative of the function y = sec(x) is sec(x)tan(x). First take the derivative of the outside of the function: y = sec(4x³) : y' = sec(5x³)tan(5x³). Then take the derivative of the inside of the function: 5x³ becomes 15x². So your final answer is: y' = ec(5x³)tan(5x³)15x²

Report an Error

Example Question #1 : Understanding The Limiting Process.

Find the slope of the tangent line to the graph of f at x = 9, given that f(x) = –x²+ 5√(x)

Possible Answers:

–18

–18 – (5/6)

18 + (5/6)

–18 + (5/6)

Correct answer:

–18 + (5/6)

Explanation:

First find the derivative of the function.

f(x) = –x² + 5√(x)

f'(x) = –2x + 5(1/2)x^–1/2

Simplify the problem

f'(x) = –2x + (5/2x^1/2)

Plug in 9.

f'(3) = –2(9) + (5/2(9)^1/2)

= –18 + 5/(6)

Report an Error

Example Question #2 : Understanding The Limiting Process.

Find the derivative

(x + 1)/(x – 1)

Possible Answers:

(–2)/(x – 1)²

(–2)/(x – 1)

(x + 1) + (x – 1)

(–2)/(x + 1)²

Correct answer:

(–2)/(x – 1)²

Explanation:

Rewrite problem.

(x + 1)/(x – 1)

Use quotient rule to solve this derivative.

((x – 1)(1) – (x + 1)(1))/(x – 1)²

(x – 1) – x – 1)/(x – 1)²

–2/(x – 1)²

Report an Error

Example Question #174 : Functions, Graphs, And Limits

$\frac{d}{dx}sin^{-1} (3x)$

Possible Answers:

$\frac{3}{\sqrt{1-9 x^2}}$

$\frac{3}{\sqrt{1-3x^2}}$

$\frac{3x}{\sqrt{1-9 x^2}}$

$\frac{3x}{\sqrt{1- x^2}}$

Correct answer:

$\frac{3}{\sqrt{1-9 x^2}}$

Explanation:

Use the chain rule and the formula

$\frac{d}{dx}sin^{-1}u = \frac{1}{\sqrt{1-x^2}}$

Report an Error

Example Question #1 : Understanding The Limiting Process.

Find the derivative of

$f(x)=4(x^{3}-2x+11)(x-2)$

Possible Answers:

24x-2

$4x^{4}-8x^{3}-8x^{2}+60x-22$

$3x^{2}-8x+15$

$4(3x^{2}-2)$

$f'(x)=16x^{3}-24x^{2}-16x+60$

Correct answer:

$f'(x)=16x^{3}-24x^{2}-16x+60$

Explanation:

The answer is $f'(x)=16x^{3}-24x^{2}-16x+60$ . It is easy to solve if we multiply everything together first before taking the derivative.

$f(x)=4(x^{3}-2x+11)(x-2)$

$f(x)=4(x^{4}-2x^{3}+-2x^{2}+4x+11x-22)$

$f(x)=4x^{4}-8x^{3}-8x^{2}+60x-22$

$f'(x)=16x^{3}-24x^{2}-16x+60$

Report an Error

Example Question #173 : Functions, Graphs, And Limits

Differentiate f(x)=5x^3 .

Possible Answers:

(5/3)x^2

15x^4

15x^3

15x^2

8x^2

Correct answer:

15x^2

Explanation:

Using the power rule, multiply the coefficient by the power and subtract the power by 1.

f(x)=5x^3

$f'(x)=5*3(x^{3-1})$

f'(x)=15x^2

Report an Error

Example Question #177 : Functions, Graphs, And Limits

Differentiate $f(x)=\frac{1}{x^2}$ .

Possible Answers:

$\frac{-2}{x}$

$\frac{-2}{x^3}$

ln(x^2)

$\frac{1}{x^3}$

2ln(x^2)

Correct answer:

$\frac{-2}{x^3}$

Explanation:

Use the product rule:

$f(x)=\frac{1}{x^2}= x^{-2}$

$f'(x)=-2*1(x^{-2-1})= {-2}*{x^{-3}}= \frac{-2}{x^3}$

Report an Error

Example Question #3 : Understanding The Limiting Process.

Differentiate: f(x)=(5x-3)(2x+1)

Possible Answers:

20x-1

10x^2-x-3

20x+1

Correct answer:

20x-1

Explanation:

Use the product rule to find the derivative of the function.

f(x)=(5x-3)(2x+1)

f'(x)= (5x-3)*2+(2x+1)*5

f'(x)= 10x-6+10x+5=20x-1

Report an Error

Example Question #4 : Understanding The Limiting Process.

Differentiate: $y=e^{x^2}$

Possible Answers:

$2e^{x^2}$

2e^x

$2x^3e^{x^3}$

$2xe^{x^2}$

Correct answer:

$2xe^{x^2}$

Explanation:

The derivative of any function of e to any exponent is equal to the function multiplied by the derivative of the exponent.

$f'(x)=e^{x^2}*2x$

Report an Error

Example Question #3 : Understanding The Limiting Process.

Find the second derivative of $y=ln(\frac{x^2}{x^2+1})$ .

Possible Answers:

$\frac{-2(3x^2+1)}{(x^3+x)^2}$

$\frac{-2}{(x^3+x)^2}$

$\frac{2}{x^3+x}$

$\frac{2x}{x^4+x^2}$

Correct answer:

$\frac{-2(3x^2+1)}{(x^3+x)^2}$

Explanation:

y=ln(x^2)-ln(x^2+1)

$y'= \frac{1}{x^2}*2x-\frac{1}{x^2+1}*2x$

$\frac{2x}{x^2}-\frac{2x}{x^2+1}= \frac{2x^3+2x-2x^3}{x^2*(x^2+1)}= \frac{2x}{x^4+x^2}$

Factoring out an x gives you $\frac{2}{x^3+x}$ .

$y''=2(x^3+x)^{-1}=-2(x^3+x)^{-2}*(3x^2+1)=\frac{-2(3x^2+1)}{(x^3+x)^2}$

Report an Error

← Previous 1 2 3 Next →

View AP Calculus AB Tutors

Andre
Certified Tutor

University of North Florida, Bachelor of Science, Physics.

View AP Calculus AB Tutors

Rachel
Certified Tutor

University of Arkansas at Little Rock, Bachelor in Arts, Mathematics.

View AP Calculus AB Tutors

Yvan
Certified Tutor

Brigham Young University-Provo, Bachelor of Science, Mathematics Teacher Education.

All AP Calculus AB Resources

3 Diagnostic Tests 164 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

GRE Tutors in Denver, Spanish Tutors in Philadelphia, Math Tutors in Miami, ISEE Tutors in Atlanta, Statistics Tutors in Seattle, Statistics Tutors in Phoenix, Chemistry Tutors in Chicago, ACT Tutors in Philadelphia, Physics Tutors in Houston, LSAT Tutors in Philadelphia

Popular Courses & Classes

GMAT Courses & Classes in Philadelphia, ISEE Courses & Classes in Los Angeles, GRE Courses & Classes in Philadelphia, LSAT Courses & Classes in Miami, SAT Courses & Classes in Houston, Spanish Courses & Classes in Dallas Fort Worth, SAT Courses & Classes in San Francisco-Bay Area, ACT Courses & Classes in New York City, SAT Courses & Classes in Boston, ACT Courses & Classes in Los Angeles

Popular Test Prep

GMAT Test Prep in Philadelphia, GMAT Test Prep in San Francisco-Bay Area, SAT Test Prep in Chicago, LSAT Test Prep in Houston, ISEE Test Prep in Chicago, GRE Test Prep in Los Angeles, SAT Test Prep in Boston, SSAT Test Prep in Philadelphia, SSAT Test Prep in Chicago, MCAT Test Prep in Denver

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

AP Calculus AB : Understanding the limiting process.

Study concepts, example questions & explanations for AP Calculus AB

All AP Calculus AB Resources

Example Questions

Example Question #1 : Understanding The Limiting Process.

Example Question #1 : Understanding The Limiting Process.

Example Question #2 : Understanding The Limiting Process.

Example Question #174 : Functions, Graphs, And Limits

Example Question #1 : Understanding The Limiting Process.

Example Question #173 : Functions, Graphs, And Limits

Example Question #177 : Functions, Graphs, And Limits

Example Question #3 : Understanding The Limiting Process.

Example Question #4 : Understanding The Limiting Process.

Example Question #3 : Understanding The Limiting Process.

All AP Calculus AB Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: