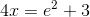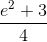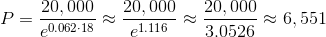All Algebra II Resources
Example Questions
Example Question #5 : Using E
Solve for
Step 1: Achieve same bases
Step 2: Drop bases and set exponents equal to eachother
Step 3: Solve for
Example Question #1 : Using E
Solve for
Step 1: Achieve same bases
Step 2: Drop bases, set exponenets equal to eachother
Step 3: Solve for
Example Question #1 : Exponential And Logarithmic Functions
Solve:
The answer does not exist.
To solve 

Since 


The answer is:
Example Question #8 : Using E
Simplify:
In order to eliminate the natural log on both side, we will need to raise both sides as a power with a base of 
The equation will become:
Subtract 
Simplify both sides.
Divide both sides by negative five.
The answer is:
Example Question #9 : Using E
Simplify:
In order to cancel the natural logs, we will need to use 
The equation becomes:
Subtract 
The equation becomes:
Use the quadratic equation to solve for the possible roots.
Simplify the quadratic equation.
The answers are:
Example Question #10 : Using E
Simplify:
In order to get rid of the natural log, we will need to use the exponential term 
The equation becomes:
Subtract nine from both sides.
Divide by three on both sides.
Simplify both sides.
The answer is:
Example Question #191 : Understanding Exponents
Simplify:
In order to solve for the x-variable, we will need to raise both sides as powers of base 

The equation becomes:
Add three on both sides.
Divide by four on both sides.
The equation is:
The answer is:
Example Question #192 : Understanding Exponents
On the day of a child's birth, a sum of money is to be invested into a certificate of deposit (CD) that draws 


If the amount of money invested is to be a multiple of 
If we let 


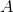
Substitute 

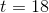

The minimum principal to be invested initially is $6,551. However, since we are looking for the multiple of $1,000 that guarantees a minimum final balance of $20,000, we round up to the nearest such multiple, which is $7,000 - the correct response.
Example Question #1 : Simple Exponents
Simplify the following expression
Example Question #2 : Simple Exponents
Simplify the following expression
Certified Tutor
Certified Tutor
All Algebra II Resources
































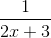






















![2x-3 -[2x]+(3)=x^2-[2x]+(3)](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/840260/gif.latex)