All Algebra 1 Resources
Example Questions
Example Question #51 : Functions And Lines
Example Question #11 : Algebraic Functions
An infinite sequence begins as follows:
Assuming this pattern continues infinitely, what is the sum of the first one hundred terms?
This can be best solved by looking at this sum as follows:
with 
Example Question #12 : Algebraic Functions
Define 

Example Question #12 : Algebraic Functions
Define 

What is 
Example Question #14 : How To Find F(X)
Define 

What is 
Example Question #61 : Functions And Lines
Each of the four tables below defines a relationship between 

One of these tables does not define a function. Identified the table.
Table 3
None of the above.
Table 4
Table 2
Table 1
Table 3
In table 3 we see an 


Example Question #16 : How To Find F(X)
Each of the following 4 sets defines a relationship between 

A =
B=
C =
D =
Set D
Set A
Set A and Set B
Set C
Set B
Set A
Only in set A one can see that there is an unique value of 



Example Question #17 : How To Find F(X)
Which of the following equations does not represent a function?
The correct answer is equation D. If we solve for 
The fact that each value of 


Example Question #3 : Functions
Which of the following equations represents a one-to-one function:
Only equation B maps each value of 



Example Question #14 : Algebraic Functions
Test whether the given function is symmetric with respect to the 

x axis
y axis
Not symmetric with respect to x axis, y axis, and the origin
All of the above
origin
Not symmetric with respect to x axis, y axis, and the origin
Since
It is not symmetric with respect the 
It is not symmetric with respect to the 
Hence multiplying by 
Hence it is not symmetric with respect to the origin.
Certified Tutor
Certified Tutor
All Algebra 1 Resources













































































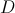



%5E%7B3%7D&space;-%5Cleft&space;(&space;-x&space;%5Cright&space;)%5E%7B2%7D&space;=&space;-x%5E%7B3%7D-x%5E%7B2%7D&space;%5Cneq&space;y)






