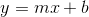All Algebra 1 Resources
Example Questions
Example Question #21 : How To Find The Equation Of A Perpendicular Line
Suppose a line has an x-intercept of one and a y-intercept of two. What is the equation of the perpendicular line which pass through the point 
Write the points that correspond to the x and y-intercepts given in the problem.
X-intercept of one:
Y-intercept of two:
Find the slope of this line connected with these points.
The slope is negative two. The slope of the perpendicular line is the negative reciprocal of this slope.
Write the slope-intercept form.
Substitute the perpendicular slope with the point that this line will pass through.
Solve for the y-intercept, 
Subtract nine halves on both sides.
Simplify both sides.
With the perpendicular slope and the y-intercept, write the equation of the line.
The answer is:
Example Question #91 : Perpendicular Lines
What is the slope of a line perpendicular to the line with the following equation?
Answer cannot be determined from this information.
Step 1: get the line into y = mx +b format to find the slope m:
Next, we need to remember that perpendicular lines have slopes that are negative reciprocals. Find the negative reciprocal of :
Example Question #1 : Slope And Line Equations
Given two points, (5, –8) (–2, 6), what is the equation of the line containing them both?
y = 2x – 2
y = –2x + 2
y = (–2/7)x + 8
No Solution
y = (2/7)x – 8
y = –2x + 2
First, you should plug the given points, (5, –8) (–2, 6), into the slope formula to find the slope of the line.
Then, plug the slope into the slope formula, y = mx + b, where m is the slope.
y = –2x + b
Plug in either one of the given points, (5, –8) or (–2, 6), into the equation to find the y-intercept (b).
6 = –2(–2) + b
6 = 4 + b
2 = b
Plug in both the slope and the y-intercept into slope intercept form.
y = –2x + 2
Example Question #1 : How To Find The Equation Of A Line
What is the equation of a line with slope of 3 and a y-intercept of –5?
y = 5x – 3
y = (3/5)x + 2
y = –5x + 3
y = 3x + 5
y = 3x – 5
y = 3x – 5
These lines are written in the form y = mx + b, where m is the slope and b is the y-intercept. We know from the question that our slope is 3 and our y-intercept is –5, so plugging these values in we get the equation of our line to be y = 3x – 5.
m = 3 and b = –5
Example Question #3 : How To Find The Equation Of A Line
A line contains the points (8, 3) and (-4, 9). What is the equation of the line?

Slope 


So 
At point (8, 3 ) the equation becomes
So
Example Question #1 : How To Find The Equation Of A Line
Given two points 





The slope of the line passing through points 

Now, the new line, since it is parallel, will have the same slope. To find the equation of this new line, we use point-slope form:



After rearranging, this becomes
Example Question #1 : How To Find The Equation Of A Line
Find the equation, in 


When finding the equation of a line from some of its points, it's easiest to first find the line's slope, or 
To find slope, divide the difference in 




Next, we just need to find 



Example Question #1 : How To Find The Equation Of A Line
We can find the equation of th line in slope-intercept form by finding 

First, calculate the slope, 
Next, using the slope and any point on the line, calculate the y-intercept, 
The correct equation in slope-intercept form is 
Example Question #2 : How To Find The Equation Of A Line
What is the equation of a line with a slope of 


None of the above
When a line is in the 







Example Question #8 : How To Find The Equation Of A Line
In 1990, the value of a share of stock in General Vortex was $27.17. In 2000, the value was $48.93. If the value of the stock rose at a generally linear rate between those two years, which of the following equations most closely models the price of the stock, 

We can treat the price of the stock as the 





To find the equation, first, we need the slope.
Now use the point-slope formula with this slope and either point (we will choose the second).
Certified Tutor
Certified Tutor
All Algebra 1 Resources