All Algebra 1 Resources
Example Questions
Example Question #16 : How To Find F(X)
Each of the following 4 sets defines a relationship between 

A =
B=
C =
D =
Set D
Set A
Set A and Set B
Set C
Set B
Set A
Only in set A one can see that there is an unique value of 



Example Question #14 : How To Find F(X)
Which of the following equations does not represent a function?
The correct answer is equation D. If we solve for 
The fact that each value of 


Example Question #1 : Functions
Which of the following equations represents a one-to-one function:
Only equation B maps each value of 



Example Question #3351 : Algebra 1
Test whether the given function is symmetric with respect to the 

Not symmetric with respect to x axis, y axis, and the origin
x axis
origin
All of the above
y axis
Not symmetric with respect to x axis, y axis, and the origin
Since
It is not symmetric with respect the 
It is not symmetric with respect to the 
Hence multiplying by 
Hence it is not symmetric with respect to the origin.
Example Question #21 : How To Find F(X)
A function is defined by the following set of ordered pairs:
What is its inverse?
The function does not have an inverse.
The function does not have an inverse.
The inverse of a function is the relation that switches the positions of the coordinates of each ordered pair. However, for a function to have an inverse, the result of those switches must itself be a function. The switches yield the relation
which is not a function, since the 

Example Question #71 : Functions And Lines
What is the inverse of the function defined by the following set of ordered pairs?
The function does not have an inverse.
The inverse of a function is the relation that switches the positions of the coordinates of each ordered pair. Therefore, the correct choice is

Example Question #72 : Functions And Lines
Find the inverse of this function.
No inverse exists
The inverse of an equation is given by solving for the x value in terms of y. To find the inverse, take the original equation, 
First multiply both sides by (x – 3).
Distribute y into the parenthesis.
Subtract xy to both sides.
Factor the x.
Divide both sides by (1 – y).
Once you have solved for x, switch the x and y terms.
Though an inverse function is found by solving for x, it still must follow the "y=" convention.
Example Question #73 : Functions And Lines
Given
Find 
Replacing or substituting 


When simplified we get
which is the correct answer.
Example Question #22 : How To Find F(X)
Given
Find 
which is equal to
Example Question #71 : Functions And Lines
Given 
Find 
Given 
Replacing 
one gets
Upon simplification one gets the correct answer which is
All Algebra 1 Resources























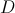


%5E%7B3%7D&space;-%5Cleft&space;(&space;-x&space;%5Cright&space;)%5E%7B2%7D&space;=&space;-x%5E%7B3%7D-x%5E%7B2%7D&space;%5Cneq&space;y)























































