The base is equal to 6.

The height of an quilateral triangle is equal to , where is the length of the base.

The equation used to find the area of a right triangle is:  where A is the area, b is the base, and h is the height of the triangle. In this question, we are given the height, so we need to figure out the base in order to find the area. Since we know both the height and hypotenuse of the triangle, the quickest way to finding the base is using the pythagorean theorem,  . a = the height, b = the base, and c = the hypotenuse.

Using the given information, we can write . Solving for b, we get  or . Now that we have both the base and height, we can solve the original equation for the area of the triangle. 

To find the area of a triangle use the formula:

, since the base is  and the height is , plugging in yields:

The easiest way to find the smallest possible integer sides is to simply factor the ratio we are given. In this case,  is already prime (since  is a prime number), so the smallest possible sides which hold to this triangle are  and . You may also recognize this number as a common Pythagorean triple.

The area of a triangle is expressed as , where  is the length and  is the height. Since our triangle is right, we know that two lines intersect at a  angle and thus serve well as our length and height. We also know that the longest side is always the hypotenuse, so the other two sides must be  and .

Applying our formula, we get:

Thus, the smallest possible area for our triangle is .

To find the area of a right triangle, find the lengths of the two perpendicular legs (since this gives us our length and height for the area formula).

In this case, we know that one angle is , and SOHCAHTOA tells us that , so:

 Substitute the angle measure and hypotenuse into the formula.

 Isolate the variable.

 Solve the left side (rounding to the nearest integer) using our Pythagorean formula:

--->  Substitute known values.

 --->  Simplify.

 Square root both sides.

So with our two legs solved for, we now only need to apply the area formula for triangles to get our answer:

So, the area of our triangle is .

To solve, simply use the formula for the area of a triangle given height h and base B.

Substitute 

 and  into the area formula.

Thus,

The area of a triangle is denoted by the equation 1/2 b x h.

b stands for the length of the base, and h stands for the height.

Here we are told that the perimeter (total length of all three sides) is 12, and the hypotenuse (the side that is neither the height nor the base) is 5 units long.

So, 12-5 = 7 for the total perimeter of the base and height.

7 does not divide cleanly by two, but it does break down into 3 and 4,

and 1/2 (3x4) yields 6.

Another way to solve this would be if you recall your rules for right triangles, one of the very basic ones is the 3,4,5 triangle, which is exactly what we have here

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

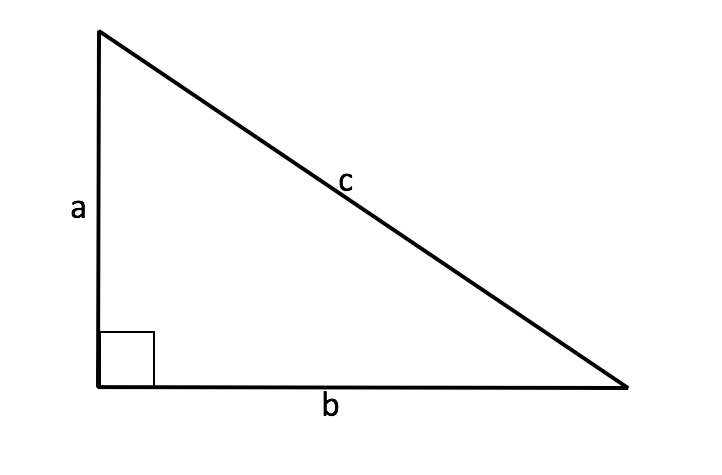
If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem.

First, we need to use the Pythagorean theorem to solve for .

Because we are dealing with a triangle, the only valid solution is  because we can't have negative values.

After you have found , plug it in to find the perimeter. Remember to simplify all square roots!

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem. The perimeter of a triangle is simply the sum of its three sides. Our problem is that we only know two of the sides.  The key for us is the fact that we have a right triangle (as indicated by the little box in the one angle).  Knowing two sides of a right triangle and needing the third is a classic case for using the Pythagorean theorem.  In simple (sort of), the Pythagorean theorem says that sum of the squares of the lengths of the legs of a right triangle is equal to the square of the length of its hypotenuse.

Every right triangle has three sides and a right angle.  The side across from the right angle (also the longest) is called the hypotenuse.  The other two sides are each called legs.  That means in our triangle, the side with length 17 is the hypotenuse, while the one with length 8 and the one we need to find are each legs.

What the Pythagorean theorem tells us is that if we square the lengths of our two legs and add those two numbers together, we get the same number as when we square the length of our hypotenuse.  Since we don't know the length of our second leg, we can identify it with the variable .

This allows us to create the following algebraic equation:

which simplified becomes

To solve this equation, we first need to get the variable by itself, which can be done by subtracting 64 from both sides, giving us

From here, we simply take the square root of both sides.

Technically,  would also be a square root of 225, but since a side of a triangle can only have a positive length, we'll stick with 15 as our answer.

But we aren't done yet.  We now know the length of our missing side, but we still need to add the three side lengths together to find the perimeter.

Our answer is 40.

How do you find the perimeter of a right triangle?

There are three primary methods used to find the perimeter of a right triangle.

1. When side lengths are given, add them together.
2. Solve for a missing side using the Pythagorean theorem.
3. If we know side-angle-side information, solve for the missing side using the Law of Cosines.

Method 1:

This method will show you how to calculate the perimeter of a triangle when all sides lengths are known. Consider the following figure:

If we know the lengths of sides , , and , then we can simply add them together to find the perimeter of the triangle. It is important to note several things. First, we need to make sure that all the units given match one another. Second, when all the side lengths are known, then the perimeter formula may be used on all types of triangles (e.g. right, acute, obtuse, equilateral, isosceles, and scalene). The perimeter formula is written formally in the following format:

Method 2:

In right triangles, we can calculate the perimeter of a triangle when we are provided only two sides. We can do this by using the Pythagorean theorem. Let's first discuss right triangles in a general sense. A right triangle is a triangle that has one  angle. It is a special triangle and needs to be labeled accordingly. The legs of the triangle form the  angle and they are labeled  and . The side of the triangle that is opposite of the  angle and connects the two legs is known as the hypotenuse. The hypotenuse is the longest side of the triangle and is labeled as .

If a triangle appears in this format, then we can use the Pythagorean theorem to solve for any missing side. This formula is written in the following manner:

We can rearrange it in a number of ways to solve for each of the sides of the triangle. Let's rearrange it to solve for the hypotenuse, .

Rearrange and take the square root of both sides. 

Simplify.

Now, let's use the Pythagorean theorem to solve for one of the legs, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

Last, let's use the Pythagorean theorem to solve for the adjacent leg, .

Subtract  from both sides of the equation.

Take the square root of both sides.

Simplify.

It is important to note that we can only use the following formulas to solve for the missing side of a right triangle when two other sides are known:

After we find the missing side, we can use the perimeter formula to calculate the triangle's perimeter.  

Method 3:

This method is the most complicated method and can only be used when we know two side lengths of a triangle as well as the measure of the angle that is between them. When we know side-angle-side (SAS) information, we can use the Law of Cosines to find the missing side. In order for this formula to accurately calculate the missing side we need to label the triangle in the following manner:

When the triangle is labeled in this way each side directly corresponds to the angle directly opposite of it. If we label our triangle carefully, then we can use the following formulas to find missing sides in any triangle given SAS information:

After, we calculate the right side of the equation, we need to take the square root of both sides in order to obtain the final side length of the missing side. Last, we need to use the perimeter formula to obtain the distance of the side lengths of the polygon.

Solution:

Now, that we have discussed the three methods used to calculate the perimeter of a triangle, we can use this information to solve the problem.

In order to calculate the perimeter we need to find the length of the hypotenuse using the Pythagorean theorem.

Rearrange.

Substitute in known values.

Now that we have found the missing side, we can substitute the values into the perimeter formula and solve.