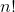All Statistics Resources
Example Questions
Example Question #1 : Probability
There are four different colored marbles in a jar—red, blue, white, and black—calculate how many outcomes are possible if a person picks two of the marbles if the order in which the marbles are picked matters.
Cannot be determined
Outcomes are not always easily identified or calculated; however, mathematical operations associated with permutations and combinations can make these processes easier. Permutations provide the number of outcomes when the order of events matter. Permutations are calculated using the following formula:
In this formula, the variable, 

Let's use this information to solve the problem. We know that this is a permutation because the order of the marbles matters. We need to assign numbers to each of the variables we have for items or marbles and we have two slots or bins that they are to be ordered into; therefore, we know the following:
Now, we need to calculate the number of permutations present in this model; however, we need to understand how to perform calculations involving factorials. Factorials are denoted with an exclamation point (!). For example, let's observe the following operation:
This denotes that for every non-negative integer, 

Let's use this information to solve our marble example.
Solve.
Simplify.
We know that there are twelve possible permutations.
Example Question #2 : Probability
What is the probability of drawing an ace from a standard deck of fifty-two cards?
In order to solve this standard, we need to understand how to calculate the probability of simple events. A probability is generally defined as the chances or likelihood of an event occurring. It is calculated by identifying two components: the event and the sample space. The event is defined as the favorable outcome or success that we wish to observe. On the other hand, the sample space is defined as the set of all possible outcomes for the event. Mathematically we calculate probabilities by dividing the event by the sample space:
Let's use this information to solve our problem. We want to know the probability of drawing an ace from a deck of cards. We know that the sample space is fifty-two because there are fifty two cards or outcomes in the deck. Also, we know that there are only four aces in a fair deck of cards; therefore,
Now, let's convert this into a percentage:
Probabilities expressed in fraction form will have values between zero and one. One indicates that an event will definitely occur, while zero indicates that an event will not occur. Likewise, probabilities expressed as percentages possess values between zero and one hundred percent where probabilities closer to zero are unlikely to occur and those close to one hundred percent are more likely to occur.
Example Question #3 : Probability
What is the probability of rolling "snake eyes"—a value of one on two separate dice—using two fair dice?
In order to solve this standard, we need to understand two primary components: probabilities and the property of independent events. A probability is generally defined as the chances or likelihood of an event occurring. It is calculated by identifying two components: the event and the sample space. The event is defined as the favorable outcome or success that we wish to observe. On the other hand, the sample space is defined as the set of all possible outcomes for the event. Mathematically we calculate probabilities by dividing the event by the sample space:
Let's use this information to solve our problem. First, let's calculate the probability of rolling a one. There is a single side of the die that has a value of one and there are six total sides on the die; therefore, we can write the following formula:
Now, let's find the probability of rolling a two on a separate die in the same roll.
Last, we need multiply these two probabilities together.
Certified Tutor
Certified Tutor
All Statistics Resources