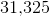All SSAT Upper Level Math Resources
Example Questions
Example Question #1 : Consecutive Integers
Three consecutive even integers have sum 924. What is the product of the least and greatest of the three?
No three consecutive even integers have sum 924.
Let the middle integer of the three be 

The three even integers are therefore 306, 308, and 310, and the product of the least and greatest of these is
Example Question #2 : Consecutive Integers
Three consecutive odd integers have sum 537. What is the product of the least and greatest of the three?
Let the middle integer of the three be 

The three integers are 177, 179, and 181, and the product of the least and greatest is
Example Question #1 : Sequences And Series
Four consecutive integers have sum 3,350. What is the product of the middle two?
Call the least of the four integers 

and they can be found using the equation
The integers are 836, 837, 838, 839.
To get the correct response, multiply:
Example Question #4 : Sequences And Series
Three consecutive integers have sum 
Let the middle integer of the three be 

This contradicts the condition that the numbers are integers. Therefore, three integers satisfying the given conditions cannot exist.
Example Question #3 : Consecutive Integers
Three consecutive integers have a sum of 
Let the middle integer of the three be 

The integers are therefore 103, 104, 105. The correct response is their product, which is
Example Question #6 : Consecutive Integers
What is the value of 
This is a geometric sequence since the pattern of the sequence is through multiplication.
You have to multiple each value by 
The value before 


Example Question #3 : Sequences And Series
Which of the following can be the sum of four consecutive positive integers?
None of the other answers are correct.
Let 



In other words, if 6 were to be subtracted from their sum, the difference would be a multiple of 4. Therefore, we subtract 6 from each of the choices and see if any of the resulting differences are multiples of 4.
Since this only happens in the case of 178, this is the only number of the four that can be a sum of four consecutive integers: 43, 44, 45, 46.
Example Question #1 : How To Find The Common Difference In Sequences
Set R consists of multiples of 4. Which of the following sets are also included within set R?
Set X, containing multiples of 2.
Set W, containing multiples of 8.
Set Z, containing multiples of 1.
Set Q, containing multiples of 7.
Set Y, containing multiples of 6.
Set W, containing multiples of 8.
The easiest way to solve this problem is to write out the first few numbers of the sets.
Set R (multiples of 4):
Set W (multiples of 8):
Set X (multiples of 2):
Set Y (multiples of 6):
Set Z (multiples of 1):
Set Q (multiples of 7):
Given that Set W is the only set in which the entire set of numbers is reflected in Set R, it is the correct answer.
Example Question #1 : How To Find The Common Difference In Sequences
What number comes next in this sequence?
4 12 9 6 18 15 12 36 33 __
Determining sequences can take some trial and error, but generally aren't as intimidating as they may at first appear. For this sequence, you multiply the first term by 3, and then subtract 3 two times in a row. Then repeat. When you get to 33, you have only subtracted 3 once, so you have to do that one more time:
Example Question #502 : Number Concepts And Operations
What number comes next in the sequence?

In order to find the next number in the sequence, take a look at the patterns and common differences between the existing numbers in the sequence. Starting with 




When we get to 




Certified Tutor
Certified Tutor
All SSAT Upper Level Math Resources