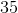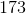Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : Right Triangles

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #91 : Right Triangles

A traffic light hangs t feet from the ground, over a street. A man standing the shadow of the traffic light is h feet tall, and his shadow is s feet long. How far is the man standing from the spot on the street directly under the traffic light?

Possible Answers:

$\frac{hs}{T}$

hsT

$\frac{Th}{s}$

$\frac{sT}{h}$

$\frac{h}{sT}$

Correct answer:

$\frac{sT}{h}$

Explanation:

We can set this problem up like a set of similar triangles.

The first triangle is created by the three points: The Traffic light, the spot beneath the traffic light, and the spot where the man is standing (which is also the spot where the traffic light's shadow is).

The height of this Triangle is "T" as given in the question, and its base is the part that we are asked to solve for.

The second triangle is created by the top of the man's head, his feet, and the end of his shadow.

The height of this Triangle is "h" as given in the questions, and the base is "s".

We set up a proportion:

$\frac{height}{base} = \frac {T}{X} = \frac{h}{s}$

where X is the distance we are asked to find. Simply cross-multiply to solve.

$X = \frac{sT}{h}$

Report an Error

Example Question #91 : Right Triangles

Screen shot 2016 02 12 at 8.29.40 am

An meteor crashed in the desert and created an oblong shaped crater. Scientists want to find the width of the crater as it is near their research facility. Line segments AC and DE intersect at B making the angles E and D the same. If AB is 2000 meters, BD is 1800 meters, DC is 600 meters and EB is 3600 meters, what is the width of the crater?

Possible Answers:

$1800\text{ m}$

$1200\text{ m}$

$1400\text{ m}$

$800\text{ m}$

$1600\text{ m}$

Correct answer:

$1200\text{ m}$

Explanation:

Screen shot 2016 02 12 at 8.29.40 am

To calculate the width of the crater, use the given information to establish that the image draws similar triangles. When triangles that have corresponding angles and a ratio to their side lengths they are considered to be similar triangles.

Identify the known information.

$\\ \angle E=\angle D \\\angle B=\angle B$

therefore,

$\angle A=\angle C$

and the bases of the triangles are parallel.

Also,

$\\\overline{AB}=1800 \text{ m} \\\overline{EB}=3600\text{ m} \\\overline{BD}=1800 \text{ m} \\\overline{CD}=600\text{ m}$

Set up the side ratios for this particular problem.

$\frac{AB}{BC}; \frac{AE}{CD}; \frac{EB}{BD}$

$\frac{1800}{BC}; \frac{x}{600}; \frac{3600}{1800}$

Looking at the only full ratio that is given, the scalar multiplier can be found.

$\frac{3600}{1800}=2$

Therefore, to find the width of the crater multiply $\overline{CD}$ by two.

$\\\frac{x}{600}=2 \\\\x=2\cdot 600 \\x=1200\text{ m}$

Report an Error

Example Question #4 : How To Find If Right Triangles Are Similar

Triangles

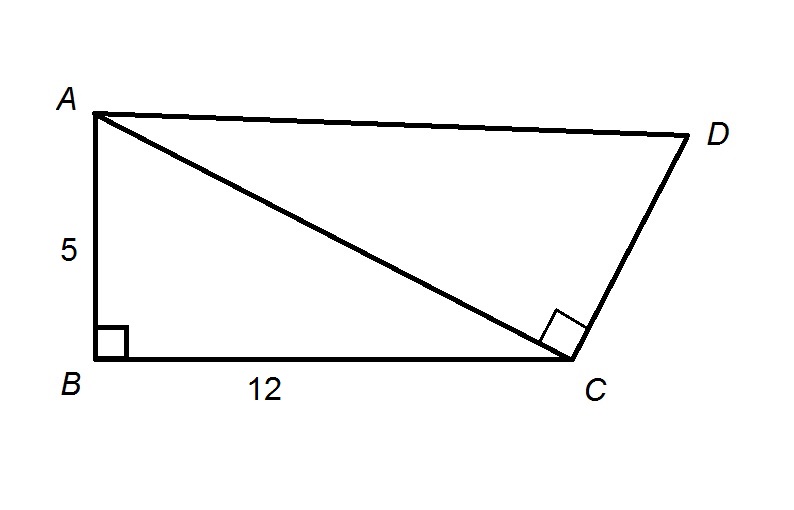
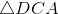
In the given diagram, $\bigtriangleup ABC \sim \bigtriangleup DCA$ . Give the area of $\bigtriangleup DCA$ to the nearest whole number.

Possible Answers:

173

Correct answer:

Explanation:

By the Pythagorean Theorem,

$(AC)^{2}= (AB)^{2} + (BC)^{2}$

Set AB = 5, BC= 13 , and solve for :

$(AC)^{2}= (AB)^{2} + (BC)^{2}$

$(AC)^{2}= 5^{2} + 12^{2}$

$(AC)^{2}= 25+144$

$(AC)^{2}=169$

Take the positive square root of both sides:

$AC = \sqrt{169} = 13$

$\bigtriangleup ABC \sim \bigtriangleup DCA$ , so corresponding sides are in proportion; specifically,

$\frac{DC}{AB} = \frac{AC}{CB}$

Set AB= 5, CB = 12, AC = 13 , and solve for :

$\frac{DC}{5} = \frac{13}{12}$

$\frac{DC}{5} \cdot 5 = \frac{13}{12} \cdot 5$

$DC = \frac{65}{12} = 5 \frac{5}{12}$

A right triangle has as its area half the product of the length of its legs, so the area of $\bigtriangleup DCA$ is

$\frac{1}{2} \cdot AC \cdot DC$

$= \frac{1}{2} \cdot 13 \cdot 5 \frac{5}{12}$

$= 35 \frac{5}{24}$

To the nearest whole number, this rounds to 35.

Report an Error

View SAT Mathematics Tutors

Joy
Certified Tutor

Boston University, Bachelor in Arts, Conservation Biology.

View SAT Mathematics Tutors

Sharif
Certified Tutor

NCCU, Bachelors, Physics and Mathematics.

View SAT Mathematics Tutors

Thomas
Certified Tutor

Michigan State University, Bachelor in Arts, History. Central Michigan University, Doctor of Philosophy, History.

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

Spanish Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in San Diego, LSAT Courses & Classes in Atlanta, MCAT Courses & Classes in New York City, Spanish Courses & Classes in Philadelphia, GRE Courses & Classes in Los Angeles, ISEE Courses & Classes in Washington DC, GMAT Courses & Classes in Miami, ACT Courses & Classes in New York City, ISEE Courses & Classes in San Diego

Popular Test Prep

MCAT Test Prep in Los Angeles, ISEE Test Prep in San Diego, ACT Test Prep in New York City, MCAT Test Prep in Dallas Fort Worth, LSAT Test Prep in Dallas Fort Worth, ISEE Test Prep in Phoenix, SSAT Test Prep in Chicago, ACT Test Prep in Miami, ISEE Test Prep in Los Angeles, MCAT Test Prep in Denver

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : Right Triangles

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #91 : Right Triangles

Example Question #91 : Right Triangles

Example Question #4 : How To Find If Right Triangles Are Similar

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: