SAT Math : SAT Mathematics
Study concepts, example questions & explanations for SAT Math
All SAT Math Resources
Example Questions
Example Question #24 : How To Find The Length Of The Hypotenuse Of A Right Triangle : Pythagorean Theorem
Given that two sides of a right triangle measure 2 feet and 3 feet, respectively, with a hypoteneuse of x, what is the perimeter of this right triangle (to the nearest tenth)?
8.6 feet
3.6 feet
6.4 feet
9.4 feet
18 feet
8.6 feet
Using the Pythagrean Theorem, we know that 
This tells us:
Taking the square root of both sides, we find that
To find the perimeter, we add the side lengths together, which gives us that the perimeter is:
Example Question #25 : How To Find The Length Of The Hypotenuse Of A Right Triangle : Pythagorean Theorem
Example Question #51 : Triangles
Kathy and Jill are travelling from their home to the same destination. Kathy travels due east and then after travelling 6 miles turns and travels 8 miles due north. Jill travels directly from her home to the destination. How miles does Jill travel?
Kathy's path traces the outline of a right triangle with legs of 6 and 8. By using the Pythagorean Theorem
Example Question #52 : Triangles
Example Question #26 : How To Find The Length Of The Hypotenuse Of A Right Triangle : Pythagorean Theorem
In order to get to work, Jeff leaves home and drives 4 miles due north, then 3 miles due east, followed by 6 miles due north and, finally, 7 miles due east. What is the straight line distance from Jeff’s work to his home?
15
11
6√2
2√5
10√2
10√2
Jeff drives a total of 10 miles north and 10 miles east. Using the Pythagorean theorem (a2+b2=c2), the direct route from Jeff’s home to his work can be calculated. 102+102=c2. 200=c2. √200=c. √100√2=c. 10√2=c
Example Question #61 : Triangles
Jim leaves his home and walks 10 minutes due west and 5 minutes due south. If Jim could walk a straight line from his current position back to his house, how far, in minutes, is Jim from home?
5√5
√5
6√6
√10
5√5
By using Pythagorean Theorem, we can solve for the distance “as the crow flies” from Jim to his home:
102 + 52 = x2
100 + 25 = x2
√125 = x, but we still need to factor the square root
√125 = √25*5, and since the √25 = 5, we can move that outside of the radical, so
5√5= x
Example Question #53 : Triangles
A square enclosure has a total area of 3,600 square feet. What is the length, in feet, of a diagonal across the field rounded to the nearest whole number?
75
95
85
100
60
85
In order to find the length of the diagonal accross a square, we must first find the lengths of the individual sides.
The area of a square is found by multiply the lengths of 2 sides of a square by itself.
So, the square root of 3,600 comes out to 60 ft.
The diagonal of a square can be found by treating it like a right triangle, and so, we can use the pythagorean theorem for a right triangle.
602 + 602 = C2
the square root of 7,200 is 84.8, which can be rounded to 85
Example Question #54 : Triangles
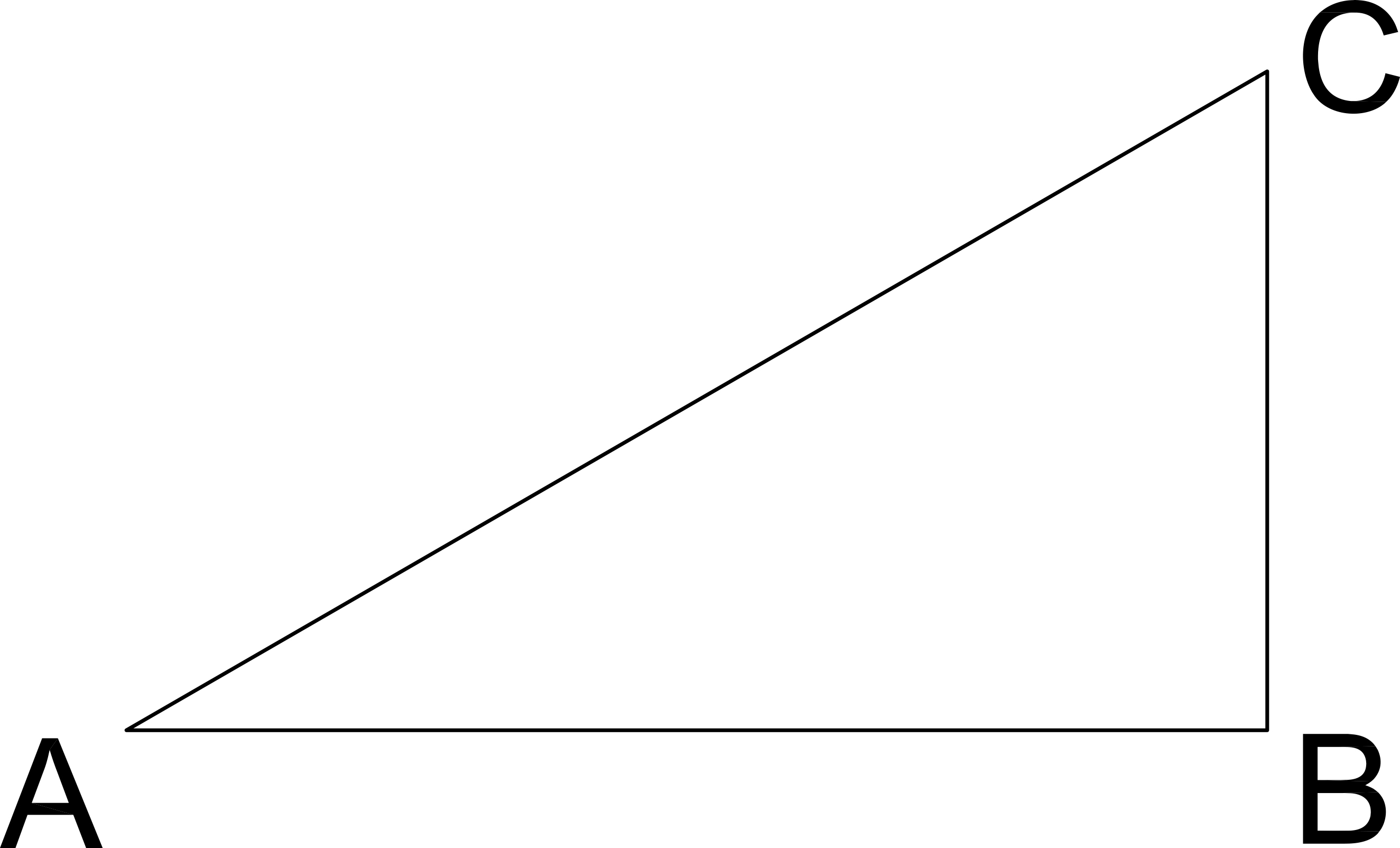
If the length of CB is 6 and the angle C measures 45º, what is the length of AC in the given right triangle?
6√2
6
9
12√2
72
6√2
Pythagorean Theorum
AB2 + BC2 = AC2
If C is 45º then A is 45º, therefore AB = BC
AB2 + BC2 = AC2
62 + 62 = AC2
2*62 = AC2
AC = √(2*62) = 6√2
Example Question #53 : Triangles
You leave on a road trip driving due North from Savannah, Georgia, at 8am. You drive for 5 hours at 60mph and then head due East for 2 hours at 50mph. After those 7 hours, how far are you Northeast from Savannah as the crow flies (in miles)?
Distance = hours * mph
North Distance = 5 hours * 60 mph = 300 miles
East Distance = 2 hours * 50 mph = 100 miles
Use Pythagorean Theorem to determine Northeast Distance
3002 + 1002 =NE2
90000 + 10000 = 100000 = NE2
NE = √100000
Example Question #54 : Triangles
A square garden has an area of 49 ft2. To the nearest foot, what is the diagonal distance across the garden?
11
7
9
10
8
10
Since the garden is square, the two sides are equal to the square root of the area, making each side 7 feet. Then, using the Pythagorean Theorem, set up the equation 72 + 72 = the length of the diagonal squared. The length of the diagonal is the square root of 98, which is closest to 10.
All SAT Math Resources
