To figure out how many x-intercepts there are, we need to set out equation equal to zero.

Factor an x out

Factor inside of the parenthesis.  

We can see there are 3 x-intercepts at .

If the equation 

were graphed, the result would be a line with a slope of 0.5 and a y-intercept of 1.2. 0.5 represents the amount Tonya pays per hour to work at a computer, because we know that the rate is $0.50, and C represents the total amount she pays. Because we must add 1.2 (or $1.20) to the total amount of hours multiplied by the hourly rate in order to get the total amount Tonya paid, we can assume there is another charge for renting a computer, in addition to the hourly rate, and that that charge is $1.20. Therefore, the y-intercept of the graph of the equation represents the initial cost of using a computer. 

If 11 people are taking both courses, this means 51-11 or 40 are taking kickboxing only and 25-11 or 14 are taking yoga only. The number of people taking at least one course, therefore, is 40 + 14 + 11 = 65. The 83 members minus the 65 that are taking courses leaves 18 who are not taking any courses.

Since we know that only 10 cows are for milk only we must subtract this number from the total amount of cows to get our answer: 40 – 10 = 30 cows. The cows that do both are still used for reproduction, so the correct answer is 30 cows.

Totalling the number in math there are 50 students on the rosters of all the math classes. With 35 total students this means that there are 15 students taking 2 math classes. For the language classes there are 40 students on the roster, showing that 5 students are taking 2 language classes. The maximum number of students taking two math classes and two language classes is only as great as the smallest number taking a double math or language class, which is 5 students (limited by the language doubles). 

The answer is 2. Taking away the 4 writing students, the 13 math-only students, and the remaining 31 English students, we have 2 students remaining.

You can construct a Venn diagran in which one circle represents art (A), the other represents gym (B), the region of overlap is designated (C), and the number of students not present in either circle is designated (S).

First, it is given that S=450.

50% of students take art and 50% take gym and the total number of students is 1250. 50% of 1250 is 625. Thus, A+C=625 and B+C=625.

Setting them equal to each other we get A+C=B+C.

Subtract C from both sides to get A=B; so the same number of students take art only and gym only.

Now 1250-450= A+B+C=800.

Since A=B we can use substitution to get 2A+C=800.

Finally you can solve the system of equations using the method of your choice (substitution or elimination) to solve the system with A+C=625 and 2A+C=800.

For substitution, we solve for C in the first equation to get C=625-A. Then we substitute this value into the second equation to get 2A+ (625-A)=800. Solve for A to get A=175, so B=175 also. Since A+B+C = 800, C=450.

means intersection and means union

U = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}

A = {1, 3, 5}

A' = {2, 4, 6, 7, 8, 9, 10}

B = {2, 4, 6}

B' = {1, 3, 5, 7, 8, 9, 10}

= {2, 4, 6}

= {1, 3, 5}

= {1, 2, 3, 4, 5, 6}

First, let's find the factors of 48, which will give us all of the elements in P. In order to find the factors of 48, list the pairs of numbers whose product is 48.

The pairs are as follows:

1 and 48; 2 and 24; 3 and 16; 4 and 12; 6 and 8

Therefore the factors of 48 are 1, 2, 3, 4, 6, 8, 12, 16, 24, and 48.

Now we can write P = {1, 2, 3, 4, 6, 8, 12, 16, 24, 48}.

Next, we need to find the factors of 90.

Again list the pairs:

1 and 90; 2 and 45; 3 and 30; 5 and 18; 6 and 15; 9 and 10

Then the factors of 90 are 1, 2, 3, 5, 6, 9, 10, 15, 18, 30, 45, and 90.

Thus, Q = {1, 2, 3, 5, 6, 9, 10, 15, 18, 30, 45, 90}.

Next find the factors of 56:

1 and 56; 2 and 28; 4 and 14; 7 and 8

Set R = {1, 2, 4, 7, 8, 14, 28, 56}

Now, we need to find set T, which is P U (Q ∩ R).

We have to start inside the parantheses with Q ∩ R. The intersection of two sets consists of all of the elements that the two sets have in common. The only elements that Q and R have in common are 1 and 2. 

Q ∩ R = {1, 2} 

Lastly, we must find P U (Q ∩ R).

The union of two sets consists of any element that is in either of the two sets. Thus, the union of P and Q ∩ R will consist of the elements that are either in P or in Q ∩ R. The following elements are in either P or Q ∩ R:

{1, 2, 3, 4, 6, 8, 12, 16, 24, 48}

Therefore, T = {1, 2, 3, 4, 6, 8, 12, 16, 24, 48}.

The problem asks us to determine which choice does NOT belong to T. The number 28 doesn't belong to T.

The answer is 28.

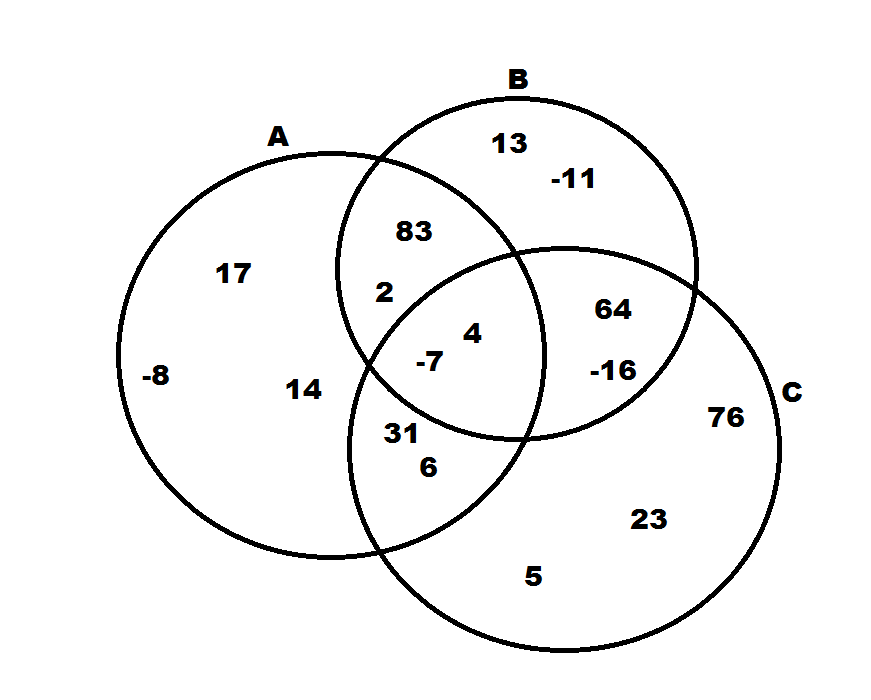
The symbol  stands for the union between two sets.  Therefore,  means the set of all numbers that are in either A or B.  Looking at our choices, the only number that isn't in either A, B, or both is 23.