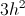Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : How to find the area of an acute / obtuse triangle

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #334 : Plane Geometry

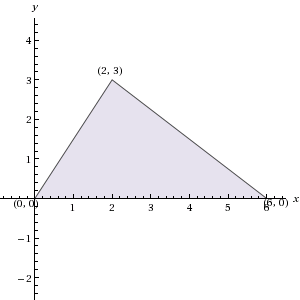
If triangle ABC has vertices (0, 0), (6, 0), and (2, 3) in the xy-plane, what is the area of ABC?

Possible Answers:

Correct answer:

Explanation:

Sketching ABC in the xy-plane, as pictured here, we see that it has base 6 and height 3. Since the formula for the area of a triangle is 1/2 * base * height, the area of ABC is 1/2 * 6 * 3 = 9.

Report an Error

Example Question #1 : How To Find The Area Of An Acute / Obtuse Triangle

The height, , of triangle PQR in the figure is one-fourth the length of . In terms of h, what is the area of triangle PQR ?

Possible Answers:

$3h^{2}$

$\frac{1}{2}h^{2}$

$h^{2}$

$2h^{2}$

Correct answer:

$2h^{2}$

Explanation:

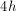
If $\dpi{100} \small h=\frac{1}{4}$ * $\dpi{100} \small \overline{PQ}$ , then the length of $\dpi{100} \small \overline{PQ}$ must be $\dpi{100} \small 4h$ .

Using the formula for the area of a triangle ( $\frac{1}{2}bh$ ), with $\dpi{100} \small b=4h$ , the area of the triangle must be $2h^{2}$ .

Report an Error

View SAT Mathematics Tutors

Quang
Certified Tutor

Georgetown University, Bachelor of Science, Finance.

View SAT Mathematics Tutors

Sarah
Certified Tutor

New York University, Bachelor of Fine Arts, Drama.

View SAT Mathematics Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

ACT Courses & Classes in Washington DC, LSAT Courses & Classes in Washington DC, MCAT Courses & Classes in San Diego, ISEE Courses & Classes in Washington DC, MCAT Courses & Classes in Washington DC, Spanish Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in Atlanta, ACT Courses & Classes in Miami, MCAT Courses & Classes in Phoenix, SSAT Courses & Classes in San Diego

Popular Test Prep

GRE Test Prep in Seattle, ACT Test Prep in Washington DC, SAT Test Prep in Washington DC, MCAT Test Prep in Chicago, LSAT Test Prep in Seattle, GMAT Test Prep in Phoenix, ISEE Test Prep in Washington DC, ACT Test Prep in Miami, ISEE Test Prep in Seattle, LSAT Test Prep in Atlanta

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : How to find the area of an acute / obtuse triangle

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #334 : Plane Geometry

Example Question #1 : How To Find The Area Of An Acute / Obtuse Triangle

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: