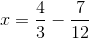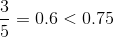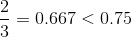Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : Operations with Fractions

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #5 : How To Divide Fractions

Evaluate the expression:

$\frac{3}{9}\div \frac{4}{15}$

Possible Answers:

$\frac{4}{45}$

$1\frac{1}{4}$

$1\frac{2}{7}$

$\frac{3}{5}$

$\frac{5}{2}$

Correct answer:

$1\frac{1}{4}$

Explanation:

When dividing fractions, you invert the second term and multiply the numbers.

$\frac{3}{9}\times\frac{15}{4}$

You can reduce the numbers that are diagonal from each other to make the numbers smaller and easier to multiply.

$\frac{3}{3}\times\frac{5}{4}=\frac{5}{4}=1\frac{1}{4}$

Report an Error

Example Question #4 : How To Divide Fractions

Simplify $\frac{\frac{13x}{5y}}{\frac{3x}{8y}}$

Possible Answers:

$\frac{104}{15}$

$\frac{18}{8}$

Fractions with variables cannot be divided

$\frac{16}{13}$

$\frac{39}{40}$

Correct answer:

$\frac{104}{15}$

Explanation:

When you dividing fractions, multiply by the reciprocal of the denominator.

$\frac{\frac{13x}{5y}}{\frac{3x}{8y}}=\frac{13x}{5y}*\frac{8y}{3x}=\frac{13*x*8*y}{5*y*3*x}=\frac{13*8}{5*3}=\frac{104}{15}$

Report an Error

Example Question #152 : Fractions

Which fraction has a value greater than that of X?

$x = \frac{4}{3}-\frac{7}{12}$

Possible Answers:

$\frac{7}{8}$

$\frac{5}{7}$

$\frac{3}{4}$

$\frac{2}{3}$

$\frac{3}{5}$

Correct answer:

$\frac{7}{8}$

Explanation:

First, start by putting changing the fractions in the equation so that they have the same denominator.

$x=\frac{4}{3}-\frac{7}{12}$

$x=\frac{16}{12}-\frac{7}{12}$

Then, you can easily subtract the numerators. The denominator stays the same at this point:

$x=\frac{9}{12}$

At this point, you can reduce the fraction by dividing the numerator and the denominator by :

$x=\frac{3}{4}$

Then, to easily compare the fraction to the answer choices, you can change the fraction to a decimal:

$\frac{3}{4}=0.75$

By changing the answer choices to decimals as well, you can easily figure out which value is greater than 0.75 :

$\frac{3}{5}=0.6<0.75$

$\frac{5}{7}=0.71<0.75$

$\frac{2}{3}=0.667<0.75$

$\frac{3}{4}=0.75=0.75$

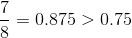
$\frac{7}{8}=0.875>0.75$ , so $\frac{7}{8}$ is the correct answer.

Report an Error

Example Question #1 : How To Add Fractions

Jesse has a large movie collection containing X movies. 1/3 of his movies are action movies, 3/5 of the remainder are comedies, and the rest are historical movies. How many historical movies does Jesse own?

Possible Answers:

(2/5)*X

(7/12)*X

(3/9)*X

(11/15)*X

(4/15)*X

Correct answer:

(4/15)*X

Explanation:

1/3 of the movies are action movies. 3/5 of 2/3 of the movies are comedies, or (3/5)*(2/3), or 6/15. Combining the comedies and the action movies (1/3 or 5/15), we get 11/15 of the movies being either action or comedy. Thus, 4/15 of the movies remain and all of them have to be historical.

Report an Error

Example Question #12 : Operations With Fractions

If x = 1/3 and y = 1/2, find the value of 2x + 3y.

Possible Answers:

5/6

6/5

13/6

Correct answer:

13/6

Explanation:

Substitute the values of x and y into the given expression:

2(1/3) + 3(1/2)

= 2/3 + 3/2

= 4/6 + 9/6

= 13/6

Report an Error

Example Question #131 : Arithmetic

Alternating1

Possible Answers:

$\dpi{100} -\frac{47}{60}$

$\dpi{100} \frac{47}{60}$

$\dpi{100} \frac{17}{60}$

$\dpi{100} \frac{43}{60}$

$\dpi{100} -\frac{43}{60}$

Correct answer:

$\dpi{100} \frac{47}{60}$

Explanation:

Alternating2

Alternating3

Report an Error

Example Question #11 : Operations With Fractions

What is the solution, reduced to its simplest form, for $x = \frac{7}{9}+\frac{3}{5}+\frac{2}{15}+\frac{7}{45}}$ ?

Possible Answers:

$x = \frac{75}{45}$

$x = \frac{7}{15}$

$x =2$

$x = \frac{115}{45}$

$x = \frac{5}{3}$

Correct answer:

$x = \frac{5}{3}$

Explanation:

$x=\frac{7}{9}+\frac{3}{5}+\frac{2}{15}+\frac{7}{45}=\frac{35}{45}+\frac{27}{45}+\frac{6}{45}+\frac{7}{45}=\frac{75}{45}=\frac{5}{3}$

Report an Error

Example Question #1 : Operations

What is the result of adding $20\%$ of $\frac{2}{7}$ to $\frac{1}{4}$ ?

Possible Answers:

$\frac{23}{11}$

$\frac{3}{39}$

$\frac{43}{140}$

$\frac{47}{140}$

$\frac{3}{28}$

Correct answer:

$\frac{43}{140}$

Explanation:

Let us first get our value for the percentage of the first fraction. 20% of 2/7 is found by multiplying 2/7 by 2/10 (or, simplified, 1/5): (2/7) * (1/5) = (2/35)

Our addition is therefore (2/35) + (1/4). There are no common factors, so the least common denominator will be 35 * 4 or 140. Multiply the numerator and denominator of 2/35 by 4/4 and the numerator of 1/4 by 35/35.

This yields:

(8/140) + (35/140) = 43/140, which cannot be reduced.

Report an Error

Example Question #12 : Operations With Fractions

Add:

$\frac{2}{9x}+\frac{4}{27}$

Possible Answers:

$\frac{2x+6}{27x}$

$\frac{4x+6}{27}$

$\frac{10}{27x}$

$\frac{6}{27x}$

$\frac{4x+6}{27x}$

Correct answer:

$\frac{4x+6}{27x}$

Explanation:

Find the least common denominator to solve this problem

Multiply 27 with , and multiply with 3 to obtain common denominators.

Convert the fractions.

$\frac{2}{9x}+\frac{4}{27}=\frac{6}{27x}+\frac{4x}{27x}$

Combine the terms as one fraction.

The answer is: $\frac{4x+6}{27x}$

Report an Error

Example Question #1 : How To Add Fractions

Solve $\frac{3}{7}+\frac{5}{8}-\frac{1}{2}$ .

Possible Answers:

$\frac{33}{56}$

$\frac{31}{56}$

$\frac{7}{8}$

$\frac{5}{7}$

Correct answer:

$\frac{31}{56}$

Explanation:

Finding the common denominator yields $\frac{24}{56}+\frac{35}{56}-\frac{28}{56}$ . We can then evaluate leaving $\frac{31}{56}$ .

Report an Error

View SAT Mathematics Tutors

Aaqib
Certified Tutor

The University of Texas at Dallas, Bachelor of Science, Neuroscience.

View SAT Mathematics Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

View SAT Mathematics Tutors

Zachary
Certified Tutor

Yale University, Bachelor of Science, Biology, General.

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

MCAT Courses & Classes in Houston, SSAT Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in Chicago, SSAT Courses & Classes in San Diego, GRE Courses & Classes in Houston, ACT Courses & Classes in Boston, SAT Courses & Classes in New York City, SSAT Courses & Classes in Miami, MCAT Courses & Classes in Philadelphia, ISEE Courses & Classes in Dallas Fort Worth

Popular Test Prep

ACT Test Prep in Boston, SSAT Test Prep in Philadelphia, SAT Test Prep in San Diego, LSAT Test Prep in Atlanta, ACT Test Prep in Philadelphia, MCAT Test Prep in San Diego, ISEE Test Prep in Boston, MCAT Test Prep in Houston, ISEE Test Prep in Seattle, GRE Test Prep in Houston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : Operations with Fractions

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #5 : How To Divide Fractions

Example Question #4 : How To Divide Fractions

Example Question #152 : Fractions

Example Question #1 : How To Add Fractions

Example Question #12 : Operations With Fractions

Example Question #131 : Arithmetic

Example Question #11 : Operations With Fractions

Example Question #1 : Operations

Example Question #12 : Operations With Fractions

Example Question #1 : How To Add Fractions

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: