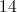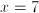All SAT Math Resources
Example Questions
Example Question #181 : Integers
Find the missing number in the following set:
The rule is as follows: add 2 to the previous number then multiply times 2.
The answer is (16 + 2) * 2 = 36.
Example Question #1 : Sets
You have the following data set:

Find the missing number 
In order to find the missing number (X), you must first identify the pattern in the set of numbers. Upon a glance, you can determine that the pattern starts with 4 and then the next number is 2n+1, with n being the preceding number in the set.
For example, let's start with 4. Multiplying 2*4+1 gives us 9, the next number in the set.
Therefore, the missing number in the set is 
Example Question #3 : How To Find The Missing Number In A Set

A sequence of boxes is constructed, the first six of which are seen above. What number is in the lower right corner of the ninth box?
The lower right number of each box is the sum of the other three numbers, which are found as follows:
The upper left numbers are simply the natural numbers in order;
The upper right numbers are the powers of 2, beginning with 
The lower left numbers are the terms of the Fibonacci sequence, which is formed by setting the first two terms equal to 1 and each subsequent term equal to its predecessors.
Therefore, the ninth box in the sequence includes the number 9 as its upper left entry, and 
The lower right entry is the sum of the other entries:
Example Question #1 : How To Find The Number Of Integers Between Two Other Integers
A custom-made ruler is 

There will be 15 gaps of 
Example Question #1 : Counting
Four consecutive odd integers sum to 40. How many of these numbers are prime?
Let x equal the smallest of the four numbers. Therefore:
Therefore the four odd numbers are 7, 9, 11, and 13. Since all are prime except 9, three of the numbers are prime.
Example Question #822 : Arithmetic
The positive integer 
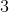
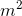





We know that the remainder, 




If 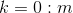

If 





If 




Thus, 
Example Question #1706 : Sat Mathematics
How many integers lie between 

Let's look at a small example, the numbers between 1 and 3 including all endpoints. We have 1, 2, and 3. If we subtract our numbers, we get 2. We need to add one more to get all the desired numbers. We can follow the same process with our larger numbers.
Take,

Then, adding 1,

Therefore, our answer is
Example Question #1 : How To Find The Number Of Integers Between Two Other Integers
Mrs. Lovell assigns her reading class to read pages three through sixty-four of their textbook. How many pages must each student read?
To count the number of pages in between these two page numbers, we want to subtract 


Therefore, our answer is
Example Question #3 : Counting
How many integers are between 

An important consideration whenever you're asked for the number of integers between two other numbers is to determine whether the two "end points" are included. Here the word "inclusive" tells us that we need to count both -8 and 17 in our calculation.
A helpful tip for these questions: if you're including both end points, you can calculate by using the range (greatest value minus smallest value) and adding 1 to account for the inclusive set. (If you need to prove that to yourself, just use "how many integers between 1 and 3 inclusive" - clearly the set is 1, 2, 3 for three integers, but 3-1 = 2, so you can see you need to add one more to get the answer).
For this question, that leaves us with 17 - (8) = 25 as the range, and if you add 1 to that for "inclusive" you get the correct answer, 26.
Another way to look at this one is to chop it up: between -8 and 17 there are 8 negative integers (-1 through -8), 17 positive integers (1 through 17), and 0 as one more integer. 8 + 17 + 1 = 26.
Example Question #2 : How To Find The Number Of Integers Between Two Other Integers
How many prime numbers are between 1 to 25?
A prime numbers is a number greater than 1 that can only be divided by 1 and itself. Simply count from 1 to 25 and see how many values fit the criteria.
2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20, 21, 22, 23, 24, 25
Prime numbers are underlined. Nine prime numbers are in this set interval.
Certified Tutor
All SAT Math Resources