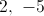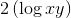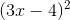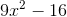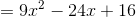SAT Math : Algebra
Study concepts, example questions & explanations for SAT Math
All SAT Math Resources
Example Questions
Example Question #571 : Algebra
Factor 2x2 - 5x – 12
(x + 4) (2x + 3)
(x + 4) (2x + 3)
(x - 4) (2x + 3)
(x – 4) (2x – 3)
(x - 4) (2x + 3)
Via the FOIL method, we can attest that x(2x) + x(3) + –4(2x) + –4(3) = 2x2 – 5x – 12.
Example Question #1 : How To Use Foil
x > 0.
Quantity A: (x+3)(x-5)(x)
Quantity B: (x-3)(x-1)(x+3)
The two quantities are equal
Quantity B is greater
Quantity A is greater
The relationship cannot be determined from the information given
Quantity B is greater
Use FOIL:
(x+3)(x-5)(x) = (x2 - 5x + 3x - 15)(x) = x3 - 5x2 + 3x2 - 15x = x3 - 2x2 - 15x for A.
(x-3)(x-1)(x+3) = (x-3)(x+3)(x-1) = (x2 + 3x - 3x - 9)(x-1) = (x2 - 9)(x-1)
(x2 - 9)(x-1) = x3 - x2 - 9x + 9 for B.
The difference between A and B:
(x3 - 2x2 - 15x) - (x3 - x2 - 9x + 9) = x3 - 2x2 - 15x - x3 + x2 + 9x - 9
= - x2 - 4x - 9. Since all of the terms are negative and x > 0:
A - B < 0.
Rearrange A - B < 0:
A < B
Example Question #572 : Algebra
Solve for all real values of 
First, move all terms to one side of the equation to set them equal to zero.
All terms contain an 
Now, we can factor the quadratic in parenthesis. We need two numbers that add to 
We now have three terms that multiply to equal zero. One of these terms must equal zero in order for the product to be zero.
Our answer will be 
Example Question #188 : New Sat
Find the product in terms of 
This question can be solved using the FOIL method. So the first terms are multiplied together:
This gives:
The x-squared is due to the x times x.
The outer terms are then multipled together and added to the value above.
The inner two terms are multipled together to give the next term of the expression.
Finally the last terms are multiplied together.
All of the above terms are added together to give:
Combining like terms gives
Example Question #4 : How To Use Foil
Expand the following expression:
Expand the following expression:
Let's begin by recalling the meaning of FOIL: First, Outer, Inner, Last.
This means that in a situation such as we are given here, we need to multiply all the terms in a particular way. FOIL makes it easy to remember to multiply each pair of terms.
Let's begin:
First:
Outer:
Inner:
Last:
Now, put it together in standard form to get:
Example Question #114 : Exponents
If 
43 = 64
Alternatively written, this is 4(4)(4) = 64 or 43 = 641.
Thus, m = 3 and n = 1.
m/n = 3/1 = 3.
Example Question #115 : Exponents
Write the following logarithm in expanded form:
Example Question #2353 : Sat Mathematics
If 
This question is asking you for the ratio of m to n. To figure it out, the easiest way is to figure out when 4 to an exponent equals 8 to an exponent. The easiest way to do that is to list the first few results of 4 to an exponent and 8 to an exponent and check to see if any match up, before resorting to more drastic means of finding a formula.
And, would you look at that. 
Example Question #2353 : Sat Mathematics
Simplify:
If you don't already have the pattern memorized, use FOIL. It's best to write out the parentheses twice (as below) to avoid mistakes:
Example Question #573 : Algebra
Simplify the radical.
We can break the square root down into 2 roots of 67 and 49. 49 is a perfect square and reduces to 7.
All SAT Math Resources
