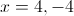We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

SAT II Math I : Solving Exponential Functions

Study concepts, example questions & explanations for SAT II Math I

Create An Account

All SAT II Math I Resources

6 Diagnostic Tests 113 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

← Previous 1 2 3 4 Next →

Example Question #1 : Solving Exponential Functions

Solve the following function:

$11=x^{2}-5$

Possible Answers:

and

Correct answer:

and

Explanation:

You must get by itself so you must add to both side which results in

$16=x^{2}$ .

You must get the square root of both side to undue the exponent.

This leaves you with x=4 .

But since you square the in the equation, the original value you plug can also be its negative value since squaring it will make it positive anyway.

This means your answer can be x=4 or x=-4 .

Report an Error

Example Question #1 : Solving And Graphing Exponential Equations

What is the horizontal asymptote of the graph of the equation $y = -2e^{x-3} -5$ ?

Possible Answers:

y=-2

$y=-\frac{5}{2}$

y=3

y=5

y=-5

Correct answer:

y=-5

Explanation:

The asymptote of this equation can be found by observing that $e^{x-3} > 0$ regardless of . We are thus solving for the value of as $e^{x-3}$ approaches zero.

$-2e^{x-3} < -2\cdot 0$

$-2e^{x-3} < 0$

$-2e^{x-3} -5 < 0-5$

$-2e^{x-3} -5 < -5$

y<-5

So the value that cannot exceed is , and the line y = -5 is the asymptote.

Report an Error

Example Question #2 : Solving And Graphing Exponential Equations

What is/are the asymptote(s) of the graph of the function

$f(x) = 5e^{x-2}-2$ ?

Possible Answers:

y = -2

y = -2, x=2

x=2

x=-2

y = -2, x=-2

Correct answer:

y = -2

Explanation:

An exponential equation of the form $f(x) = ae^{x-h} +k$ has only one asymptote - a horizontal one at y = k . In the given function, k = -2 , so its one and only asymptote is y = -2 .

Report an Error

Example Question #3 : Find The Equations Of Vertical Asymptotes Of Tangent, Cosecant, Secant, And Cotangent Functions

Find the vertical asymptote of the equation.

$y=\frac{x^2-9}{x^2-16}$

Possible Answers:

x=4, -4

x=1

x=0

x=4

There are no vertical asymptotes.

Correct answer:

x=4, -4

Explanation:

To find the vertical asymptotes, we set the denominator of the function equal to zero and solve.

0=x^2-16

16=x^2

$\sqrt{16}=\sqrt{x^2}$

4, -4=x

Report an Error

Example Question #3 : Solving And Graphing Exponential Equations

Consider the exponential function y=2^x+3 . Determine if there are any asymptotes and where they lie on the graph.

Possible Answers:

There are no asymptotes. goes to positive infinity in both the and directions.

There is one horizontal asymptote at y=3 .

There is one vertical asymptote at y=2 .

There is one vertical asymptote at y=3 .

Correct answer:

There is one horizontal asymptote at y=3 .

Explanation:

For positive values, increases exponentially in the direction and goes to positive infinity, so there is no asymptote on the positive -axis. For negative values, as decreases, the term 2^x becomes closer and closer to zero so approaches as we move along the negative axis. As the graph below shows, this is forms a horizontal asymptote.

Exp_asymp

Report an Error

Example Question #1 : Solving And Graphing Exponential Equations

Solve the equation for .

$\small 9^x=3^6$

Possible Answers:

$\small x=3$

$\small x=0$

$\small x=1$

$\small x=2$

Correct answer:

$\small x=3$

Explanation:

Begin by recognizing that both sides of the equation have a root term of .

$\small 9^x=3^6$

(3^2)^x=3^6

Using the power rule, we can set the exponents equal to each other.

$3^{(2*x)}=3^6$

$\small 2x=6$

$\small x=3$

Report an Error

Example Question #2 : Solving And Graphing Exponential Equations

Solve the equation for .

$\small 3^{2x}=81$

Possible Answers:

x=2

x=1

x=4

x=9

x=3

Correct answer:

x=2

Explanation:

Begin by recognizing that both sides of the equation have the same root term, .

$\small 3^{2x}=81$

$\small 3^{2x}=9^2$

$3^{2x}=(3^2)^2$

We can use the power rule to combine exponents.

$3^{2x}=3^4$

Set the exponents equal to each other.

2x=4

$\small x=2$

Report an Error

Example Question #3781 : Algebra Ii

In 2009, the population of fish in a pond was 1,034. In 2013, it was 1,711.

Write an exponential growth function of the form $y=ab^{x}}$ that could be used to model , the population of fish, in terms of , the number of years since 2009.

Possible Answers:

y=1.35(1.0011)^x

y=1711(1.3932)^x

y=1.2(1711)^x

y=1.1(1034)^x

y=1034(1.1342)^x

Correct answer:

y=1034(1.1342)^x

Explanation:

Solve for the values of a and b:

In 2009, y=1034 and x=0 (zero years since 2009). Plug this into the exponential equation form:

$1034=ab^{(0)}$ . Solve for to get .

In 2013, y=1711 and x=4 . Therefore,

1711=ab^4 or 1711=(1034)b^4 . Solve for to get

$b=\sqrt[4]{\frac{1711}{1034}}\approx1.1342$ .

Then the exponential growth function is

$y=1034(1.1342)^{x}$ .

Report an Error

Example Question #4 : Solving Exponential Equations

$4^{5x}=8^{4x-1}$

Solve for .

Possible Answers:

1.5

Correct answer:

1.5

Explanation:

8 and 4 are both powers of 2.

$4^{5x}=8^{4x-1}$

$2^{10x}=2^{12x-3}$

10x=12x-3 -2x=-3 x=1.5

Report an Error

Example Question #5 : Solving Exponential Equations

Solve for :

$80^{2x+3}=80^{5x-9}$

Possible Answers:

x=4

$x=\frac{1}{4}$

x=80

No solution

x=2

Correct answer:

x=4

Explanation:

Because both sides of the equation have the same base, set the terms equal to each other.

2x+3=5x-9

Add 9 to both sides: 2x+12=5x

Then, subtract 2x from both sides: 12=3x

Finally, divide both sides by 3: x=4

Report an Error

← Previous 1 2 3 4 Next →

View SAT Subject Test in Mathematics Level 1 Tutors

Amanda
Certified Tutor

Seattle University, Current Undergrad Student, Applied Mathematics.

View SAT Subject Test in Mathematics Level 1 Tutors

Matthew
Certified Tutor

Rider University, Bachelor of Science, Chemistry. Rutgers University-Camden, Master of Science, Organic Chemistry.

View SAT Subject Test in Mathematics Level 1 Tutors

Gregory
Certified Tutor

Pennsylvania State University-Main Campus, Bachelor of Science, Chemical Engineering. Carnegie Mellon University, Doctor of P...

All SAT II Math I Resources

6 Diagnostic Tests 113 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

SSAT Tutors in Washington DC, Math Tutors in New York City, Chemistry Tutors in Atlanta, Math Tutors in Houston, GRE Tutors in Philadelphia, ISEE Tutors in Miami, SAT Tutors in San Francisco-Bay Area, Chemistry Tutors in Dallas Fort Worth, Calculus Tutors in Houston, GRE Tutors in Chicago

Popular Courses & Classes

LSAT Courses & Classes in Miami, LSAT Courses & Classes in New York City, ACT Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in Denver, ISEE Courses & Classes in San Francisco-Bay Area, GRE Courses & Classes in Boston, SSAT Courses & Classes in Washington DC, SSAT Courses & Classes in Boston, Spanish Courses & Classes in Atlanta, Spanish Courses & Classes in Denver

Popular Test Prep

GMAT Test Prep in Miami, LSAT Test Prep in Dallas Fort Worth, SSAT Test Prep in Chicago, MCAT Test Prep in Los Angeles, ISEE Test Prep in Denver, SAT Test Prep in Denver, SSAT Test Prep in Denver, ISEE Test Prep in Houston, ACT Test Prep in Miami, SSAT Test Prep in Dallas Fort Worth

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT II Math I : Solving Exponential Functions

Study concepts, example questions & explanations for SAT II Math I

All SAT II Math I Resources

Example Questions

Example Question #1 : Solving Exponential Functions

Example Question #1 : Solving And Graphing Exponential Equations

Example Question #2 : Solving And Graphing Exponential Equations

Example Question #3 : Find The Equations Of Vertical Asymptotes Of Tangent, Cosecant, Secant, And Cotangent Functions

Example Question #3 : Solving And Graphing Exponential Equations

Example Question #1 : Solving And Graphing Exponential Equations

Example Question #2 : Solving And Graphing Exponential Equations

Example Question #3781 : Algebra Ii

Example Question #4 : Solving Exponential Equations

Example Question #5 : Solving Exponential Equations

All SAT II Math I Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: