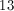Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : PSAT Mathematics

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1045 : Algebra

Worker $\dpi{100} \small A$ can make a trinket in 4 hours, Worker $\dpi{100} \small B$ can make a trinket in 2 hours. When they work together, how long will it take them to make a trinket?

Possible Answers:

$\dpi{100} \small 6\ hours$

$\dpi{100} \small \ 1 \frac{1}{3}\ hours$

$\dpi{100} \small \frac{1}{2}\ hour$

$\dpi{100} \small \ 1 \frac{1}{2}\ hours$

$\dpi{100} \small 3\ hours$

Correct answer:

$\dpi{100} \small \ 1 \frac{1}{3}\ hours$

Explanation:

The rates are what needs to be added. Rate $\dpi{100} \small A$ is $\dpi{100} \small \frac{1}{4}$ , or one trinket every 4 hours. Rate $\dpi{100} \small B$ is $\dpi{100} \small \frac{1}{2}$ , one per two hours.

$\dpi{100} \small \frac{1}{4}+ \frac{1}{2}=\frac{3}{4}$ , their combined rate in trinkets per hour.

Now invert the equation to get back to hours per trinket, which is what the question asks for: $\dpi{100} \small \frac{4}{3}\ or \ 1 \frac{1}{3}$

Report an Error

Example Question #1046 : Algebra

$a\ \mho \ b = a(b+1)-3$

Quantity A Quantity B

$1\ \mho\ 1$ $2\ \mho\ 0$

Possible Answers:

The relationship cannot be determined from the information given.

Quantity A is greater

Quantity A and Quantity B are equal

Quantity B is greater

Correct answer:

Quantity A and Quantity B are equal

Explanation:

Since $a\ \mho \ b = a(b+1)-3$ , then we have that

$1\ \mho \ 1=1(1+1)-3=-1$

and

$2\ \mho \ 0=2(0+1)-3=-1$ .

Thus, the two quantities are equal.

Report an Error

Example Question #1047 : Algebra

If the average of two numbers is $\dpi{100} \small 3y$ and one of the numbers is $\dpi{100} \small y+z$ , what is the other number, in terms of $\dpi{100} \small y$ and $\dpi{100} \small z$ ?

Possible Answers:

$\dpi{100} \small 3y+z$

$\dpi{100} \small 5y+z$

$\dpi{100} \small 4y-z$

$\dpi{100} \small 5y-z$

$\dpi{100} \small y+z$

Correct answer:

$\dpi{100} \small 5y-z$

Explanation:

The average is the sum of the terms divided by the number of terms. Here you have $\dpi{100} \small y+z$ and the other number which you can call $\dpi{100} \small x$ . The average of $\dpi{100} \small x$ and $\dpi{100} \small y+z$ is $\dpi{100} \small 3y$ . So $\dpi{100} \small 3y=\frac{(x+y+z)}{2}$

Multiply both sides by 2.

Solve for $\dpi{100} \small x=5y-z$ .

Report an Error

Example Question #1048 : Algebra

Alice is twice as old as Tom, but four years ago, she was three years older than Tom is now. How old is Tom now?

Possible Answers:

$\dpi{100} \small 7$

$\dpi{100} \small 9$

$\dpi{100} \small 21$

$\dpi{100} \small 13$

$\dpi{100} \small 3$

Correct answer:

$\dpi{100} \small 7$

Explanation:

The qustion can be broken into two equations with two unknows, Alice age $\dpi{100} \small (A)$ and Tom's age $\dpi{100} \small (T)$ .

$\dpi{100} \small A=2T$

$\dpi{100} \small A-4=T+3$

$\dpi{100} \small 2T-4=T+3$

$\dpi{100} \small T=7$

Report an Error

Example Question #1001 : Psat Mathematics

A jet goes from City 1 to City 2 at an average speed of 600 miles per hour, and returns along the same path at an average speed if 300 miles per hour. What is the average speed, in miles per hour, for the trip?

Possible Answers:

400miles/hour

500miles/hour

300miles/hour

350miles/hour

450miles/hour

Correct answer:

400miles/hour

Explanation:

Chose a number for the distance between City 1 and 2; 1800 works well, as it is a multiple of 600 and 300.

Now, find the time for each trip, the total distance, and the total time.

$t_1=\frac{d_1}{v_1}=\frac{1800}{600}=3$

$t_2=\frac{d_2}{v_2}=\frac{1800}{300}=6$

$total\ t=t_1+t_2=3+6=9$

$total\ d=d_1+d_2=1800+1800=3600$

Now we can find the average speed by dividing the total distance by the total time.

$average speed = \frac{total distance}{total time} = \frac{3600 miles}{9 hours} = 400 miles/hour$

Report an Error

Example Question #1002 : Psat Mathematics

$f(x)=3x^{2}-5$

g(x)=9-2x

Find f(g(5)) .

Possible Answers:

131

Correct answer:

Explanation:

Plug 5 into g(x) first:

g(5)=9-2(5)

=-1

Now, plug this answer into f(x) :

$f(-1)=3(-1)^{2}-5$

=3-5

=-2

Report an Error

Example Question #1003 : Psat Mathematics

If f(x) = 3x + 7 and g(x) = x^2 - 12 , what is f(g(x)) ?

Possible Answers:

9x^2 - 29

3x^2 + 29

3x^3 - 29

9x^3 + 29

3x^2 - 29

Correct answer:

3x^2 - 29

Explanation:

Plug g(x) into f(x) as if it is just a variable. This gives f(g(x)) = 3(x²– 12) + 7.

Distribute the 3: 3x²– 36 + 7 = 3x²– 29

Report an Error

Example Question #1004 : Psat Mathematics

f(x) = 4x + 17

Solve f(x) for the equation above for x = 3.

Possible Answers:

Correct answer:

Explanation:

The correct answer is 29. We plug in 3 into the equation above and solve for x. So we find that f(x) = 4(3) + 17. 12 + 17 = 29

Report an Error

Example Question #441 : Gre Quantitative Reasoning

For which value of are the following two functions equal?

$F(x)=2x+3^x+\frac{9x}{3}$

$G(x)=\frac{2^x+4^x}{2}-4x-5x+1$

Possible Answers:

Correct answer:

Explanation:

It is important to follow the order of operations for this equation and find a solution that satisfies both F(x) and G(x).

Recall the order of operations is PEMDAS: Parentheses, Exponents, Multiplication/Division, Addition/Subtraction.

The correct answer is 4 because

F(x) = 2x + 3^x+ (9x/3) = 2(4) + 3⁴ + ((9 * 4)/3) = 101, and

G(x) = (((2⁴ + 4⁴)/2) - 4 * 4) – 5(4) + 1 = 101.

Report an Error

Example Question #442 : Gre Quantitative Reasoning

The function is defined as f(x) = 3x^2 + 6x + 27 . What is f(-1) ?

Possible Answers:

-36

Correct answer:

Explanation:

Substitute -1 for in the given function.

$f(-1)=3(-1)^{2}+6(-1)+27$

f(-1)=3(1)-6+27

f(-1)=3-6+27

f(-1)=24

If you didn’t remember the negative sign, you will have calculated 36. If you remembered the negative sign at the very last step, you will have calculated -36; however, if you did not remember that (-1)^2 is 1, then you will have calculated 18.

Report an Error

View PSAT Mathematics Tutors

Keith
Certified Tutor

Xavier University, Bachelor in Business Administration, Accounting. Ohio University-Main Campus, Masters in Education, Mathem...

View PSAT Mathematics Tutors

Joy
Certified Tutor

Boston University, Bachelor in Arts, Conservation Biology.

View PSAT Mathematics Tutors

Nico
Certified Tutor

New York University, Bachelors, Philosophy.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Calculus Tutors in San Francisco-Bay Area, Reading Tutors in Boston, Computer Science Tutors in Boston, French Tutors in Houston, Spanish Tutors in New York City, Biology Tutors in Philadelphia, Computer Science Tutors in San Francisco-Bay Area, GMAT Tutors in Seattle, ACT Tutors in Boston, LSAT Tutors in San Francisco-Bay Area

Popular Courses & Classes

Spanish Courses & Classes in Los Angeles, SAT Courses & Classes in New York City, SSAT Courses & Classes in Dallas Fort Worth, SSAT Courses & Classes in Denver, GMAT Courses & Classes in Philadelphia, SAT Courses & Classes in Atlanta, ISEE Courses & Classes in Chicago, GRE Courses & Classes in Houston, Spanish Courses & Classes in New York City, GMAT Courses & Classes in Miami

Popular Test Prep

GMAT Test Prep in Boston, ISEE Test Prep in San Francisco-Bay Area, SAT Test Prep in San Diego, LSAT Test Prep in Miami, GRE Test Prep in San Francisco-Bay Area, MCAT Test Prep in Phoenix, GMAT Test Prep in Denver, GRE Test Prep in Atlanta, GRE Test Prep in Philadelphia, SSAT Test Prep in Boston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : PSAT Mathematics

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #1045 : Algebra

Example Question #1046 : Algebra

Example Question #1047 : Algebra

Example Question #1048 : Algebra

Example Question #1001 : Psat Mathematics

Example Question #1002 : Psat Mathematics

Example Question #1003 : Psat Mathematics

Example Question #1004 : Psat Mathematics

Example Question #441 : Gre Quantitative Reasoning

Example Question #442 : Gre Quantitative Reasoning

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: