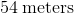PSAT Math : Quadrilaterals
Study concepts, example questions & explanations for PSAT Math
All PSAT Math Resources
Example Questions
Example Question #3 : Rectangles
Note: Figure NOT drawn to scale.
In the above figure,
Give the area of 
Insufficient information is given to determine the area.
Corresponding sidelengths of similar polygons are in proportion, so
We can use the Pythagorean Theorem to find 
The area of 
Example Question #5 : Rectangles
Note: Figure NOT drawn to scale.
In the above figure,
Give the area of Polygon 
Polygon 
The area of 
Now we find the area of 
The similarity of 
so
The area of 
Now add: 
Example Question #1 : How To Find If Rectangles Are Similar
Note: Figure NOT drawn to scale.
Refer to the above figure.
What percent of 
Insufficient information is given to answer the problem.
or
Therefore, 
Example Question #1 : How To Find If Rectangles Are Similar
Note: figure NOT drawn to scale.
Refer to the above figure.
Give the area of 
.
Set
Example Question #201 : Geometry
A rectangle has a width of 2x. If the length is five more than 150% of the width, what is the perimeter of the rectangle?
6x2 + 10x
5x + 10
5x + 5
10(x + 1)
6x2 + 5
10(x + 1)
Given that w = 2x and l = 1.5w + 5, a substitution will show that l = 1.5(2x) + 5 = 3x + 5.
P = 2w + 2l = 2(2x) + 2(3x + 5) = 4x + 6x + 10 = 10x + 10 = 10(x + 1)
Example Question #11 : Rectangles
Note: Figure NOT drawn to scale
Refer to the above figure, which shows a rectangular garden (in green) surrounded by a dirt path (in orange). The dirt path is seven feet wide throughout. Which of the following polynomials gives the perimeter of the garden in feet?
The length of the garden, in feet, is 
The width of the garden, in feet, is 
The perimeter, in feet, is twice the sum of the two:
Example Question #11 : Rectangles
Note: Figure NOT drawn to scale
Refer to the above figure, which shows a square garden (in green) surrounded by a dirt path (in orange) eight feet wide throughout. What is the perimeter of the garden?
The inner square, which represents the garden, has sidelength 
Example Question #11 : Rectangles
Farmer Dave has a rectangular field that is 50 yards wide and 40 yards long. He wants to enclose the field with a wire fence. How much wire does Farmer Dave need?
180 yards
200 yards
170 yards
160 yards
210 yards
180 yards
To solve this problem, find the perimeter of the rectangle. There are two sides that each measure 50 yards and two sides that each measure 40 yards. Together these four sides measure 180 yards.
Example Question #3 : How To Find The Perimeter Of A Figure
A rectangular garden has an area of 
We define the variables as 
We substitute these values into the equation for the area of a rectangle and get 
Lengths cannot be negative, so the only correct answer is 
Therefore, 
Example Question #1 : How To Find The Area Of A Rectangle
A contractor is going to re-tile a rectangular section of the kitchen floor. If the floor is 6ft x 3ft, and he is going to use square tiles with a side of 9in. How many tiles will be needed?
24
2
40
32
32
We have to be careful of our units. The floor is given in feet and the tile in inches. Since the floor is 6ft x 3ft. we can say it is 72in x 36in, because 12 inches equals 1 foot. If the tiles are 9in x 9in we can fit 8 tiles along the length and 4 tiles along the width. To find the total number of tiles we multiply 8 x 4 = 32. Alternately we could find the area of the floor (72 x 36, and divide by the area of the tile 9 x 9)
Certified Tutor
All PSAT Math Resources
