All PSAT Math Resources
Example Questions
Example Question #3 : How To Find The Part From The Whole With Percentage
Becky and Jason are running for class president, and each of the 30 students in the class voted. Becky received 60% of the votes. How many students voted for Jason?
18
12
21
15
12
If Becky received 60% of the vote, Jason must have received 40% of the vote. 40% is equal to 0.40. By multiplying the number of votes times the percentage, we can calculate how many votes Jason received.
0.40 * 30 = 12.
Example Question #1071 : Sat Mathematics
I ate 30% of an entire pizza. My friend Sally ate 20% of the remaining pizza. What percentage of the original pizza is left?
50%
44%
70%
56%
14%
56%
After I eat 30% of the pizza, 70%, or 0.7, is left. Sally eats 20%, or 0.2 of that. So to compute how much of the original pizza Sally has eaten, we must multiply 0.7 by 0.2. We will arrive with 0.14 or 14%. Thus we subtract my the 30% of the pizza I ate and the 14% of the pizza Sally ate from 100% or the original whole, and we will arrive with 56%.
Example Question #4 : How To Find The Part From The Whole With Percentage
The pie chart illustrates how Carla allocates her money each week.
If she spends $200 on groceries each week, how much does she spend on rent?
1000
750
450
500
350
350
1. 20% of Carla's whole budget is equal to $200. With this information, one can find Carla's weekly budget.
Set up the equation: 0.2b = 200 (b = budget).
b = 200/0.2 = 1000 = Carla's weekly budget
2. To find the amount Carla spends for rent, one needs to find what 35% of $1000 is.
0.35 x 1000 = 350
3. Because Carla spends 35% of her total budget on rent, she spends $350 on rent.
Example Question #5 : How To Find The Part From The Whole With Percentage
There are 



If p is the number of pounds of cargo that is initially on the ship and m is the number of pounds of cargo that we transfer (or remove from the ship), we can find how many pounds of cargo are still on the ship after the transfer by the expression p – m. In order to model this as a percent, we would have to form a fraction, using the initial pounds of cargo, p, as the whole amount and p – m as the fractional part.
So,
we would get 
However, this problem asks us to find the percent, so we would simply multiply by 100
![\dpi{100} \small \left [\frac{100\left (p-m \right )}{p} \right ]\%](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/18571/gif.latex)
Example Question #6 : How To Find The Part From The Whole With Percentage
Three salesmen, Gor, Levon, and Raffi, competed to sell the highest number of cars in the month of August. A total of 250 cars were sold.
Gor sold 100 cars. Levon sold 62% of the remaining cars, and Raffi sold the rest.
How many cars did Raffi sell?
39
93
77
75
57
57
We first subtract the 100 cars that Gor sold from the total of 250 sold. We are left with 150 cars, and we know that Levon sold 62% of them. 100% – 62% = 38%. Hence, Raffi sold 38% of 150 cars. 150 * 0.38 = 57
Example Question #2041 : Psat Mathematics
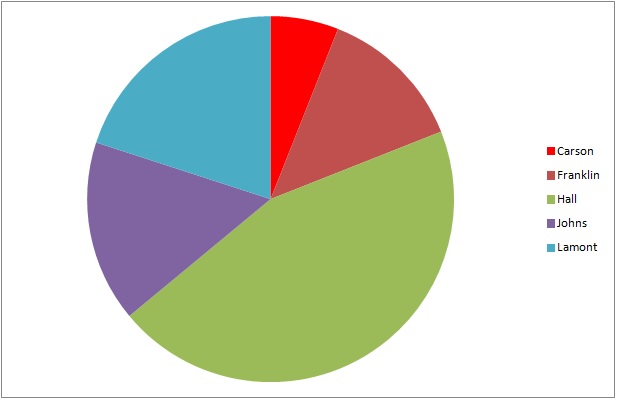
The above graph represents the results of a general election for mayor of Washington City. Hall is a Republican; Johns is a Democrat; the other three are independents.
5,328 people voted for independent candidates. Which is the best estimate for the number of people who voted for Hall?
The green wedge, which represents Hall, is about 45% of the circle. The three wedges representing independents Carson, Franklin, and Lamont - dark red, bright red, and turquoise, respectively - together comprise about 40% of the circle. We can set up, and solve for the unknown in, a proportion statement as follows:
The best estimate of the five is 6,000.
Example Question #482 : Arithmetic

The above graph represents the results of a general election for two seats on the city council of Lincoln City. Frankllin and Hall are Democrats; Johns and Lamont are Republicans; Carson is an independent.
6,219 people voted for Republicans. Which of the following is the best estimate for the number of people who voted for Democrats?
The two wedges that represent the Democratic candidates - green (Hall) and dark red (Franklin) - together make up about 60% of the circle. The two wedges that represent the Republican candidates - purple (Johns) and blue (Lamont) - make up about 35% of the circle. We can estimate the number of people who voted for a Democrat using a proportion statement:
10,660 is the best estimate.
Example Question #11 : Whole And Part
A school is made up of 400 students. Each student must belong to one of the four clubs shown in the chart below. How many students are in chorus?

You must first determine the percentage of the students in chorus. To do this, subtract the other percentages from 100: 100 – (35 + 29 + 24) = 12.
Therefore, 12% of the 400 students at this school attend chorus.
To determine the number of students in the chorus, multiply .12 (12%) by 400.
0.12(400) = 48
Certified Tutor
Certified Tutor
All PSAT Math Resources

![\dpi{100} \small \left [\frac{p}{100\left (p-m \right )} \right ]\%](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/135/gif.latex)
![\dpi{100} \small \left [\frac{p-m}{100} \right ]\%](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/136/gif.latex)
![\dpi{100} \small \left [\frac{100m}{p} \right ]\%](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/137/gif.latex)
![\dpi{100} \small \left [\frac{100\left (p-m \right )}{p} \right ]\%](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/134/gif.latex)
![\dpi{100} \small \left [\frac{100p}{m} \right ]\%](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/138/gif.latex)
























