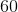PSAT Math : How to find the greatest common factor
Study concepts, example questions & explanations for PSAT Math
All PSAT Math Resources
Example Questions
Example Question #71 : Integers
If 
Since v is divisible by 2, 3 and 15, v must be a multiple of 30. Any number that is divisible by both 2 and 15 must be divisible by their product, 30, since this is the least common multiple.
Out of all the answer choices, v + 30 is the only one that equals a multiple of 30.
Example Question #1 : Factors / Multiples
Suppose that 
We are given information that m/4 is 10 greater than m/3. We set up an equation where m/4 = m/3 + 10.
We must then give the m variables a common denominator in order to solve for m. Since 3 * 4 = 12, we can use 12 as our denominator for both m variables.
m/4 = m/3 + 10 (Multiply m/4 by 3 in the numerator and denominator.)
3m/12 = m/3 + 10 (Multiply m/3 by 4 in the numerator and denominator.)
3m/12 = 4m/12 + 10 (Subtract 4m/12 on both sides.)
-m/12 = 10 (Multiply both sides by -12.)
m = -120
-120/4 = -30 and -120/3 = -40. -30 is 10 greater than -40.
Example Question #4 : Factors / Multiples
Which of the following is a possible value of 
We need to find ways to factor 18 such that the three factors are different, and then find the sum of those factors in each case.
18 can be factored as the product of three integers in four ways:
I)
II)
III)
IV)
Disregard I and IV since each repeats a factor.
In (II), the sum of the factors is 
Example Question #1 : Factors / Multiples
Which of the following cannot be equal to 
We look for ways to write 45 as the product of three integers, then we find the sum of the integers in each situation. They are:
Sum:
Sum:
Sum:
Sum:
Of the five choices, only 33 is not a possible sum of the factors. This is the correct choice.
Example Question #7 : Factors / Multiples
What is the greatest common factor of 
The greatest common factor is the largest factor that both numbers share. Each number has many factors. The factors for 72 are as follows:
Starting from the largest factor, 72, we can see that it is also a factor of 144
Therefore, 72 is the greatest common factor.
Example Question #2 : Factors / Multiples
The greatest common divisor of 
The greatest common divisor of 
The greatest common divisor of 
If 
The greatest common divisor of 
The greatest common divisor of 
The greatest common divisor of 
Thus:
We substitute these equalities into the given expression and simplify.
Since 
Therefore:
or
Certified Tutor
Certified Tutor
All PSAT Math Resources
