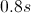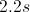MCAT Physical : Newtonian Mechanics and Motion
Study concepts, example questions & explanations for MCAT Physical
All MCAT Physical Resources
Example Questions
Example Question #1 : Newtonian Mechanics And Motion
A 200-gram object moves in the +x direction at 4m/s and collides with an identical object moving in the –y direction at 3m/s. If the two objects stick together after the collision, what is the magnitude of their resulting velocity?
2.5m/s
7m/s
6.5m/s
3.5m/s
5m/s
2.5m/s
Use conservation of momentum in each direction: 
The initial momentum in the x direction is provided only by the first object.
This also equals the final x-momentum, when the two objects move together at the same velocity.
The initial momentum in the y direction is provided only by the second object.
Again, this equals the final y-momentum.
Combine the two velocities using the Pythagorean theorem.
Example Question #2 : Newtonian Mechanics And Motion
Two children are playing with sleds on a snow-covered hill. Sam weighs 50kg, and his sled weighs 10kg. Sally weighs 40kg, and her sled weighs 12kg. When they arrive, they climb up the hill using boots. Halfway up the 50-meter hill, Sally slips and rolls back down to the bottom. Sam continues climbing, and eventually Sally joins him at the top.
They then decide to sled down the hill, but disagree about who will go first.
Scenario 1:
Sam goes down the hill first, claiming that he will reach a higher velocity. If Sally had gone first, Sam says they could collide.
Scenario 2:
Sally goes down the hill first, claiming that she will experience lower friction and thus reach a higher velocity. If Sam had gone first, Sally says they could collide.
Scenario 3:
Unable to agree, Sam and Sally tether themselves with a rope and go down together.
If the hill is frictionless, what momentum does Sam experience when he reaches the bottom of the 50m hill on his sled in Scenario 1?
1340kg*m/s
0kg*m/s
1581kg*m/s
60kg*m/s
1898kg*m/s
1898kg*m/s
The momentum is equal to Sam's total mass times his velocity. His velocity can be found from his potential energy, using conservation of energy. Keep in mind, his total mass includes the mass of his sled.
PE = mgh = (50kg + 10kg) * 10m/s2 * 50m
PE = 30,000J
KE = 30,000J = 1/2mv2 = 1/2(60kg)(v2)
v2 = 1000 m2/s2 = 31.2m/s
p = mv = 60 kg * 31.2 m/s = 1898 kg*m/s
If you had used 30J in place of 30kJ in the KE calculation, you would have chosen 60kg*m/s
Example Question #3 : Newtonian Mechanics And Motion
Two children are playing with sleds on a snow-covered hill. Sam weighs 50kg, and his sled weighs 10kg. Sally weighs 40kg, and her sled weighs 12kg. When they arrive, they climb up the hill using boots. Halfway up the 50-meter hill, Sally slips and rolls back down to the bottom. Sam continues climbing, and eventually Sally joins him at the top.
They then decide to sled down the hill, but disagree about who will go first.
Scenario 1:
Sam goes down the hill first, claiming that he will reach a higher velocity. If Sally had gone first, Sam says they could collide.
Scenario 2:
Sally goes down the hill first, claiming that she will experience lower friction and thus reach a higher velocity. If Sam had gone first, Sally says they could collide.
Scenario 3:
Unable to agree, Sam and Sally tether themselves with a rope and go down together.
A third boy, John, comes to play on a neighboring hill. Sally goes to play with him, and they find that after they sled down the first hill, Sally was traveling 12m/s. John was only moving at 8m/s. John weighs 80kg with his sled. Which of the following is true?
Sally has lower momentum, John has lower kinetic energy
Sally has lower momentum, John has greater kinetic energy
Sally has greater momentum, John has lower kinetic energy
Sally has greater momentum, while they have roughly equal kinetic energies
Sall has greater momentum, John has greater kinetic energy
Sally has lower momentum, John has lower kinetic energy
Kinetic energy emphasizes velocity, by squaring the velocity term.
KE = 1/2mv2
Sally's KE = 1/2(52 kg)(12 m/s)2 = 3,744J
John's KE = 1/2(80 kg)(8m/s)2 = 2,560J
p = mv
Sally's Momentum = 52kg * 12m/s = 624 kg*m/s
John's Momentum = 80kg * 8m/s = 640 kg*m/s
Example Question #4 : Newtonian Mechanics And Motion
Two astronauts, Ann and Bob, conduct a collision experiment in a weightless, frictionless environment. Initially Ann moves to the right with a momentum of 
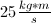
Apply conservation of momentum before and after the collision.
Taking left to be the negative direction, and noting that Bob's initial momentum is 0 since he is at rest, we can use the provided information to see that 
Solving for 
Example Question #5 : Newtonian Mechanics And Motion
In a totally inelastic collision between two objects, all of the following are true, except that __________.
the two objects stick together
kinetic energy is conserved
momentum is conserved
energy is conserved
kinetic energy is conserved
The difference between an elastic and inelastic collision is that in an elastic collision BOTH momentum and kinetic energy are conserved. Momentum is conserved in any collision, but when a collision is inelastic, kinetic energy is dissipated when the two individual objects combine. This energy can be in the form of heat, explosion, sound, etc. In a totally inelastic collision, the two objects colliding stick together and travel with the same velocity in one direction.
Overall energy is conserved, as the energy is merely transferred from a kinetic form to a non-kinetic form.
Example Question #6 : Newtonian Mechanics And Motion
Which object has the most momentum?
I. A 
II. A 
III. An 
III
I
II and III are equal
II
I, II, and III are equal
III
We can find the momentum for each object using the momentum formula, 
Example Question #7 : Newtonian Mechanics And Motion
Two cars collide in a head-on collision. Afterward, they move together with a net momentum eastward. Which of the following collisions could produce this result?
A 
A 
A 
A 
A 
A
The momentum formula is 
Answer possibilities:
Example Question #1 : Momentum
A 
What is the velocity of the bowling ball after the collision?
We can set this up as a conservation of momentum problem. The sum of the initial momentum for each object must equal the sum of the final momentum of each object.
We have two objects and four different velocities. The system must conserve momentum between the time before the collision and after the collision. We are given the mass of each object and their initial velocities.
We are given the magnitude of the billiard ball's final velocity, and told that it changes directions, thus becoming negative. Remember that both velocity and momentum are vector quantities.
Finally, we can solve of the final velocity of the bowling ball. We can predict that the bowling ball's velocity will be positive since the net initial velocity is positive, and the final billiard ball velocity is negative. The bowling ball must compensate for the negative momentum of the billiard ball.
Example Question #9 : Newtonian Mechanics And Motion
A 
The best way to solve this problem is to use the impulse, or change in momentum. Impulse can be calculate from either of two equations:
We can set these equations equal, and use the given values for mass and velocity to calculate the impulse.
We are given the applied force, allowing us to solve for the time.
Example Question #1 : Translational Motion
In which of the following collisions is momentum not conserved?
All of the collisions describe situations in which momentum is conserved
A football player tackles another football player travelling in the opposite direction
Two cars travelling in perpendicular directions crash and slide along the asphalt in one piece
A star explodes and releases material in several different directions
A bullet is shot at a moving target and becomes embedded upon impact
All of the collisions describe situations in which momentum is conserved
Momentum is conserved in both elastic and inelastic collisions. During an elastic collision, kinetic energy is also conserved and the two objects remain separate after impact. During an inelastic collision, kinetic energy is lost to the surroundings and the objects traditionally stick together upon impact. The energy lost in an inelastic collision is generally transformed into heat or sound.
The only time momentum will not be conserved is when an outside force is introduced or the masses of objects do not remain constant.
Certified Tutor
Certified Tutor
All MCAT Physical Resources
