All Linear Algebra Resources
Example Questions
Example Question #71 : Linear Algebra
True or False, the Constrained Extremum Theorem only applies to skew-symmetric matrices.
Possible Answers:
False
True
Correct answer:
False
Explanation:
It only applies to symmetric matrices, not skew-symmetric ones. The Constrained Extremum Theorem concerns the maximum and minimum values of the quadratic form 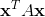

Example Question #1 : Eigenvalues As Optimization
The maximum value of a quadratic form 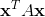



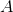
Possible Answers:
The eigenvalue with the greatest multiplicity
None of the other answers
The largest eigenvalue
The second largest eigenvalue
The smallest eigenvalue
Correct answer:
The largest eigenvalue
Explanation:
This is the statement of the Constrained Extremum Theorem. Likewise, the minimum value of the quadratic form corresponds to the smallest eigenvalue of 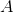
Ping
Certified Tutor
Certified Tutor
The University of Texas at Dallas, Bachelor of Science, Computer Science. University of North Texas, Master of Arts, Education.
Manuel
Certified Tutor
Certified Tutor
University of Puerto Rico, Bachelor of Engineering, Electrical Engineering. Marquette University, Master of Electrical Engine...
All Linear Algebra Resources
Popular Subjects
Algebra Tutors in New York City, GMAT Tutors in Houston, Statistics Tutors in Dallas Fort Worth, Math Tutors in Atlanta, Calculus Tutors in Denver, English Tutors in Boston, GMAT Tutors in San Diego, LSAT Tutors in Boston, Math Tutors in Boston, GRE Tutors in Washington DC
Popular Courses & Classes
GRE Courses & Classes in Seattle, GMAT Courses & Classes in Houston, Spanish Courses & Classes in Los Angeles, Spanish Courses & Classes in Houston, SSAT Courses & Classes in New York City, Spanish Courses & Classes in Boston, ISEE Courses & Classes in Denver, SSAT Courses & Classes in Washington DC, SAT Courses & Classes in New York City, ISEE Courses & Classes in Atlanta
Popular Test Prep
SSAT Test Prep in Philadelphia, MCAT Test Prep in Chicago, SAT Test Prep in Seattle, GMAT Test Prep in Seattle, GMAT Test Prep in Houston, SAT Test Prep in Phoenix, GRE Test Prep in Houston, GMAT Test Prep in Chicago, GMAT Test Prep in San Francisco-Bay Area, SAT Test Prep in Miami




