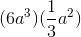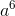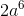We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

ISEE Upper Level Math : Numbers and Operations

Study concepts, example questions & explanations for ISEE Upper Level Math

Create An Account

All ISEE Upper Level Math Resources

6 Diagnostic Tests 244 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

← Previous 1 2 3 4 5 6 7 8 9 10 11 12 Next →

Example Question #5 : How To Multiply Exponents

Simplify:

$(6a^3)(\frac{1}{3}a^2)$

Possible Answers:

a^6

2a^6

2a^4

a^5

2a^5

Correct answer:

2a^5

Explanation:

Based on the product rule for exponents in order to multiply two exponential terms with the same base, add their exponents:

$(x)^a(x)^b=x^{a+b}$

So we can write:

$(6a^3)\left(\frac{1}{3}a^2\right)=\left(\frac{6}{3}\right)\times a^{3+2}=2a^5$

Report an Error

Example Question #6 : How To Multiply Exponents

Evaluate:

$\frac{4x^2(x^3)^{3}}{8x^7}$

Possible Answers:

$\frac{1}{2}x^3$

x^4

x^3

$\frac{1}{2}x^4$

$\frac{1}{2}x^5$

Correct answer:

$\frac{1}{2}x^4$

Explanation:

Based on the power rule for exponents we can write:

$(a^m)^{n}=a^{m\times n}$

That means; to raise a power to a power we need to multiply the exponents. In addition, based on the product rule for exponents, in order to multiply two exponential terms with the same base we need to add their exponents:

$(x)^a(x)^b=x^{a+b}$

So we can write:

$\frac{4x^2(x^3)^{3}}{8x^7}=\frac{4x^2(x^{3\times 3})}{8x^7}=\frac{4x^2\times x^9}{8x^7}=\left(\frac{4}{8}\right)\frac{x^{2+9}}{x^7}=\frac{1}{2}\cdot \frac{x^{11}}{x^7}$

in order to divide two exponents with the same base, we can keep the base and subtract the powers. So we get:

$\frac{4x^2(x^3)^{3}}{8x^7}=\frac{1}{2}\cdot \frac{x^{11}}{x^7}=\frac{1}{2}x^{11-7}=\frac{1}{2}x^4$

Report an Error

Example Question #7 : How To Multiply Exponents

Evaluate:

$-2x^2(x^{-3})^{3}$

Possible Answers:

$2x^{7}$

$2x^{-7}$

$-2x^{7}$

$-x^{-7}$

$-2x^{-7}$

Correct answer:

$-2x^{-7}$

Explanation:

Based on the negative exponent rule we have:

$a^{-n}=\frac{1}{a^n}$

which says negative exponents in the numerator get moved to the denominator and become positive exponents. And negative exponents in the denominator get moved to the numerator and become positive exponents. So we can write:

$-2x^2(x^{-3})^{3}=-2x^2\left(\frac{1}{x^3}\right)^{3}$

In addition, based on the power rule for exponents we can write:

$(a^m)^{n}=a^{m\times n}$

That means; to raise a power to a power we need to multiply the exponents. We also know that when a fraction is raised to a power, the numerator and the denominator are both raised to that power. So we can write:

$-2x^2(x^{-3})^{3}=-2x^2\left(\frac{1}{x^3}\right)^{3}=-2x^2\times \frac{1^3}{x^{3\times 3}}$

$=-2x^2\times \frac{1}{x^9}$

$=\frac{-2x^2}{x^9}$

$=-2x^{2-9}$

$=-2x^{-7}$

Report an Error

Example Question #21 : Exponential Operations

If x=2 , find the value of:

$\frac{3x^{\sqrt{2}}(x^{\sqrt{3}})^\sqrt{3}}{x^{\sqrt{2}}}$

Possible Answers:

Correct answer:

Explanation:

Based on the power rule for exponents we can write:

$(a^m)^{n}=a^{m\times n}$

That means; to raise a power to a power we need to multiply the exponents. So we can write:

$\frac{3x^{\sqrt{2}}(x^{\sqrt{3}})^\sqrt{3}}{x^{\sqrt{2}}}=3\times \left(\frac{x^{\sqrt{2}}}{x^{\sqrt{2}}}\right) \times (x^{\sqrt{3}})^{\sqrt{3}}=3x^{\sqrt{3}\times \sqrt{3}}=3x^3$

Substitute x=2 and we get:

$3x^3=3\times 2^3=3\times 8=24$

Report an Error

Example Question #3 : How To Multiply Exponents

Simplify:

$(x^{2\sqrt{2}})^{3\sqrt{2}}$

Possible Answers:

$x^{6}$

$x^{12}$

$x^{6\sqrt{}2}$

$x^{2}$

$x^{8}$

Correct answer:

$x^{12}$

Explanation:

Based on the power rule, we know that in order to raise a power to a power we need to multiply the exponents, i.e.

$(x^{m})^{n}=x^{m\times n}$ .

$(x^{2\sqrt{2}})^{3\sqrt{2}}=x^{2\sqrt{2}\times 3\sqrt{2}}=x^{2\times 3\times 2}=x^{12}$

Report an Error

Example Question #23 : Exponents

Simplify:

$(\frac{12x^{3}}{3x^{-1}})^{4}$

Possible Answers:

$256x^{12}$

$16x^{8}$

$256x^{8}$

$256x^{16}$

$16x^{16}$

Correct answer:

$256x^{16}$

Explanation:

The Negative Exponent Rule says $a^{-n}=\frac{1}{a^n}$ .

$(\frac{12x^{3}}{3x^{-1}})^{4}=(\frac{12}{3}\times x^{3+1})^{4}=(4x^4)^{4}$

The power rule says that, in order to raise a power to a power, we need to multiply the exponents, i.e. $(x^{m})^{n}=x^{m\times n}$ .

$(\frac{12x^{3}}{3x^{-1}})^{4}=(4x^4)^{4}=4^4\times x^{4\times 4}=256x^{16}$

Report an Error

Example Question #71 : Numbers And Operations

What is the value of this equation?

$(x^{2})^{3}-(2^{3})^{2}$

Possible Answers:

$x^{5}-64$

$x^{6}-32$

$x^{6}+64$

$x^{6}-64$

Correct answer:

$x^{6}-64$

Explanation:

When an exponent is raised to another exponent, the exponents should be multiplied toghether. This will result in:

$(x^{2})^{3}-(2^{3})^{2}$

$x^{6}-2^{6}$

$x^{6}-64$

Report an Error

Example Question #72 : Numbers And Operations

Which expression is equal to $(x^{2})^{3}$ ?

Possible Answers:

$x^{\frac{2}{3}}$

$x^{6}$

$x^{\frac{3}{2}}$

$x^{5}$

Correct answer:

$x^{6}$

Explanation:

When exponent of a value is raised to another exponent, the values of the exponents are multiplied by each other.

$(x^{2})^{3}$

2 is multiplied by 3, and so the exponent of x is 6.

$x^{6}$

Report an Error

Example Question #73 : Numbers And Operations

What is the value of $(2^{6})^{\frac{1}{2}}$ ?

Possible Answers:

Correct answer:

Explanation:

When one exponent is raised to another exponent, the values of the exponents should be multiplied together. Thus,

$(2^{6})^{\frac{1}{2}}$ can be simplified to $2^{3}$ , given that $6\cdot \frac{1}{2}=3$ .

$2^{3}=2\cdot2\cdot2=8$

Report an Error

Example Question #74 : Numbers And Operations

What is the expression below equal to?

$3x^{4}*5x^{9}$

Possible Answers:

$8x^{9}$

$15x^{36}$

$15x^{13}$

$8x^{36}$

Correct answer:

$15x^{13}$

Explanation:

When exponents are multiplied by each other, the powers should be added together. Meanwhile, numbers not raised to an exponent are simply multiplied by each other.

Therefore, the answer is $15x^{13}$ , because 3*5=15 , and 9+4=13 .

Report an Error

← Previous 1 2 3 4 5 6 7 8 9 10 11 12 Next →

View Tutors

Jaroslaw
Certified Tutor

Heriot Watt University, Master of Science, Physics. Heriot Watt University, Doctor of Science, Theoretical and Mathematical P...

View Tutors

Matt
Certified Tutor

View Tutors

Gregory
Certified Tutor

Pennsylvania State University-Main Campus, Bachelor of Science, Chemical Engineering. Carnegie Mellon University, Doctor of P...

All ISEE Upper Level Math Resources

6 Diagnostic Tests 244 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

English Tutors in Houston, Reading Tutors in Chicago, Statistics Tutors in New York City, SSAT Tutors in Boston, MCAT Tutors in Miami, Algebra Tutors in Chicago, ISEE Tutors in Houston, ISEE Tutors in Los Angeles, Statistics Tutors in Boston, SSAT Tutors in New York City

Popular Courses & Classes

SAT Courses & Classes in New York City, ISEE Courses & Classes in Miami, LSAT Courses & Classes in New York City, ACT Courses & Classes in San Diego, SSAT Courses & Classes in Miami, ACT Courses & Classes in Seattle, SAT Courses & Classes in San Diego, SSAT Courses & Classes in Atlanta, GMAT Courses & Classes in Los Angeles, MCAT Courses & Classes in Houston

Popular Test Prep

MCAT Test Prep in New York City, SAT Test Prep in Philadelphia, ISEE Test Prep in Washington DC, ACT Test Prep in San Diego, GRE Test Prep in Dallas Fort Worth, GRE Test Prep in San Francisco-Bay Area, SSAT Test Prep in Atlanta, ACT Test Prep in Seattle, SAT Test Prep in Boston, SSAT Test Prep in New York City

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ISEE Upper Level Math : Numbers and Operations

Study concepts, example questions & explanations for ISEE Upper Level Math

All ISEE Upper Level Math Resources

Example Questions

Example Question #5 : How To Multiply Exponents

Example Question #6 : How To Multiply Exponents

Example Question #7 : How To Multiply Exponents

Example Question #21 : Exponential Operations

Example Question #3 : How To Multiply Exponents

Example Question #23 : Exponents

Example Question #71 : Numbers And Operations

Example Question #72 : Numbers And Operations

Example Question #73 : Numbers And Operations

Example Question #74 : Numbers And Operations

All ISEE Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: