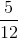All ISEE Middle Level Quantitative Resources
Example Questions
Example Question #31 : Adding Fractions In Word Problems
On Friday it snowed 

To solve this problem, we are putting the amount of snowfall from the afternoon and the evening together, so we add the fractions.

Example Question #32 : Adding Fractions In Word Problems
On Thursday it snowed 

To solve this problem, we are putting the amount of snowfall from the afternoon and the evening together, so we add the fractions.

Example Question #33 : Adding Fractions In Word Problems
On Wednesday it snowed 

To solve this problem, we are putting the amount of snowfall from the afternoon and the evening together, so we add the fractions.

Example Question #34 : Adding Fractions In Word Problems
On Tuesday it snowed 

To solve this problem, we are putting the amount of snowfall from the afternoon and the evening together, so we add the fractions.

Example Question #222 : How To Add Fractions
On Monday it snowed 

To solve this problem, we are putting the amount of snowfall from the afternoon and the evening together, so we add the fractions.

Example Question #35 : Adding Fractions In Word Problems
On Saturday it snowed 

To solve this problem, we are putting the amount of snowfall from the afternoon and the evening together, so we add the fractions.

Example Question #2001 : Numbers And Operations
In order to solve this problem, we first have to find common denominators.
Now that we have common denominators, we can add the fractions. Remember, when we add and subtract fractions, we only add or subtract the numerator.
Example Question #2002 : Numbers And Operations
In order to solve this problem, we first have to find common denominators.
Now that we have common denominators, we can add the fractions. Remember, when we add and subtract fractions, we only add or subtract the numerator.
Example Question #2003 : Numbers And Operations
In order to solve this problem, we first have to find common denominators.
Now that we have common denominators, we can add the fractions. Remember, when we add and subtract fractions, we only add or subtract the numerator.
Example Question #2004 : Numbers And Operations
In order to solve this problem, we first have to find common denominators.
Now that we have common denominators, we can add the fractions. Remember, when we add and subtract fractions, we only add or subtract the numerator.
All ISEE Middle Level Quantitative Resources