ISEE Middle Level Math : Geometry
Study concepts, example questions & explanations for ISEE Middle Level Math
All ISEE Middle Level Math Resources
Example Questions
Example Question #111 : Plane Geometry
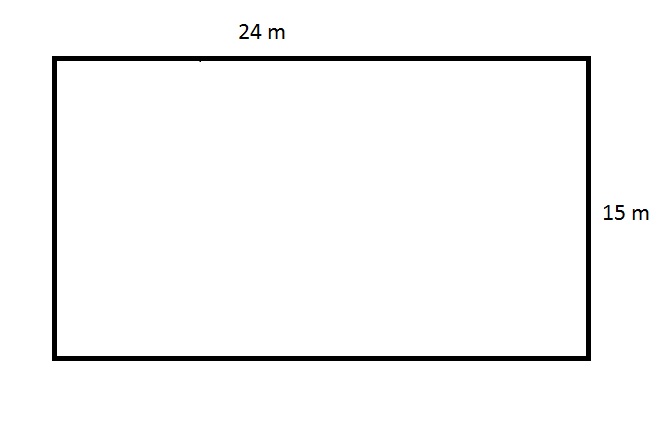
The above figure depicts the rectangular swimming pool at an apartment. The apartment manager needs to purchase a tarp that will cover this pool completely, but the store will only sell the material in multiples of one hundred square meters. How many square meters will the manager need to buy?
Insufficient information is given to answer the question.
The tarp needed to cover this pool must be, at minimum, the product of its length and width, or
The manager will need to buy a number of square yards of tarp equal to the next highest multiple of one hundred, which is 400 square meters.
Example Question #152 : Geometry
The four angles of a square are labeled A, B, C, and D. What is the sum of 
More information is needed to solve
In a square, each angle is 90 degrees.
We can plug in 90 for each variable and find the sum.
Example Question #24 : How To Find The Area Of A Rectangle
The above depicts a rectangular swimming pool for an apartment. The pool is six feet deep everywhere.
An apartment manager wants to paint the four sides and the bottom of the swimming pool. How many square feet will he need to paint?
The correct answer is not given among the other responses.
The bottom of the swimming pool has area
There are two sides whose area is
and two sides whose area is
Add the areas:
Example Question #25 : How To Find The Area Of A Rectangle
If the angles of a quadrilateral are equal to 
Given that there are 360 degrees in a quadrilateral,
Example Question #32 : How To Find The Area Of A Rectangle
What is the value of 
Given that there are 360 degrees in a quadrilateral,
Example Question #181 : Geometry
If the length of a rectangle is 7.5 feet and the width is 2 feet, what is the value of 
The area of a rectangle is calculated by multiplying the length by the width. Here, the length is 7.5 and the width is 2, so the area will be 15.
Given that the area is also equal to 
Example Question #161 : Geometry
Which of the following is equal to the area of a rectangle with length 
Multiply each dimension by 
Multiply these dimensions to get the area of the rectangle in square centimeters:
Example Question #182 : Geometry
Find the area of a rectangle whose length is 6 and width is 5.
To solve, simply use the formula for the area of a rectangle.
In this particular case the length and width are given,
Thus:
Example Question #30 : How To Find The Area Of A Rectangle
The area of a four-sided room that has dimensions of 
False
True
False
The area of a rectangle is the length times the width. So to calculate it, you must multiple the two different lengths together. Adding the four wall lengths would get you the perimeter instead.
Example Question #31 : How To Find The Area Of A Rectangle
Use the following to answer the question.
Find the area of the rectangle if it's width is half of it's length.
To find the area of a rectangle, we use the following formula:
where l is the length and w is the width of the rectangle.
Now, given the rectangle,
we can see the length is 12 feet. We also know the width is half of the length. Therefore, the width is 6 feet. Knowing this, we can substitute into the formula. We get
Certified Tutor
Certified Tutor
All ISEE Middle Level Math Resources
