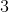In order to find the volume of the figure, we will first need to find the volumes of the rectangular prism and the half cylinder.

From the figure, you should notice that the length of the prism is also the diameter of the half cylinder. Thus, half the length of the prism will also be the radius of the half cylinder. Also, notice that the width of the prism will be the height of the cylinder.

For the given cylinder then, find the radius.

Then, recall how to find the volume of a cylinder:

, where  is the radius and  is the height.

Divide the volume by  to find the volume of the half cylinder.

Next, recall how to find the volume of a rectangular prism.

Plug in the given values to find the volume of the rectangular prism.

To find the volume of the figure, add the volume of the half cylinder and the volume of the rectangular prism together.

Remember to round to  places after the decimal.

Let  be the radius of the sphere. Then the radius of the base of the cylinder is also , and the height of the cylinder is .

The volume of the cylinder is

,

which, after substituting, is

The volume of the sphere is 

Therefore, the ratio of the former to the latter is

and 

That is, the volume of the cylinder is  times that of the sphere, so the volume of the cylinder is

.

Since we are putting the snow on a circular base, we are dealing with a cylinder. Notice that the radius of the cylinder and the volume of the cylinder are already given to you.

Recall how to find the volume of a cylinder:

Rearrange the equation to solve for the height:

Plug in the given volume and radius:

The height is between  and  meter.

The volume of a prism is length times width times height.

We are given the length is 7m and the width is 5m.

So, we plug these into our formula:

 .

We then solve for height:

 .

The height is therefore 6m. 

The goal is to find the height of the rectangular prism with the given information of its width and length. The volume of a rectangular prism is , where  is width and  is height. 

Because we're given the final volume and two of the three variables, we can substitute in the information we know and solve for the missing variable. 

Therefore, the height of the prism is .

If we let  be the width of our prism, then since the length is twice the width, our length would be .  Since the height is twice the length, our height would be .

Since the volume of a rectangular prism is simply the product of width, length, and height, we get

We then simply solve for the width.

Therefore, our width is 3.  Since our length is twice the width, the length is 6.

The formula for the volume of a right, rectangular prism is , so substitute the known values and solve for lenght.  

.  So the length is 8 inches.

Use the formula for finding the surface area of a right, rectangular prism,

and substitute the known values, and then solve for w.

So, 

So, the width is 7 cm.

Since the length of the box is twice the width and the height is three times the width,  and . 

The surface area of the box is

To find the width:

To find the surface area of a prism, the problem can be approached in one of two ways.

1. Through an equation that uses lateral area
2. Through finding the area of each side and taking the sum of all the faces

Using the second method, it's helpful to realize rectangular prisms contain  faces. With that, it's helpful to understand that there are  pairs of sides. That is, there are two faces with the same dimensions. Therefore, we really only have three sides for which we need to calculate areas: 

Faces 1 & 2:

Faces 3 & 4:

Faces 5 & 6:

Now, we can add up the areas of all six sides:

The surface area is .