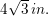We have the length, the width, and the volume for this cube. We just need to figure out the height.

Recall how to find the volume of a cube:

Normally, these three values would be the same. However, in this case, since the pool is not being filled up the entire way, the height of the cube will be different from its length and width.

The pool will be  meters deep.

A geometric cube has edges, all of equal length and the formula to solve for volume is:

And since all edges are of equal length, we can use this formula to solve for length of sides:

The formula for volume of a perfect sphere is represented in this formula, with representing length of sides:

Since we know the volume, we can use the formula for volume to determine length of edges:

The diagonal of a cube is simply given by:

Where  is the side length of the cube.

So since our 

Write the volume of a cube and substitute the given volume to find a side length.

Write the diagonal formula for a cube and substitute the side length.

There is a formula for the length of a cube's diagonal given the side length.  However, we might not remember that formula as it is less common.  However, we can also find the length using the Pythagorean Theorem.

But first, we need to find the side length.  We know the volume is 64.  Our formula for volume is

Substituting gives

Taking the cube root gives us a side length of 4.  Now let's look at our cube.

We need to begin by finding the length of the diagonal of the bottom face of our cube (the green segment).  This can be done either by using the Pythagorean Theorem or by realizing that the right triangle is in fact a 45-45-90 triangle.  Either way, we realize that our diagonal (the hypotenuse) is .

We now seek to find the diagonal of the cube (the blue segment).  We do this by looking at the right triangle formed by it, the left vertical edge, and the face diagonal we just found.  This time our only recourse is to do the Pythagorean Theorem.

In general, the formula for the diagonal of a cube with side length  is

The length of our diagonal is 

Write the equation for the volume of a cube.  Substitute the volume to find the side length, .

Write the equation for finding diagonals given an edge length for a cube.

Substitute the side length to find the diagonal length.

The answer is .

Write the equation for finding the volume of a cube, and substitute in the volume.

Write the diagonal equation for cubes and substitute in the given length.

Write the surface area formula for cubes and substitute the given area.

Write the diagonal formula for cubes and substitute the side length.

When calculating the diagonal of the cube, point A to point B. 

We must first find the diagonal of the base of the cube. 

The base of the cube is a square where all sides are 8. 

The diagonal of this square is found either by the pythagorean theorem or by what we know about 45-45-90 triangles to get the diagonal of the base below: 

The diagonal of the base would be from point A to point C in the drawing. 

We can see that the diagonal of the base and side BC of the cube form the two legs of a right triangle that will allow us to find the 3D diagonal of the whole cube. 

Use the pythagorean theorem with BC and the Diagonal of the Base. 

Take the square root of both sides. 

After simplifying everything we get the final answer for the Diagonal of the Cube (AB).